Find the distance travelled by by the uniformly acclerated object moving in one dimension in nth second.
To find the distance traveled by a uniformly accelerated object in one dimension during the nth second, we can use the following steps:
1. Understanding the Motion:
- We have an object moving with uniform acceleration a.
- Let the initial velocity of the object be u.
- We need to find the distance traveled during the nth second.
2. Formula for Distance in nth Second:
- The distance traveled in the nth second can be calculated using the formula:
Sn=u+12a(2n−1)
- Here, Sn is the distance traveled in the nth second, u is the initial velocity, a is the acceleration, and n is the specific second we are interested in.
3. Deriving the Formula:
- The total distance traveled in n seconds can be expressed as:
Sn=S(n)−S(n−1)
- Where S(n) is the total distance covered in n seconds and S(n−1) is the total distance covered in n−1 seconds.
- The total distance S(n) can be calculated using the equation of motion:
S(n)=un+12an2
- Similarly, for S(n−1):
S(n−1)=u(n−1)+12a(n−1)2
- Substituting these into the distance formula gives:
Sn=(un+12an2)−(u(n−1)+12a(n−1)2)
- Simplifying this expression leads to the formula for distance in the nth second:
Sn=u+12a(2n−1)
4. Final Expression:
- Thus, the distance traveled by the uniformly accelerated object in the nth second is given by:
Sn=u+12a(2n−1)
- We have an object moving with uniform acceleration a.
- Let the initial velocity of the object be u.
- We need to find the distance traveled during the nth second.
- The distance traveled in the nth second can be calculated using the formula:
Sn=u+12a(2n−1)
- Here, Sn is the distance traveled in the nth second, u is the initial velocity, a is the acceleration, and n is the specific second we are interested in.
- The total distance traveled in n seconds can be expressed as:
Sn=S(n)−S(n−1)
- Where S(n) is the total distance covered in n seconds and S(n−1) is the total distance covered in n−1 seconds.
- The total distance S(n) can be calculated using the equation of motion:
S(n)=un+12an2
- Similarly, for S(n−1):
S(n−1)=u(n−1)+12a(n−1)2
- Substituting these into the distance formula gives:
Sn=(un+12an2)−(u(n−1)+12a(n−1)2)
- Simplifying this expression leads to the formula for distance in the nth second:
Sn=u+12a(2n−1)
- Thus, the distance traveled by the uniformly accelerated object in the nth second is given by:
Sn=u+12a(2n−1)
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 3 Short answer
37 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 4 Short answer
30 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 Short answer
25 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
Derive an equation for the distance covered by a uniformly accelerated body in nth second of its motion. A body travels half its total path in the last second of its fall from rest, calculate the time of its fall.
View SolutionObtain a relation for the distance travelled by an object moving with a unifrom acceleration in the interval between 4th and 5th seconds.
View Solution
Knowledge Check
The distance travelled by the moving body is :
AThe area between the speed time graph and time axis.BThe area between the speed time graph and speed axisCThe area between the distance time graph and time axisDThe area between the distance time graph and distance axisAssertion : In the equation, s=u+at−12a where, s is the distance travelled by uniformly accelerated body in tth second.
Reason : The above equation is dimensionally incorrect.AIf both Assertion and Reason are correct and Reason is the correct explanation of assertion.BIf both Assertion and Reason are correct but Reason in not the correct explanation of Assertion.CIf Assertion is true but Reason is false.DIf Assertion is false but Reason is true.
How is the position-time graph of uniformly accelerated motion id one dimension helpful in studying the motion of the object ?
View SolutionDerive the equations of motion for an object in uniformly accelerated motion in one dimension using calculus.
View SolutionConsider a particle intially moving with a velocity of 5 m s−1 starts decelerating at a constant rate of 2 m s−2.
a. Determine the time at which the particle becomes stationary.
b. Find the distance travelled in the second second.
c. Find the distance travelled in the third second.
View SolutionThe ratio of the distances travelled by a body starting from rest and moving with uniform acceleration in the first, third and fifth seconds of its journey is
View SolutionThe speed of a car increases uniformly from zero to 10ms−1 in 2s and then remains constant (figure)
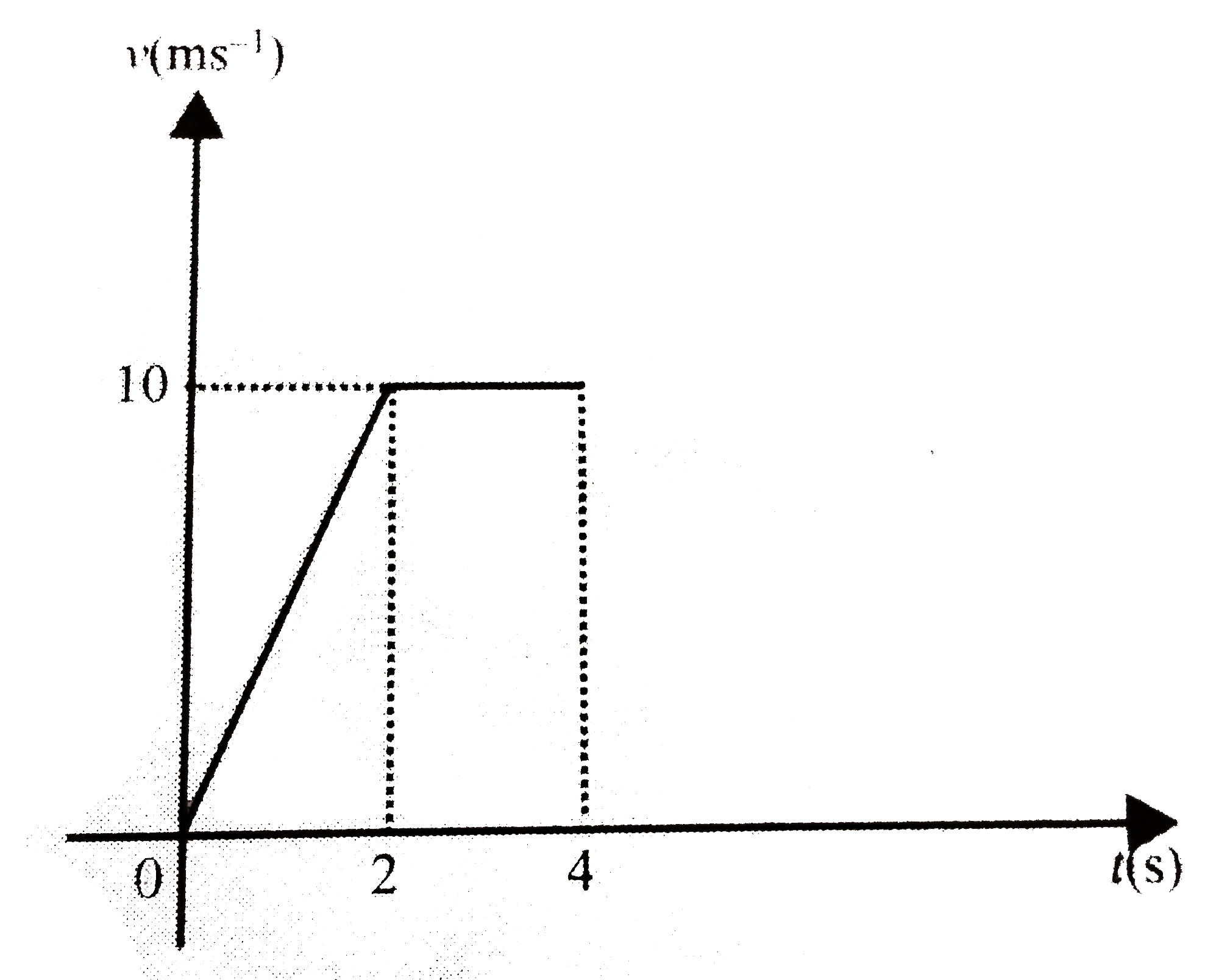
a. Find the distance travelled by the car in the first two seconds.
b. Find the distance travelled by the car in the next two seconds.
c. Find the total distance travelled in 4s.
View SolutionFind the ratio of the distances travelled by a freely falling body in first, second and third second of its fall.
View Solution
