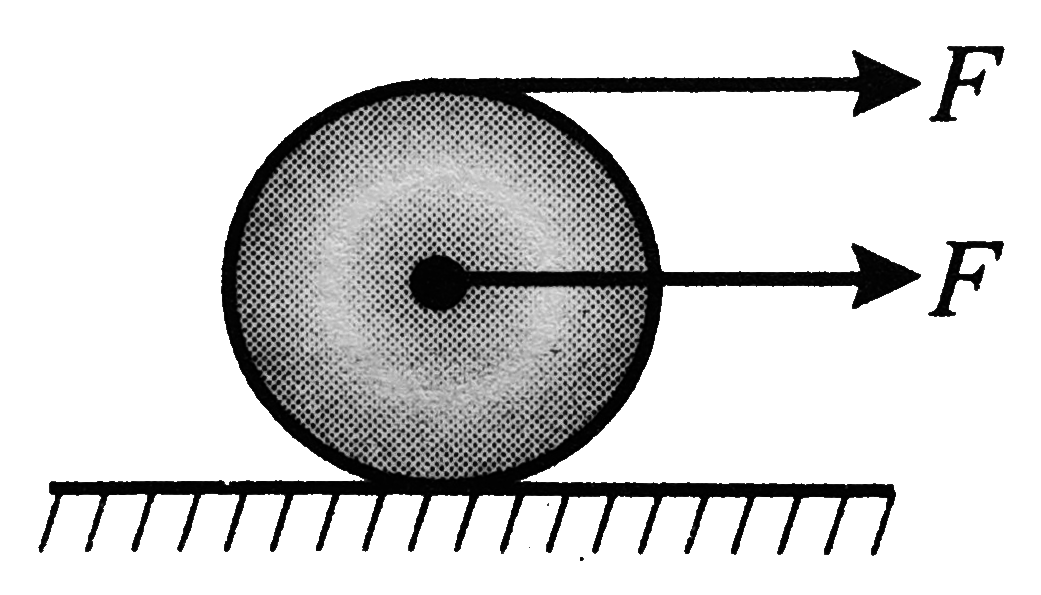
A unifrom disc is acted by two equal forces of magnitude F. One of them, acts tangentially to the disc, while other one is acting at the central point of the disc. The friction between disc surface and ground surface in nF. If r be the radius of the disc, then the value of n would be (in N)
0
1.2
2.0
3.2
The correct Answer is:A
Let f be the frictional force between disc surface and ground surface. Let a be the linear acceleration of the disc. For the motion of the disc, we can write
2F−f=ma …(i)
and (F+f)r=Iα
where I=12mr2andα=ar
∴(F+f)r=12mr2×ar=12mra ..(ii)
Putting value of ma from (i) in (ii), we get
(F+f)r=12(2F−f)r
or F+f=F−f/2orf=0=nF (Given)
So n=0
Similar Questions
Knowledge Check
- Question 1 - Select One
A disc is pulled by a force F acting at a point above the centre of mass of the disc.
The direction of frictional force (fr) acting on disc pushed on a rough surface will be represented by.A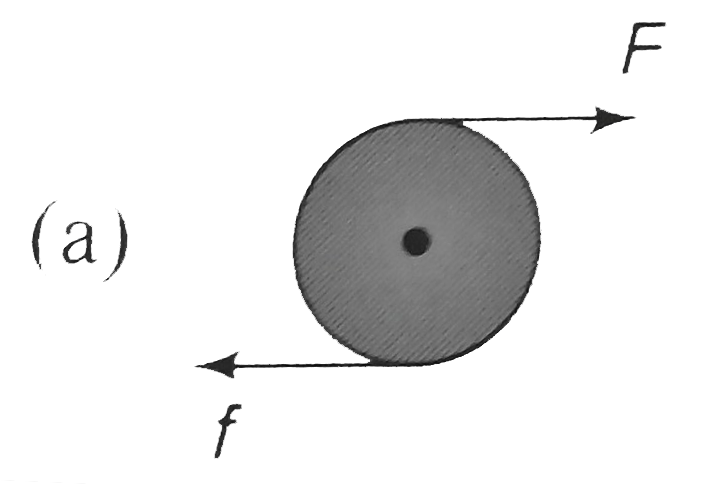
.B
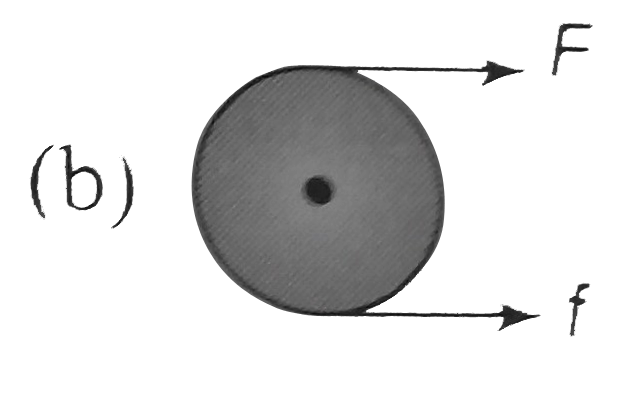.C
.DInformation is insufficient. - Question 2 - Select One
A force F acts tangentially at the highest point of a disc of mass m kept on a rough horizontal plane. If the disc rolls without slipping, the acceleration of centre of the disc is:
A2F3mB10F7mCzeroD4F3m - Question 3 - Select One
A disc of mass m and radius R, is placed on a smooth text service fixed surface . Point A is the geometrical centre of the disc while point B is the centre of mass of the disc . The moment of inertia of the dics about an axis through its centre of mass and perpendicular to the plane of the figure is I .A constant force F is applied to the top of the disc . The acceleration of the centre A of the disc at the instant B is below A (on the same vertical line ) will be :
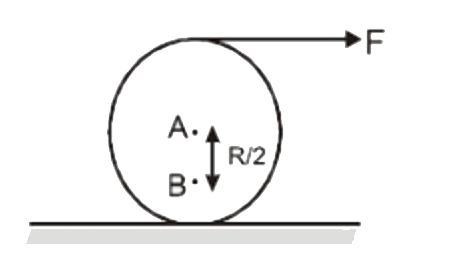AFmBFm+3FR24lCFm+FR24lDFm+3FR2l
A uniform circular disc of radius R is rolling on a horizontal surface. Determine the tangential velocity : the centre of mass
View SolutionA point source of radiation of power P is placed on the axis of completely absorbing disc. The distance between the source and the disc is 2times the radius of the disc. The force that light exerts on the disc is Px40c. Then the value of x
View SolutionA disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.
Force of friction between the disc and the plank isView SolutionStatement I: A disc is allowed to roll purely on an inclined plane as shown in Fig. A force F parallel to the incline and passing through the centre of the disc acts which remains constant during the motion.
It is possible that for certain values of F, the friction on the disc is acting along downward direction and for certain other values of F, the friction on the disc be acting along upward direction and there is no other possibility.
Statement II: The friction (if acting) will be static and not kinetic in nature.View SolutionA disc of mass m has a charge Q distributed on its surface. It is rotating about an XX' with a angular velocity ω. The force acting on the disc is
View Solution