A spool with thread wound on it, of mass m, rests on a rough horizontal surface. Its moment of inertia relative to its own axis is equal to I=γmR2, where γ is a numerical factor, and R is the outside radius of the spool. The radius of the wound thread layer is equal to r. The spool is pulled without sliding by the thread with a constant force F directed at an angle α to the horizontal (figure). Find:
(a) the projection of the acceleration vector of the spool axis on the x-axis,
(b) the work performed by the force F during the first t seconds after the beginning of motion.

(a) Let us choose the positive direction of the rotation angle φ, such that wcx and βz have identical signs (Fig.) Equation of motion, Fx=mwcx and Ncz=Icβz gives:
Fcosα−fr=mwcx :frR−Fr=Icβz=γmR2βz
In the absence of the slipping of the spool wcx=βzR
From the three equations wcx=wc=F[cosα−(r/R)]m(1+γ) , where cosα>rR (1)
(b) As static friction (fr) does not work on the spool, from the equation of the increment of mechanical energy Aext=Δt.
Aext=12mv2c+12γmR2v2cR2=12m(1+γ)v2c
=12m(1+γ)2wcx=12m(1+γ)2wc(12wct2)
=F2(cosα−rR)2t22m(1+γ)
Note that at cosα=r/R, there is no rolling and for cosα<r/R, wcx<0, i.e. the spool will move towards negative x-axis and rotate in anticlockwise sense.

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation34videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
A spool of inner radius R and outer radius 3R has a moment of inertia =MR2 about an axis passing through it's geometric centre, where M is the mass of the spool. A thread wound on the inner surface of the spool is pulled horizontally with a constant force =Mg. Find the acceleration of the point on which is being pulled assuming that the spool rolls purely on the floor.
 .View Solution
.View SolutionA bar of mass m is pulled by means of a thread up an inclined plane forming an angle α with the horizontal (figure). The coefficient of friction is equal to k. Find the angle β which is thread must form with the inclined plane for the tension of the thread to be minimum. What is it equal to?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?
AMR22BMR2C32MR2D2MR2 - Question 2 - Select One
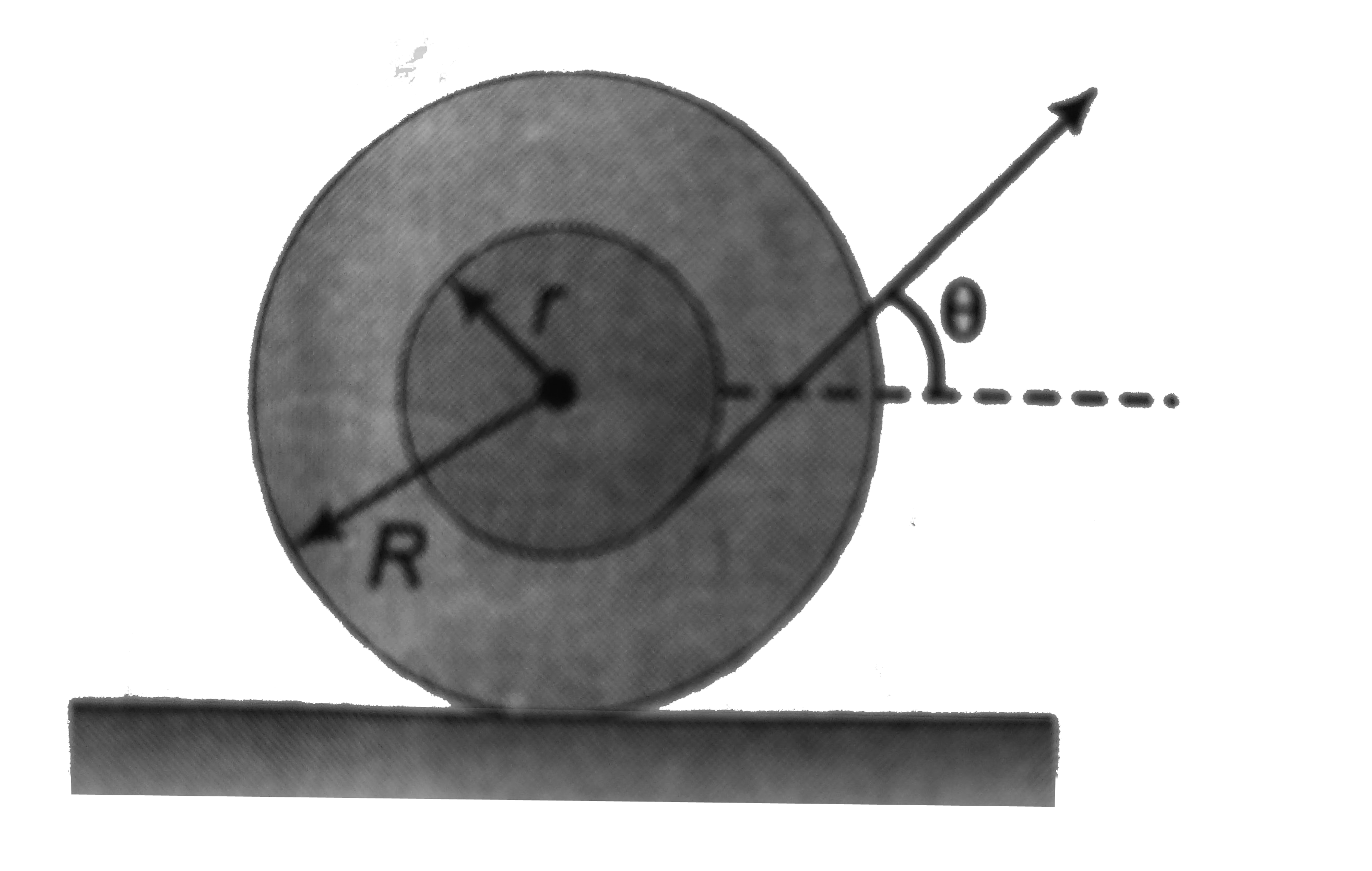
A spool is pulled at an angle θ with the horizontal on a rough horizontal surface as shown in the figure. If the spool remains at rest, the angle θ is equal toAcos−1(Rr)Bsin−1(√1−r2R2)Cπ−cos−1(rR)Dsin−1(rR) - Question 3 - Select One
If the moment of inertia of a rigid body is numerically equal to its mass, radius of gyration is
Aequal to its radiusBequal to diameterCequal to one unitDinfinite
A spool with a thread wound on it is placed on an inclined smooth plane set an angle α=30∘ to the horizontal. The free end of the thread is attached to the wall as shown in figure. The mass of the spool is m=200g, its moment of inertia relative to its own axis I=0.45g⋅m2, the radius of the wound thread layer r=3.0cm. Find the acceleration of the spool axis.
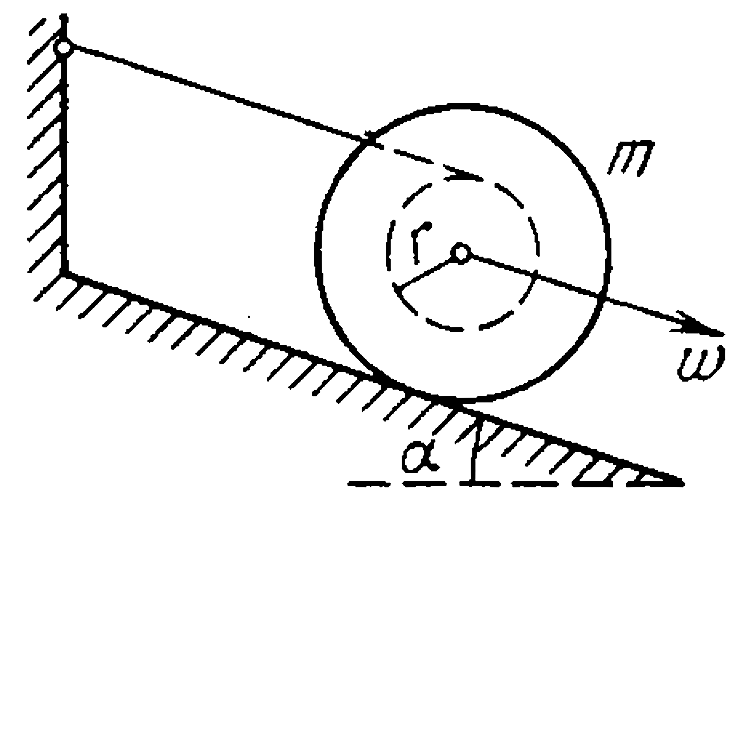 View Solution
View SolutionA spool with a thread wound on it is placed on a smooth inclined plane set at an angle of 30 to the horizontal. The free end of the thread is attached to the wall as shown. The mass of the spool is m r m 200g, its moment of inertia relative to its own axis is I 0.45gm2, the radius of the wound thread layer is r 3 cm. The acceleration of the spool axis is:
 View Solution
View SolutionA uniform cylinder of mass m rests on two rough horizontal planks. A thread is wound on the cylinder. The hanging end of the thread is pulled vertically down with a constant force F
View SolutionThe relation between length L and radius R of the cylinder , if its moment of inertia about its axis is equal to that about the equatorial axis, will be
View SolutionA uniform disc of radius R lies in x-y plane with its centre at origin. Its moment of inertia about the axis x=2R and y=0 is equal to the moment of inertia about the axis y=d and z=0, where d is equal to
View Solution

