Thin threads are tightly wound on the ends of a uniform solid cylinder of mass m. The free ends of the threads are attached to the ceiling of an elevator car. The car starts going up with an acceleration w0. Find the acceleration w′ . Find the acceleration w′ of the cylinder relative to the car and the force F exerted by the cylinder on the ceiling (through the threads).
Let us depict the forces and their points of application corresponding to the cylinder attached with the elevator. Newton's second law for solid in vector form in the frame of elevator, gives:
2→T+m→g+m(−→w0)=m→w (1)
The equation of moment in the C.M. frame relative to the cylinder axis i.e. from Nz=Icβz.
2TR=mR22β=mR22w′R
[as thread does not slip on the cylinder, w′=βR]

or, T=mw′4
As (1) →T↑⏐⏐⏐⏐↓→w
so in vector form
→T=−m→w4 (2)
Solving Eqs. (1) and (2), →'w=23(→g−→w0) and sought force
→F=2→T=13m(→g−→w0)
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics25videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation34videos
PHYSICAL FUNDAMENTALS OF MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena38videos
THERMODYNAMICS AND MOLECULAR PHYSICS
Similar Questions
Knowledge Check
- Question 1 - Select One
A 2kg block hangs without vibrating at the bottom end of a spring with a force constant of 400N/m. The top end of the spring is attached to the ceiling of an elevator car. The car is rising with an upward acceleration of 5m/s2 when the acceleration suddenly ceases at time t=0 and the car moves upward with constant speed (g=10m/s2
The amplitude of the oscillation isA7.5cmB5cmC2.5cmD1cm
A pulley fixed to the ceiling of an elevator car carries a thread whose ends are attached to the loads of masses 3m and m . The car starts gong up with acceleration g/s. Assuming the masses of the pulley and the thread, as well as friction, to be negligible, find the force exerted by the pulley on the ceiling of car.
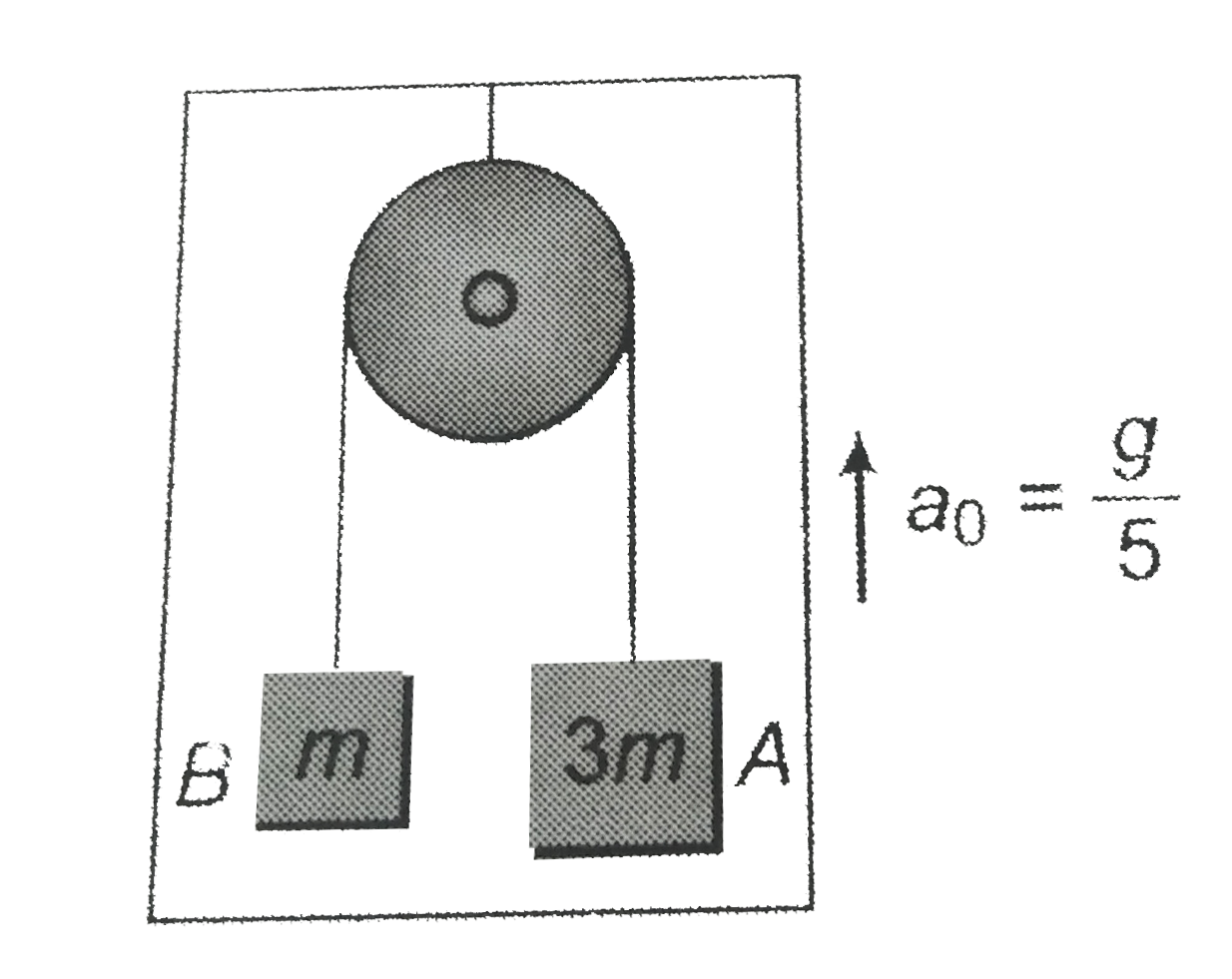 View Solution
View SolutionA pulley fixed to the ceiling of an elevator car carries a thread whose ends are attached to the loads of masses m1 and m2. The car starts going up with an acceleration wo. Assuming the masses of the pulley and the thread, as well as the friction, to be negligible find:
(a) the acceleration of the load m1 relative to the elevator shaft and relative to the car,
(b) the force exerted by the pulley on the ceiling of the car.View SolutionA pulley fixed to the ceiling of an elevator car carries a thread whose ends are attached to the masses m1andm2. The car starts going up with an acceleration a0. Assuming the masses of the pulley and the thread as well as the friction to be negligible, find:
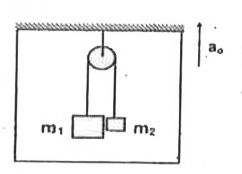
(a) the acceleration of the load m1 relative to the elevator shaft and relative to the car
(b) the force exerted by the pulley on the ceiling of the car. Given m1>m2.View SolutionA pulley fixed to the ceiling of an elevator car carries a thread whose ends are attached to the masses m1andm2. The car starts going up with an acceleration a0. Assuming the masses of the pulley and the thread as well as the friction to be negligible, find:

(a) the acceleration of the load m1 relative to the elevator shaft and relative to the car
(b) the force exerted by the pulley on the ceiling of the car. Given m1>m2.View SolutionA 2kg block hangs without vibrating at the bottom end of a spring with a force constant of 400N/m. The top end of the spring is attached to the ceiling of an elevator car. The car is rising with an upward acceleration of 5m/s2 when the acceleration suddenly ceases at time t=0 and the car moves upward with constant speed (g=10m/s2 The amplitude of the oscillation is
View SolutionA pendulum clock is mounted in an elevator car which starts goint up with a constant acceleration w, with w<g. At a height h the acceleration of the car reverses, its magnitude remaining constant. How soon after the start of the motion will the clock show the right time again ?
View SolutionA 2kg block hangs without vibrating at the bottom end of a spring with a force constant of 400N/m. The top end of the spring is attached to the ceiling of an elevator car. The car is rising with an upward acceleration of 5m/s2 when the acceleration suddenly ceases at time t=0 and the car moves upward with constant speed (g=10m/s2
The amplitude of the oscillation isView Solution

