A top of mass m=1.0kg and moment of inertia relative to its own axis I=4.0g⋅m2 spins with an angular velocity ω=310rad/s. Its point of rest is located on a block which is shifted in a horizontal direction with a constant acceleration w=1.0m/s2. The distance between the point of rest and the centre of inertia of the top equals l=10cm. Find the magnitude and direction of the angular velocity of precession ω′.
The effective g is √g2+w2 inclined at angle tan−1wg with the vertical. Then with reference to the new "vertical" we proceed as in problem. Thus
ω′=ml√g2+w2Iω=0⋅8rad/s.
The vector →'ω forms an angle θ=tan−1wg=6∘ with the normal vertical.
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body
25 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics
25 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions

A rod of mass 2 kg ad length 2 m is rotating about its one end O with an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.
View Solution
A rod of mass 2 kg ad length 2 m is rotating about its one end O wth an angular velocity ω=4rad/s. Find angular momentum of the rod about the axis rotation.
View Solution
Knowledge Check
A disc of radius 0.1 m starts from rest with an angular acceleration of 4.4rad/s2 .Then linear velocity of the point on its after 5 s is
A0.22/sB2.2m/sC4.4m/sD1.1m/sThe moment of an inertia about an axis of a body which is rotating with angular velocity 1rad s−1 is numerically equal to
Aone - fourth of its rotational kinetic energyBhalf of the rotational kinetic energyCrotational kinetic energyDtwice the rotational kinetic energyA body of moment of inertia about its axis of rotation is 3kgm2 and angular velocity 3 rad/s. The kinetic energy of rotating body is same as that of body of mass 27 kg moving with a speed of
A1.0 m/sB0.5 m/sC1.5 m/sD2.0 m/s
A top of mass m=0.50kg, whose axis is tilted by an angle θ=30∘ to the vertical, precesses due to gravity. The moment of inertia of the top relative to its symmetry axis is equal to I=2.0g⋅m2, the angular velocity of rotation about that axis is equal to ω=350rad/s, the distance from the point of rest to the centre of inertia of the top is l=10cm. Find:
(a) the angular velocity of the top's precession,
(b) the magnitude and direction of the horizontal component of the reaction force acting on the top at the point of rest.
View SolutionA uniform sphere of mass m=5.0kg and radius R=6.0cm rotates with an angular velocity ω=1250rad/s about a horizontal axle passing through its centre and fixed on the mounting base by means of bearings. The distance between the bearings equals l=15cm. The base is set in rotation about a vertical axis with an angular velocity ω′=5.0rad/s. Find the modulus and direction of the gyroscopic forces.
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
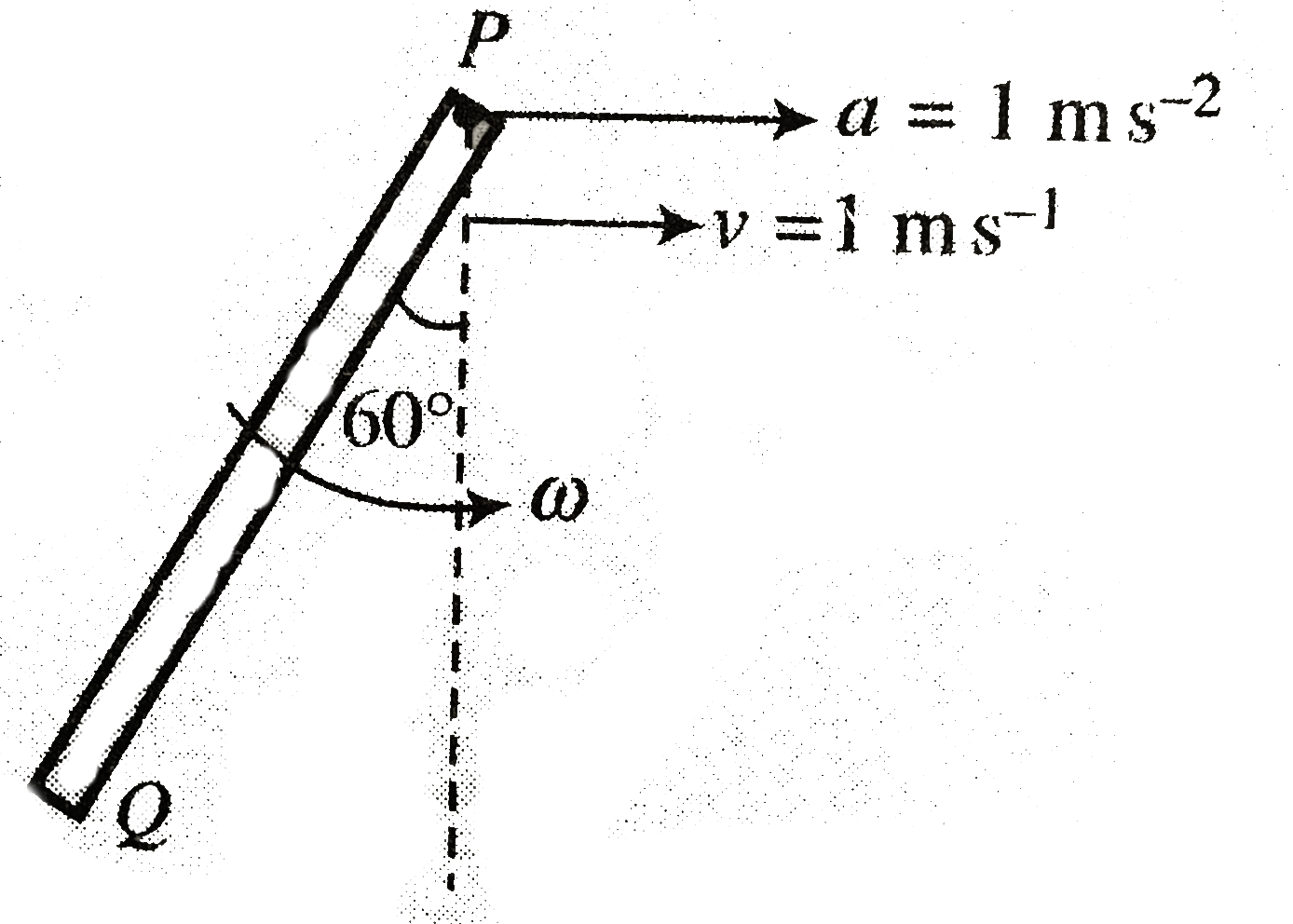
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
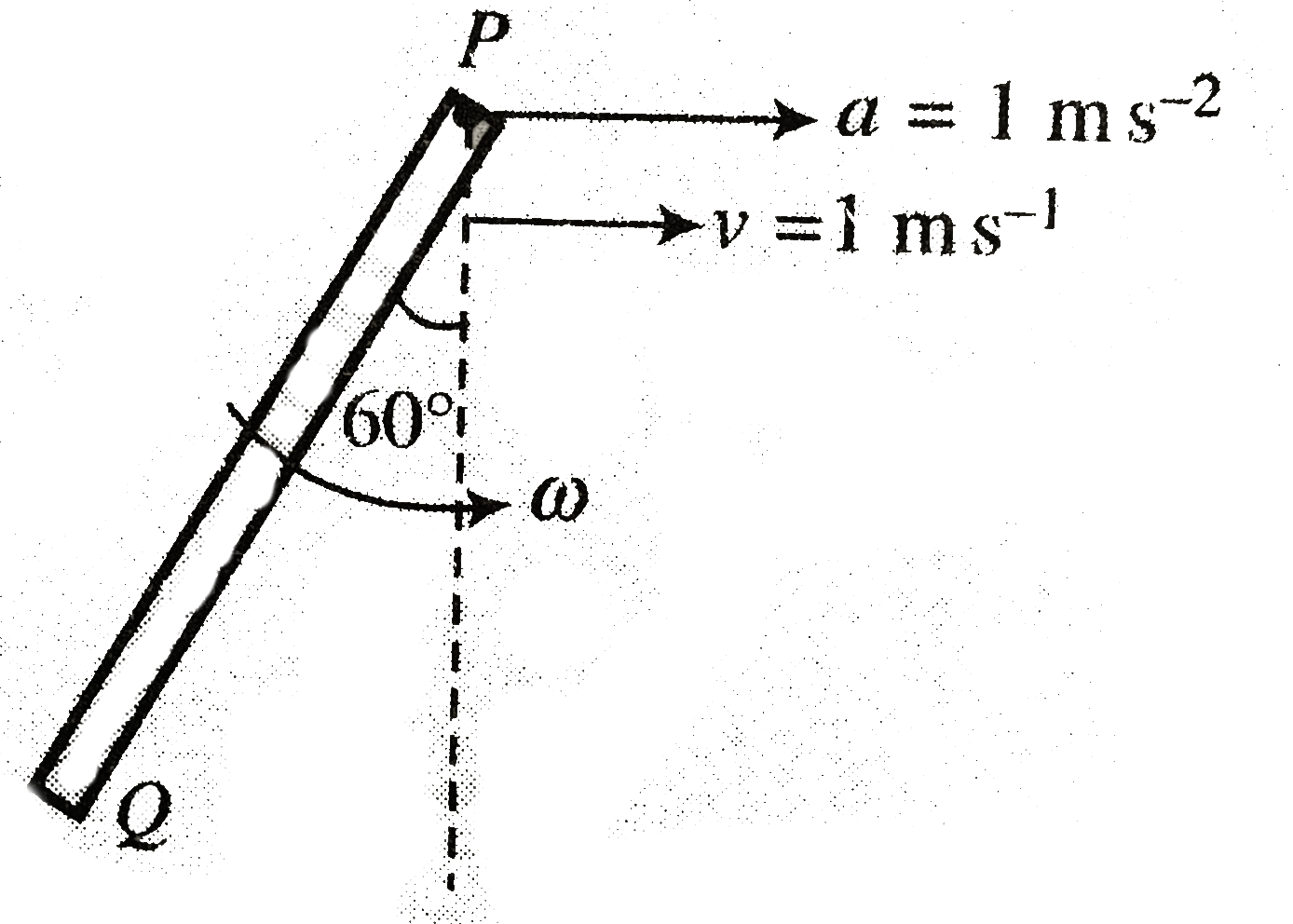
View SolutionA hollow sphere partly filled with water has moment of inertia I when it is rotating about its own axis at an angular velocity ω. If its angular velocity is doubled then its moment of inertia becomes
View Solution
