The space between the plates of a parallel -plate capacitor is filled up with inhomogneous poorly conducting medium whose resistivity varies linearly in the direction perpendicular to the plates. The ratio of the maximum value of resistivity to the minimum one is equal to η The gap width equals d. Find the volume density of the charge in the gap if a voltage V is applied to the capacitor. ε is assumed to be 1 everywhere.
As in the previous problem
Ex=Cρ(x)=C(ρ0−ρ1x)
where ρ0+ρ1dηρ0 or, ρ1=(η−1)ρ0d
By intergation V=∫d0Cρ(x)dx=Cρ0d(1+η−12)=12Cρ0d(η−1)
Thus C=2Vρ0d(η+1)
Thus volume density of charge present in the medium
=dQsdx=ε0dE(x)/dx
=2ε0Vρ0d(η+1)×(η−1)ρ0d=2ε0V(η−1)(η+1)d2
Topper's Solved these Questions
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics
69 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electromagnetic Induction
84 Videos
- ELECTRODYNAMICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Electric Capacitance - Energy Of An Electric Field
46 Videos
- ELECTRICITY AND MAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions
6 Videos
- ELECTROMAGNETISMBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions
24 Videos
Similar Questions
If the plates of a parallel plate capacitor are not equal in area, then
View SolutionThe gap between the plates of a parallel-plate capacitor is filled up with an inhomongeous poorly conducting medium whose conductivity varies lineraly in the direaction perpendicular to the plates form the σ1=1.0pS/m→σ2=2.0pS/m. Each plate has an area S=230cm2, and the separation between the plates is d=2.0mm . Find teh current flowing thorugh the capacitor due to a voltage V=300V.
View Solution
Knowledge Check
If the plates of a parallel plate capacitor are not equal in area then quantity of charge
Aquantity of charge on the plates will be the same, but nature of charge will differ.Bquantity of charge as well as nature of charge on the plates will be differentCquantity of charge on the plates will be different, but nature of charge will be the same.Dquantity of charge as well as nature of charge will be the same.If the gap between the plates of a parallel plate capacitor is filled with medium of dielectric constant k=2 , then the field between them
AIncreases by a factor 2BIncreases by a factor √2CDecreased by a factor √2DDecreased by a factor 1/2In figure half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?
 A
A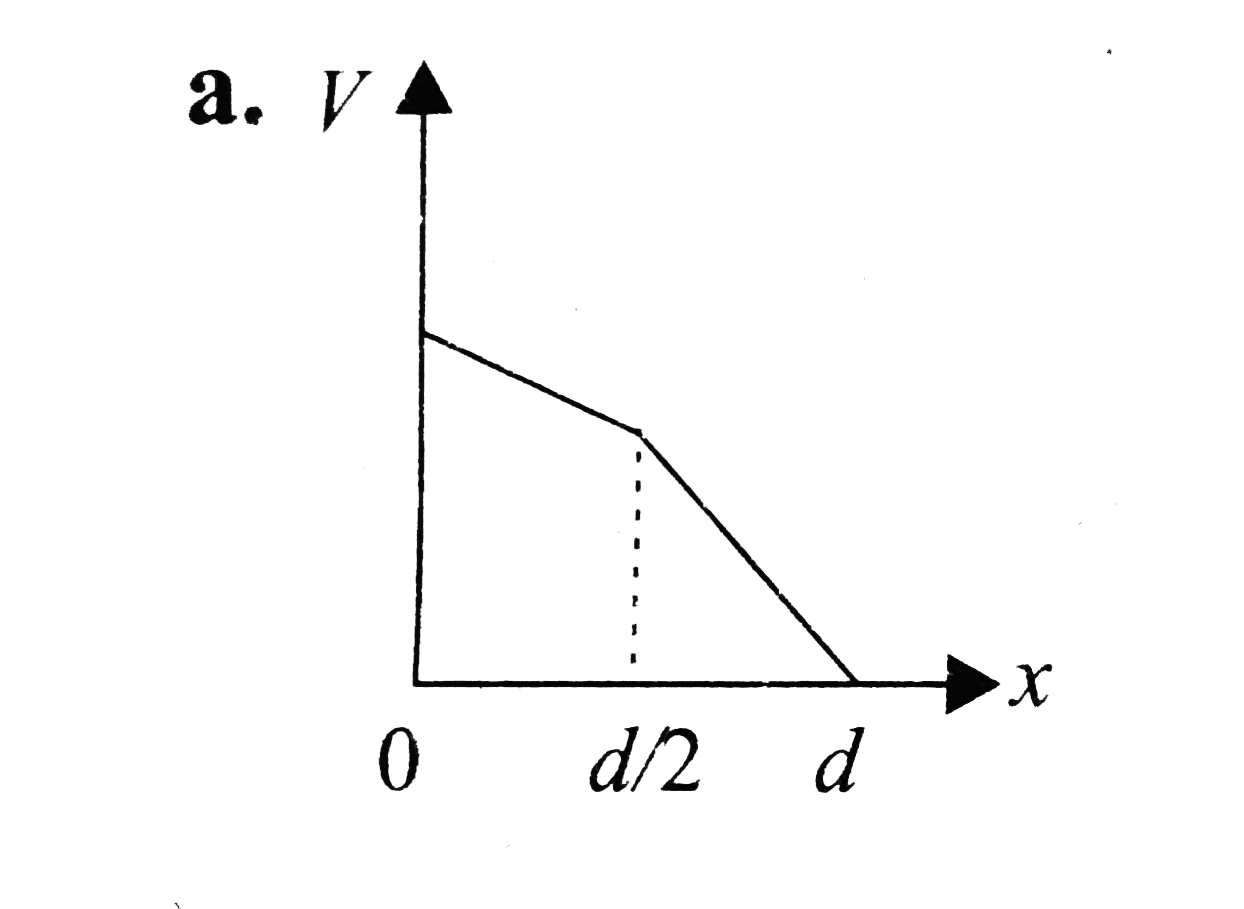 B
B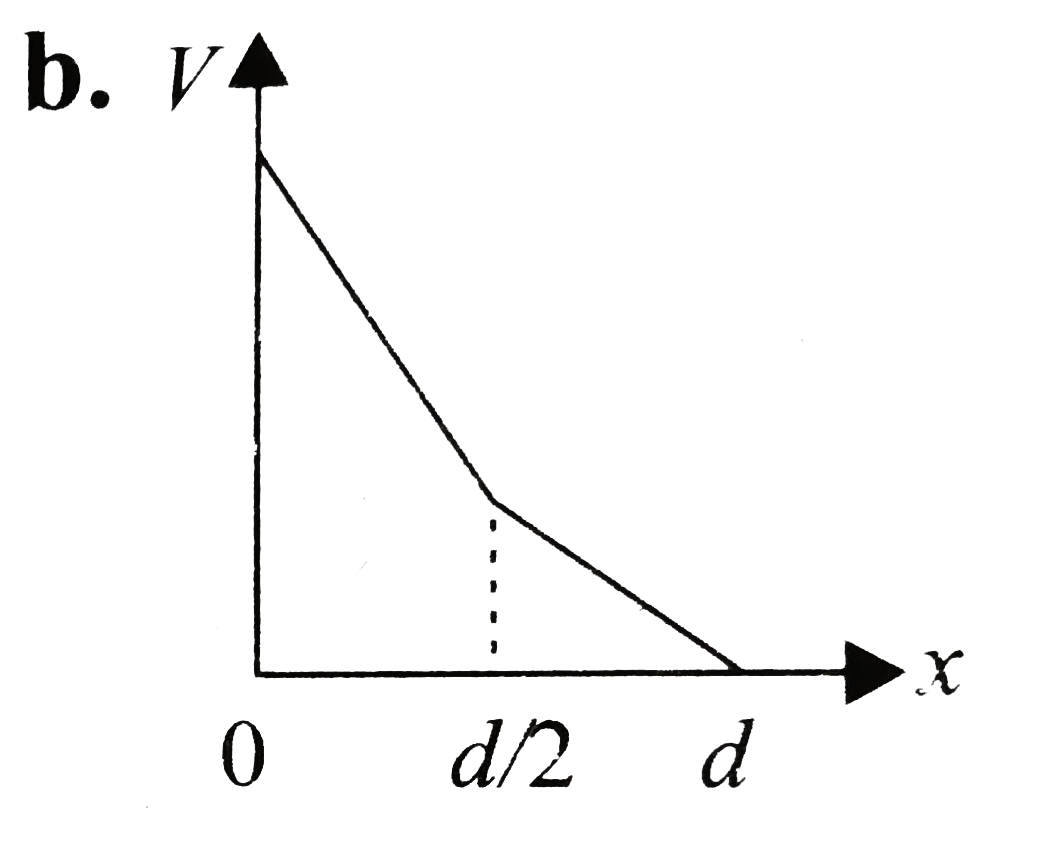 C
C D
D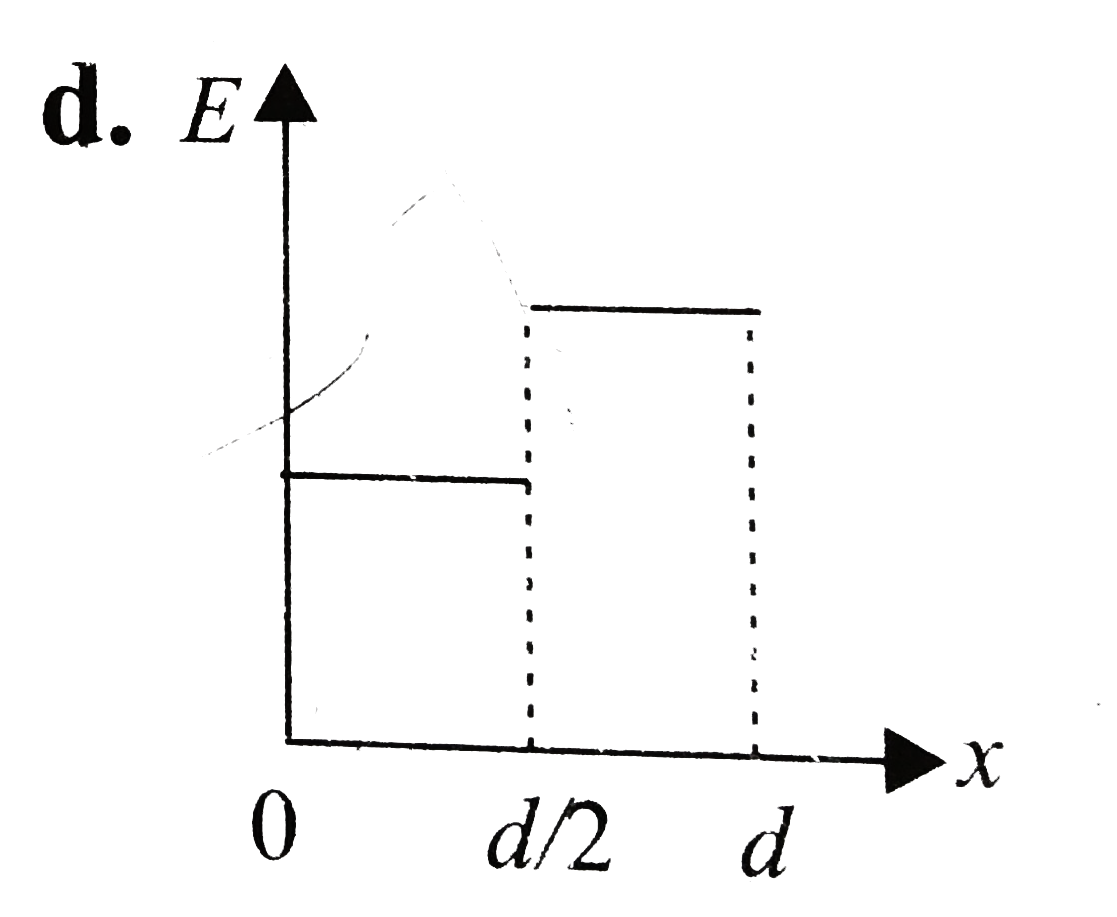
Half of the space between the plates of a parallel plate capacitor is filled with dielectric material of constant K. Then which of the plots are possible?
View SolutionTo reduce the capacitance of parallel plate capacitor, the space between the plate is
View SolutionThe space between the plates of a parallel plate air capacitor is filled with an isotropic dielectric medium of which dielectric constant varies in the direction perpendicular to the plates according to the relation given as
k=k1[1+sinπdx]
Where d is the separation, between the plates and k1 is a constant. The area of the plates is A. Determine the capacitance of the capacitor.
View SolutionThe distance between the plates of a parallel plate capacitor is 'd'. The capacitance of the capacitor gets doubled when a metal plate of thicknessd2 is placed between the plates.
View SolutionTo reduce the capacitance of parallel plate capacitor, the space between the plate is
View Solution
