Demonstarate that the magnetic energy of interaction of two current-carrying loops located in vacumm can be represented as Wia=(1μ0)∫B1B2dV, where B1 and B2 are the magnetic inductions within a volume element dV, produced indiviually by the currents of the first and the secound loop respectively.
The interaction energy is
12μ0∫∣∣∣−→B1+−→B1∣∣∣2dV−12μ0∫∣∣∣−→B1∣∣∣2dV−12μ0∫∣∣∣−→B2∣∣∣2dV
=1μ0∫−→B1.−→B2dV
Here, if −→B1 is the magnetic field produced by the first of the current carrying loops, and −→B2 that of the secound one, then the magnetic field due to both the loops will −→B1+−→B2.
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics69videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
The space within a current carrying toroid is filled with aluminium of magnetic suceptibiliuty 2.1×10−5 what is the percentage increase in the magnetic induction B ?
View SolutionProve the energy stored in a current inductor, per unit volume is B22μ0, where B is the magnetic field inside the inductor.
View Solution
Knowledge Check
- Question 1 - Select One
The magnetic induction at centre of loop due to current flowing through small element 'dl' is given as dB=μ04πIdlsinθr2 where θ is
A0∘B180∘C90∘D60∘ - Question 2 - Select One
Magnetic induction produced at the center of a circular loop of radius R carrying a current is 'B' The magnetic moment of the loop is
Ahigh susceptibility and low retentivityBlow susceptibility and high retentivityClow susceptibility and low retentivityDhigh susceptibility and high retentivity - Question 3 - Select One
A circular loop is placed in magnetic field B=2t. Find the direction of induced current produced in the loop.
 AClockwiseBAnti clockwiseCNo induced currentDrandom direction
AClockwiseBAnti clockwiseCNo induced currentDrandom direction
Figure shows a square current carrying loop ABCD of side 2m and currenti=12A. The magnetic moment →M of the loop is
 View Solution
View SolutionA coaxial cable consists of a thin inner current carrying conductor fixed along the axis of a hollow current conductor, Let B1 and B2 be the magnetic fields in the region between the conductors, and outside the conductor respectively.
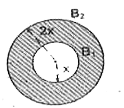 View Solution
View SolutionMagnetic induction at the center of a circular loop carrying a current is 'B' . If 'A' is the area of the coil, the magnetic dipole moment of the loop is
View SolutionThe intensity of magnetic induction at the centre of a current − carrying circular coil is B1 and at a point on its axis at a distance equal to its radius from the centre is B2 , then B1/B2 is
View SolutionThe magnetic moment of a current carrying loop is 2.1×10−25amp×m2. The magnetic field at a point on its axis at a distance of 1Å is
View Solution

