A plane light wave with wavelength λ falls normally on a phase diffraction grating whose side view is shown in Fig. The grating is cut on a glass plate with refractive index n. Find the depth h of the lines at which the intensity of the central Fraunhofer maximum is equal to zero. what is in this case the diffraction angle corresponding to the first maximum?
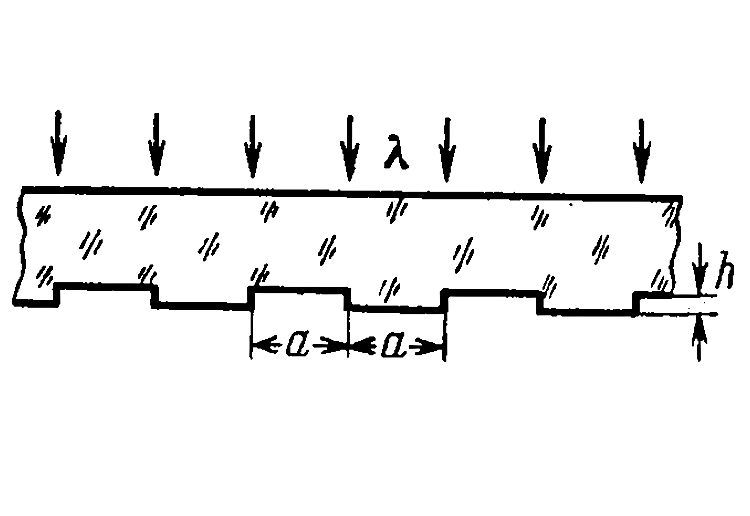
The intensity of the central Fraunhofer maximum will be zero if the waves from successive grooves (not in the same plane) differ in phase by an rod multiple of π. Then since the phase difference is
δ=2πλ(n−1)h
fro the central ray we have
2πλ(n−1)h=(k−12)2π,k=1,2,3,........
or h=λn−1(k−12).
The path difference between the rays 1 & 2 is approximately (neglecuing terms of order θ2)
asinθ+a−na
=asinθ−(n−1)a
Thus for a maximum
asinθ−(k'−12)λ=mλ
or asinθ=(m+k'+12)λ
k'=0,1,2,.........
m=0,±1,±2,......
The first maximum after the central minimum is obtained when m+k'=0
We get asinθ1=12λ

Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Polarization Of Light43videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Dispersion And Absorption Of Light24videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Interference Of Light33videos
OPTICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - MECHANICSEXERCISE - Mechanics Problems92videos
MECHANICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation36videos
OSCILLATIONS AND WAVES
Similar Questions
A plane light wave with wavelength λ=0.65μm falls normally on a large glass plate whose opposite side has a longrectangular recess 0.60mm wide. Using Fig. find the depth H of the recess at which the diffraction pattern on the screen 77cm away from the plate has the maximum illuminance at its centre.
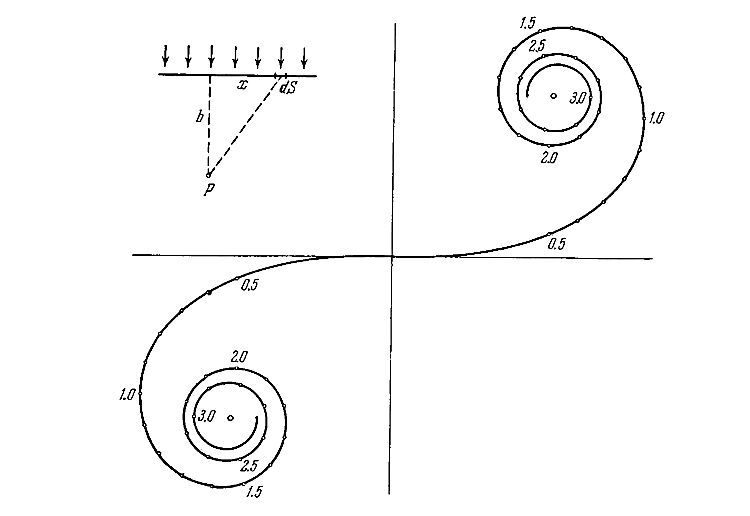 View Solution
View SolutionLight with wavelength 535nm falls normally on a diffraction grating. Find its period if the diffraction angle 35∘ corresponds to one of the Fraunhofer maxima and the highest order of spectrum is equal to five.
View Solution
Knowledge Check
- Question 1 - Select One
A beam of monochromatic light of wavelength λ falls normally on a diffraction grating of line spacing d. If θ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of sinθ ?
AλdBdλC2λdD2dλ
Light with wavelength λ=589.0nm falls normally on a diffraction grating with period d=2.5μm, comprising N=10000 lines. Find the angular width of the diffraction maximum of second order.
View SolutionA plane light wave with wavelength λ=0.60μm falls normally on a sufficiently large glass plate having a round recess on the oppiste side (Fig.) For the observation point P that recess correcponds to the first one and and a half Fresnel zones. Find the depth h of the recess at which the intensity of light at the point P is
(a) maximum,
(b) minimum,
(c ) equal to the intensity of incident light.
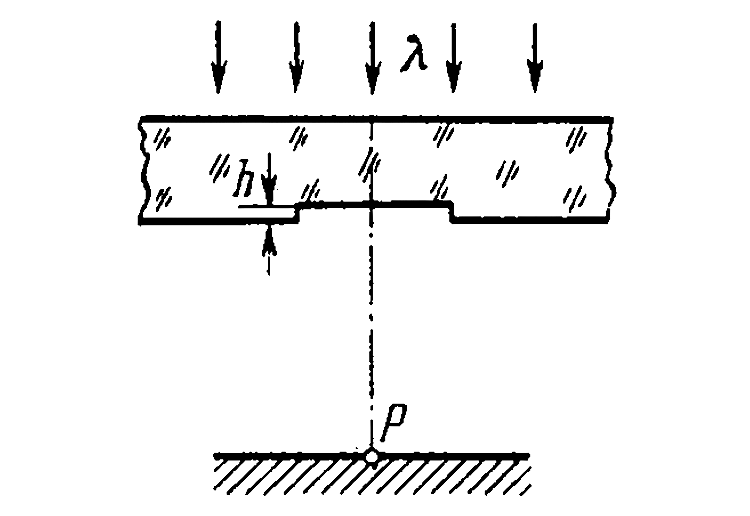 View Solution
View SolutionA plane light wave with wavelength λ=0.60μm and intensity I0 falls normally on a large glass plate whose side view is shown in Fig. At what height h of the ledge will be intensity of light at points located directly below be
(a) minimum,
(b) twice as low as I0 (the losses due to reflection are to be neglected).
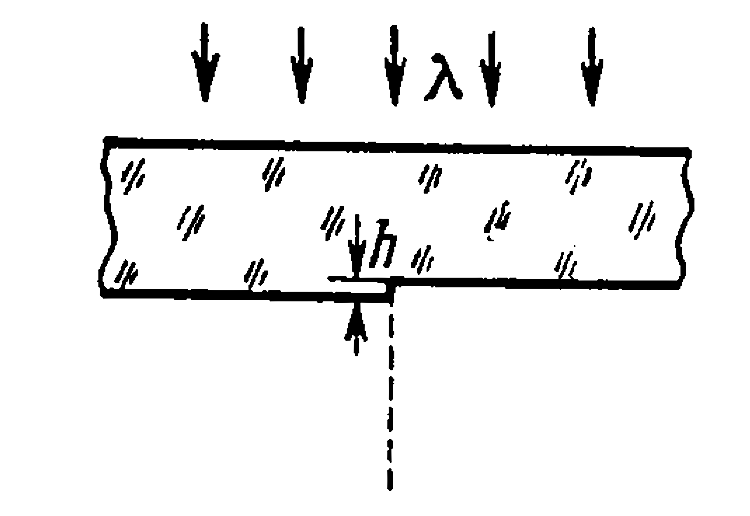 View Solution
View SolutionA plane light wave with wavelength λ and intensity I0 falls normally on a large glass plate whose opposite side serves as an opaque screen with a round aperture equal to the first Fresnel zone for the observation point P. In the middle of the aperture there is a round recess equal to half the Fresnel zone. What must the depth h of that recess be for the intensity of light at the point P to be the highest? What is this intensity equal to?
View SolutionA plane light wave with wavelength λ=0.60μm falls normally on the face of a glass wedge with refracting angle Θ=15∘. The opposite face of the wedge is opaque and has a slit of width b=10μm parallel to the edge. Final:
(a) the angle Δθbetween the direction to the Fqaunhofer maximum of zeroth order and that of incident light,
(b) the angular width of the frqunhofer maximum of the zeroth order.View SolutionLight with wavelength λ=0.60μm falls normally on a diffraction grating inscribed on a plane surfcae of a plano-curvex cylinderical glass lens with curvature radius R=20cm. The period of the grating is equal to d=6.0μm. Find the distance between the principle maxima of first order located symmetrically in the focal plane of that lens.
View SolutionA plane light wave with wavelength λ=0.65μm falls normally on a large glass plate whose opposite side has a large and an opaque strip of width a=0.30mm (Fig.) A screen is placed at a distance b=110cm from the plate. The height h of the ledge is such that the intensity of light at point 2 of the screen is the heighest possible. making use of Fig. find the ratio of intensities at point 1 and 2.
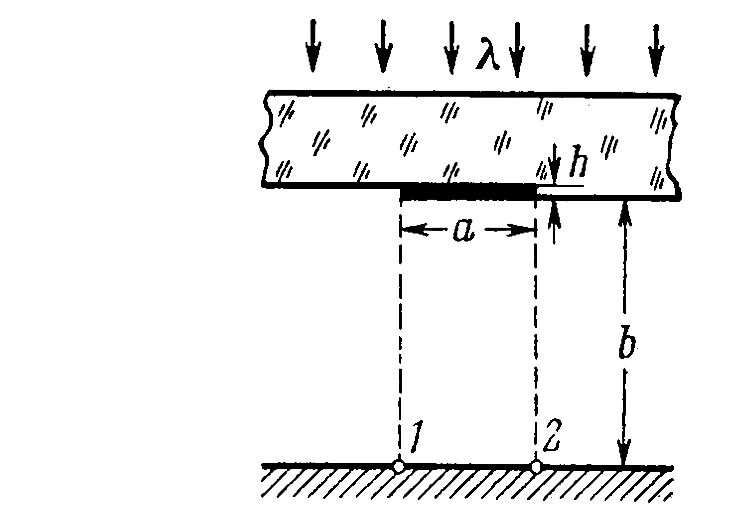
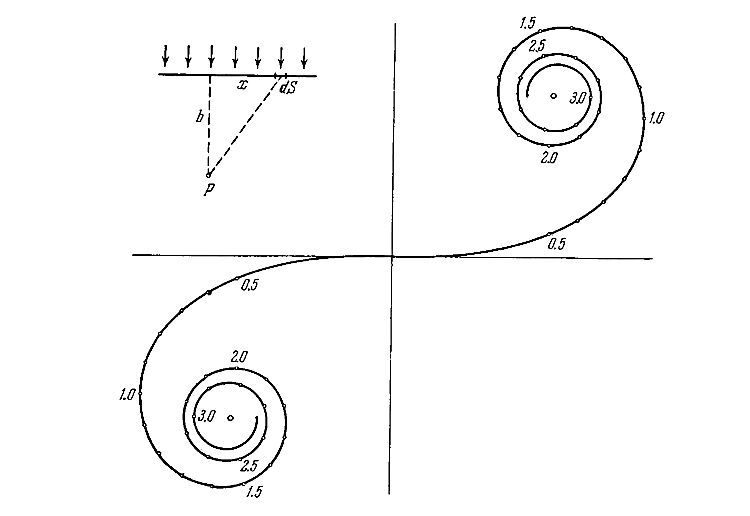 View Solution
View Solution

