From the condition of the foregoing problem find:
(a) normalized eigenfunctions of the particle in the states for which Ψ(r) depends only on r ,
(b) the most probable value rpr for the ground state of the particle and probability of the particle to be in in the region r<rpr
(a) The nomalized wave functions are obtained from the normalization
1=∫|Ψ|2dV=∫|Ψ|24πr2dr
=∫ro0A24πχ2dr=4πA2∫ro0sin2nπrrodr
=4πA2r0nπ∫¯¯¯¯¯nπ0sin2xdr=4πA2r0nπ.nπ2=r0.2πA2
Hence A=1√2πr0 andΨ=1√2π.r0sinnπrr0r
(b) The radial probability distribution function is
Pn(r)=4πr2(Ψ)2=2r0sin2nπrr0
For the ground state n=1
so P1(r)=2r0sin2πrr0sin2πrr0
By inspection this is maximum for r=r02. Thus rpr=r02
The probability for the particle to be found in the region r<rpr is clearly 50% as one can immediately see from a graph of sin2x.
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Properties Of Atom
69 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Molecules And Crystals
48 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Elementary Particles
20 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
Show that the radial probability density for the ground state of the hydrogen atom has maximum at r = a.
View SolutionThe potential energy of a particle in a certain field has the form U=a/r2−b/r, where a and b are positive constants, r is the distance from the centre of the field. Find:
(a) the value of r0 corresponding to the equilibrium position of the particle, examine where this position is steady,
(b) the maximum magnitude of the attraction force, draw the plots U(r) and Fr(r) (the projections of the force on the radius vector r).
View Solution
Knowledge Check
The potential energy of a particle in a force field is:
U=Ar2−Br,. Where A and B are positive
constants and r is the distance of particle from the centre of the field. For stable equilibrium the distance of the particle isAabBbaC2abD2baA particle is released from the top of the smooth hemisphere R as shown.
the normal contact between the particle and the hemisphere in position θ is
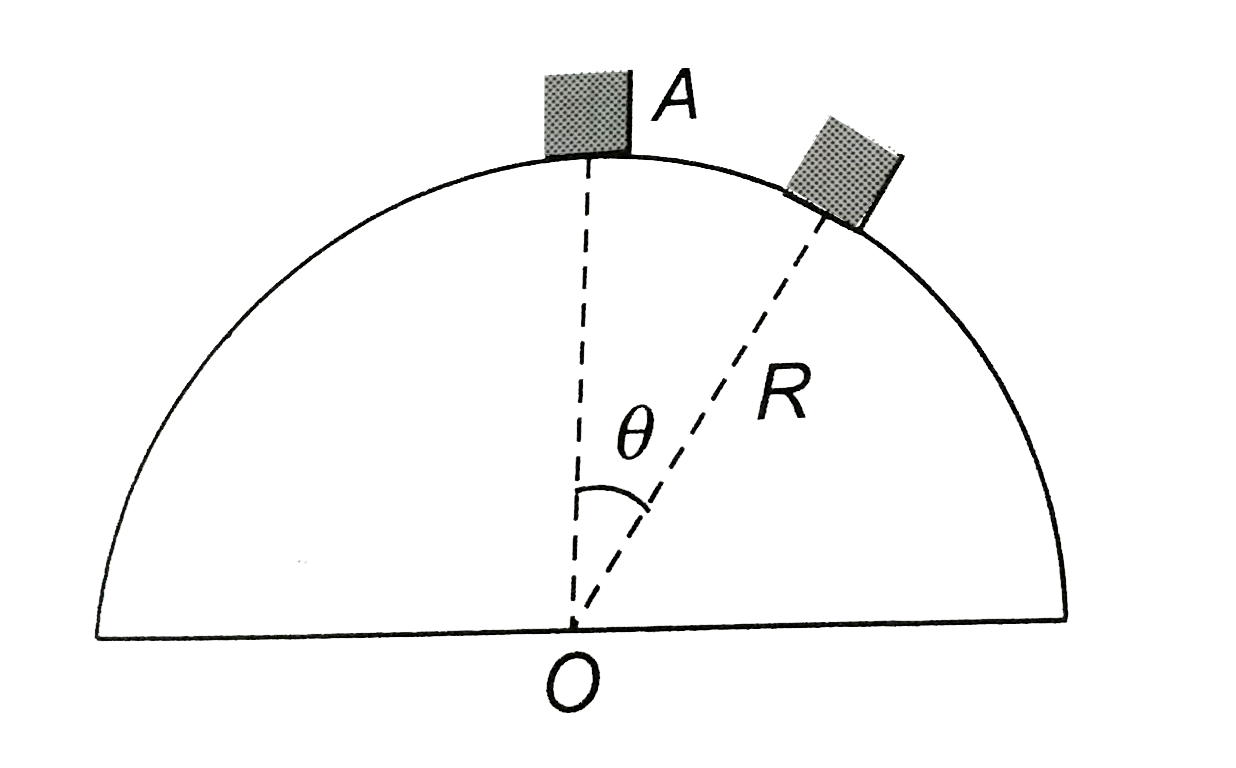 Amg(3−2cosθ)Bmg(3cosθ−2)Cmg(4cosθ−3)Dmg(4−3cosθ)
Amg(3−2cosθ)Bmg(3cosθ−2)Cmg(4cosθ−3)Dmg(4−3cosθ)The potential energy of a particle in a certain field has the form U=a/r2−b/r, where a and b are positive constants, r is the distance from the centre of the field. Find:
(a) the value of r0 corresponding to the equilibrium position of the particle, examine where this position is steady,
(b) the maximum magnitude of the attraction force, draw the plots U(r) and Fr(r) (the projections of the force on the radius vector r).A2abB2abC−abDab
The potential energy of a particle in a conservative field is U=ar3−br2 where a and b are positive constants and r is the distance of particle from the centre of field. For equilibrium, the value of r is
View SolutionLet an ordinary coin be tossed 15 times. If Pr denotes the probability of getting r tails and Pk is maximum then k = _____.
View SolutionThe potential energy of a particle in a conservative field is U =a/r^3-b/r^2, where a and b are positive constants and r is the distance of particle from the centre of field. For equilibrium, the value of r is
View SolutionThe potential energy of a particle in a force field is:
U=Ar2−Br,. Where A and B are positive
constants and r is the distance of particle from the centre of the field. For stable equilibrium the distance of the particle is
View SolutionThe potential energy of a particle in a conservative field is U=ar3−br2 , where a and b are positive constants and r is the distance of particle from the centre of field. For equilibrium, the value of r is
View Solution
