Find the possible values of energy of a paraticle of mass $m$ located in s spheri

Text Solution

Verified by Experts

The schrodinger equation is
$\nabla^{2} Ψ + \frac{2 m}{ħ^{2}} (E - U (r)) Ψ = 0$
when $Ψ$ depends on $t$ only $\nabla^{2} Ψ = \frac{1}{r^{2}} \frac{d}{d r} (r^{2} \frac{d Ψ}{d r})$
If we put $Ψ = \frac{χ (r)}{r}, \frac{d Ψ}{d r} = \frac{χ'}{r} - \frac{χ}{r^{2}}$
and $\nabla^{2} Ψ = \frac{χ'}{r}$ . Thus we get
$\frac{d^{2} χ}{d r^{2}} + \frac{2 m}{ħ^{2}} (E - U (r)) χ = 0$
The solution is $χ = A \sin k r, r < r_{0}$
$k^{2} = \frac{2 m E}{ħ^{2}}$
and $χ = 0 r > r_{0}$
(for $r < r_{0}$ we have rejected a term $B \cos k r$ as it does not vanish at $r = 0$ ). Continuity of the wavefunction at $r = r_{0}$ requires
$k r_{0} = n π$
Hence $E_{n} = \frac{n^{2} π^{2} ħ^{2}}{2 m r_{0}^{2}}$ .

Updated on:21/07/2023

The energy of a particle moving at 5 m/s is 125 J. The mass is
A4kg
B6 kg
C10 kg
D25 kg
Find the potential energy of the gravitational interaction of a point mass $m$ and a rod of mass $m$ and length $l$ , if they are along a straight line. Point mass is at a distance of a from the end of the rod.

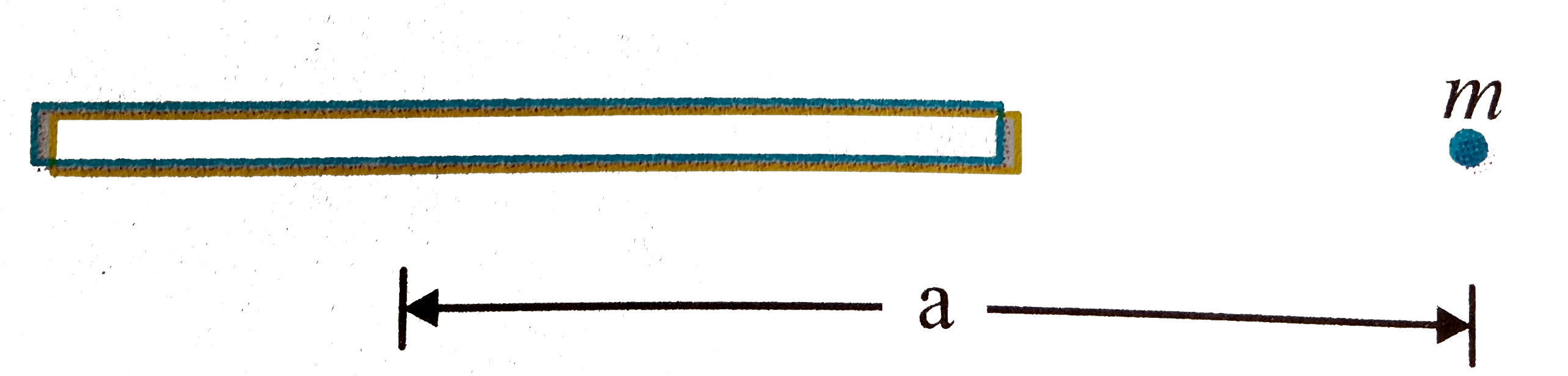
Find the potential energy of the gravitational
A $U = \frac{- G m^{2}}{l} ln (\frac{2 a + l}{2 a - l})$
B $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a + l}{2 a - l})$
C $U = \frac{- G m^{2}}{l^{2}} ln (\frac{2 a - l}{2 a + l})$
D $U = \frac{- G m^{2}}{l^{2}} {ln (\frac{2 a - l}{2 a + l})}^{2}$
If the mass and kinetic energy of a particle are m and E respectively, then the value of its momentum is-
A $\sqrt{m E}$
B $\sqrt{2 m E}$
C $\sqrt{2 E / m}$
D $\sqrt{2 m / E}$