Find the energy of an electron fo a hydrogen atom in a stationary state for which the wave function takes the from Ψ(r)=A(1+ar)e−αr, where A,aandα are constant.
The Schrodinger equation for the problem in Gaussian units
∇2Ψ+2mħ2[E+e2r]Ψ=0
In MKS units we should read (e2/4πε0) for e2
we put Ψ=χ(r)r. Then χ''+2mħ2[E+e2r]xho=0 ....(1)
We are given that χ=rΨ=Ar(1+ar)e−ar
so χ'=A(1+2ar)e−ar−αAr(1+ar)e−ar
χ''=α2Ar(1+ar)e−ar−2αA(1+2ar)+2aAe−ar
Substituting in (1) gives the condition
α2(r+ar2)=2α(1+2ar)+2a+2mħ2=0....(2)
aα2+2mħ2Ea=0....(3)
a2−4aα+2mħ2(E+e2a)=0 (4)
From (3) either a=0,
In the first case α=me2ħ2=−ħ22mα2=−me42ħ2
This state is the ground state.
a=α−me48ħ2 and a=−12me2ħ2
This state is one with n=2(2s).
Topper's Solved these Questions
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Properties Of Atom
69 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Molecules And Crystals
48 Videos
- ATOMIC AND NUCLEAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ATOMIC AND NUCLEAR PHYSICSEXERCISE - Elementary Particles
20 Videos
- DIRECT CURRENTBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - DIRECT CURRENTEXERCISE - All Questions
15 Videos
Similar Questions
Find the mean electrostatic potential produced by an electron in the centre of a hydrogen atom if the electron is in the ground state for which the wave function is Ψ(r)=Ae−r/r1, where A is a certain constant, r1 is the first Bohr radius.
View SolutionThe wave fucnction of an electron of a hydrogen atom in the ground state takes the from Ψ(r)=Ae−r/r1, where A is a certain constant, r1 is the first Bohr radius. Find:
(a) the most probable distance between the electron and the nucleus.
(b) the mean value of modulus of the Coulomb force acting on the electron
(c )the mean value of the potential energy of the electron in the field of the nucleus.
View Solution
Knowledge Check
The total energy (E) of the electron is an orbit of radius r in hydrogen atom is
Ae28πε0rB−e24πε0rC−e28πε0rDzeroThe kinetic energy of the electron in an orbit of radius r in hydrogen atom is (e= electronic charge)
Ae2r2Be22rCe2rDe22r2
Find the current density as a funciton of distance r from the axis of a radially symmetrical parallel stream of electrons if the magnetic induction inside the streams varies as B=brα, where bandalpha are positive constants.
View SolutionThe total energy (E) of the electron is an orbit of radius r in hydrogen atom is
View SolutionThe wavefunction of a particle of mass m in a unidimensional potential field U(x)=kx2/2 has in the ground state the form Ψ(x)=Ae−αx2, where A is a normalization factor and α is positive constant. Making use of a Schrodinger equation, find the constant α and the energy E of the particle in this state.
View SolutionEnergy of electron in first excited state in Hydrogen atom is −3.4eV. Find K.E. and P.E. of electron in the ground state.
View SolutionA space is filled up with a charge with volume desnsity ρ=ρ0e−αr3, where ρ0 and α are positive constansts, r is the distance from the centre of this system. Find the magnitude of the electric field strength vector as a function of r. Investigate the obtained expresssion for the small and large values of r,i.e., at αr3<<1 and αr3>>1.
View SolutionRead the pasage given below as well as the adjoining energy level diagram and then answer the questions given after the passage .
As per Bohr's theory og hydrogen atom the energy of an atom in a state corresponding to principal quantum number n is given as :
En=−13.6n2eV
The energy of an atom is the least when its electron is revolving in an orbit closets to the nucleus i.e., the one for which n =1. This state is called the ground state. When a hydrogen atom receives energy by processes such as electron collision , the atom may acquire sufficient energy to raise the electron to higher energy states and the atom is then said to be in an excited state . From these excited states the electron can then fall back to a state of lower energy , emitting a photon in the process.
The energy level diagram for the stationary states of a hydrogen atom , compouted from Bohr's relation for energy , is given in Fig.12.02. The principal quantum number n labels the stationary states in the ascending order of energy . Obviously , the highest energy corresponds to n=∞ and has an energy of 0 eV. This is the energy of the atom when the electron is completely removed (r=∞) from the nucleus and is at rest.
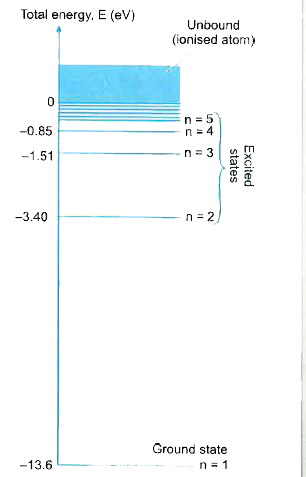
Now answer the follwoing questions:
Write the formula for spectral lines the of hydrogen atom in visible light in terms of Rydberg's constant.
View Solution
