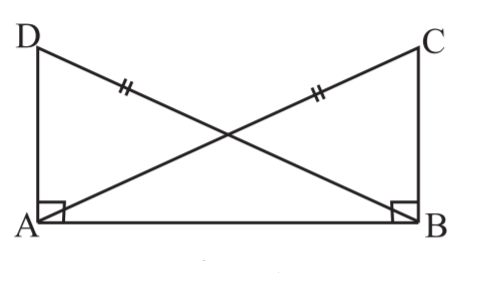
In the figure, AB=AD and BC=DC (i) State three pairs of equal parts in △ABC and △ADC (ii) Is △ABC≅△ADC? Give reasons (iii) Does AC bisect ∠BAD? Give reasons
AB=AD
BC=DC
1)In∠ABC and ∠ADC
AB=AD
BC=DC
AC=AC
2)∠ABC≅∠ADC(SSS)
3)∠BAC=∠DAC
AC bisects the ∠BAD.
Similar Questions
In the figure, AB=AD and BC=DC (i) State three pairs of equal parts in △ABC and △ADC (ii) Is △ABC≅△ADC? Give reasons (iii) Does AC bisect ∠BAD? Give reasons
View SolutionIn Fig 7.23, AB=ACandAD is the bisector of ? BAC. (i) State three pairs of equal parts in triangles ADBandADC. (ii) Is ΔADB[≡~?]ΔADC ? Given reasons. (iii) Is ∠B=∠C ? Given reasons
View SolutionIn the given figure, AB=ACandAD is the bisector of ? BAC. (i) State three pairs of equal parts in triangles ADBandADC. (ii) Is ΔADB≅ΔADC ? Given reasons. (iii) Is ∠B=∠C ? Given reasons
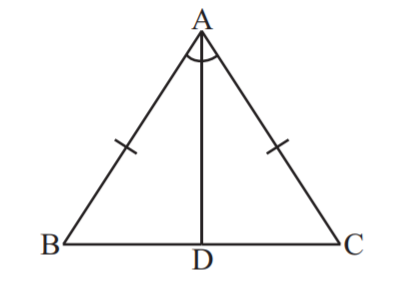
View SolutionIn the given figure, AD=CDandAB=CB. (i) state the three pairs of equal in ΔABDandΔCBD. (iii) Is ΔABD[≡~?]ΔCBD ? Why or why not ? (iii) Does BD bisect ? ABC ? Given reasons
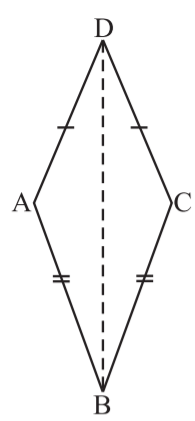
View SolutionIn fig 7.13, AD=CDandAB=CB. (i) state the three pairs of equal in ΔABDandΔCBD. (iii) Is ΔABD[≡~?]ΔCBD ? Why or why not ? (iii) Does BD bisect ? ABC ? Given reasons
View SolutionObserve Figure and state the three pairs of equal parts in triangles ABC and DBC.
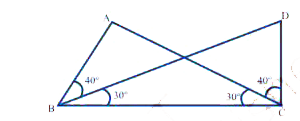
(i) IsΔABC≅ΔDCB? Why? (ii) Is AB = DC? Why? (iii) Is AC = DB? Why
View Solution△ ABC and △ DBC are isosceles triangles on the same base BC. Prove that line AD bisects BC at right angles
View SolutionIn the given figure . If AB=CD,AD=BC then prove that △ADC≅△CBA
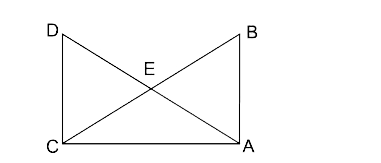
View SolutionIn Fig 7.31, DA⊥AB,CB⊥ABandAC=BD. State the three pairs of equal parts in ΔABCandΔDAB. Which of the following statements is meaningful? (i) ΔABC[_≅?]ΔBAD (ii) ΔABC[_≅?]ABD
View SolutionIn Fig 7.31, DA⊥AB,CB⊥ABandAC=BD. State the three pairs of equal parts in ΔABCandΔDAB. Which of the following statements is meaningful?
(i) ΔABC≅ΔBAD
(ii) ΔABC≅ABD
View Solution
