In Fig 7.23, AB=ACandAD is the bisector of ? BAC. (i) State three pairs of equal parts in triangles ADBandADC. (ii) Is ΔADB[≡~?]ΔADC ? Given reasons. (iii) Is ∠B=∠C ? Given reasons
To solve the given question step by step, we will analyze the information provided and apply the properties of congruent triangles.
Step 1: Identify the Equal Parts in Triangles ADB and ADC
We need to state three pairs of equal parts in triangles ADB and ADC.
1. AB = AC (Given)
2. AD = AD (Common side)
3. ∠BAD = ∠CAD (AD is the bisector of ∠BAC)
Step 2: Check for Congruence of Triangles ADB and ADC
Now, we need to determine if triangle ADB is congruent to triangle ADC.
- We have identified:
- One pair of equal sides: AB = AC
- Another pair of equal sides: AD = AD (common side)
- One pair of equal angles: ∠BAD = ∠CAD
Using the SAS (Side-Angle-Side) congruence criterion, we can conclude:
- Triangle ADB ≅ Triangle ADC (by SAS congruence rule)
Step 3: Determine if Angle B Equals Angle C
Finally, we need to check if ∠B = ∠C.
Since triangles ADB and ADC are congruent, by the property of congruent triangles, we know that corresponding parts of congruent triangles are equal.
- Therefore, ∠B = ∠C.
Summary of the Solution
(i) The three pairs of equal parts in triangles ADB and ADC are:
- AB = AC
- AD = AD
- ∠BAD = ∠CAD
(ii) Yes, triangle ADB is congruent to triangle ADC by the SAS congruence rule.
(iii) Yes, ∠B = ∠C because corresponding parts of congruent triangles are equal.
---
We need to state three pairs of equal parts in triangles ADB and ADC.
2. AD = AD (Common side)
3. ∠BAD = ∠CAD (AD is the bisector of ∠BAC)
Now, we need to determine if triangle ADB is congruent to triangle ADC.
- One pair of equal sides: AB = AC
- Another pair of equal sides: AD = AD (common side)
- One pair of equal angles: ∠BAD = ∠CAD
- Triangle ADB ≅ Triangle ADC (by SAS congruence rule)
Finally, we need to check if ∠B = ∠C.
- Therefore, ∠B = ∠C.
(i) The three pairs of equal parts in triangles ADB and ADC are:
- AB = AC
- AD = AD
- ∠BAD = ∠CAD
Topper's Solved these Questions
Similar Questions
In fig 7.13, AD=CDandAB=CB. (i) state the three pairs of equal in ΔABDandΔCBD. (iii) Is ΔABD[≡~?]ΔCBD ? Why or why not ? (iii) Does BD bisect ? ABC ? Given reasons
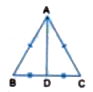
View SolutionIn the adjoining figure, ΔABC is an isosceles triangles in which AB=AC and AD is a median. Prove that :
(i) ΔADB≅ΔADC
(ii) ∠BAD=∠CAD
View SolutionIn Fig 7.31, DA⊥AB,CB⊥ABandAC=BD. State the three pairs of equal parts in ΔABCandΔDAB. Which of the following statements is meaningful? (i) ΔABC[_≅?]ΔBAD (ii) ΔABC[_≅?]ABD
View SolutionABC is an isosceles triangle with AB=AC and D is a point on ABC BC such that AD⊥BC (see figure ). To prove that ∠BAD=∠CAD a student proceeded as follows
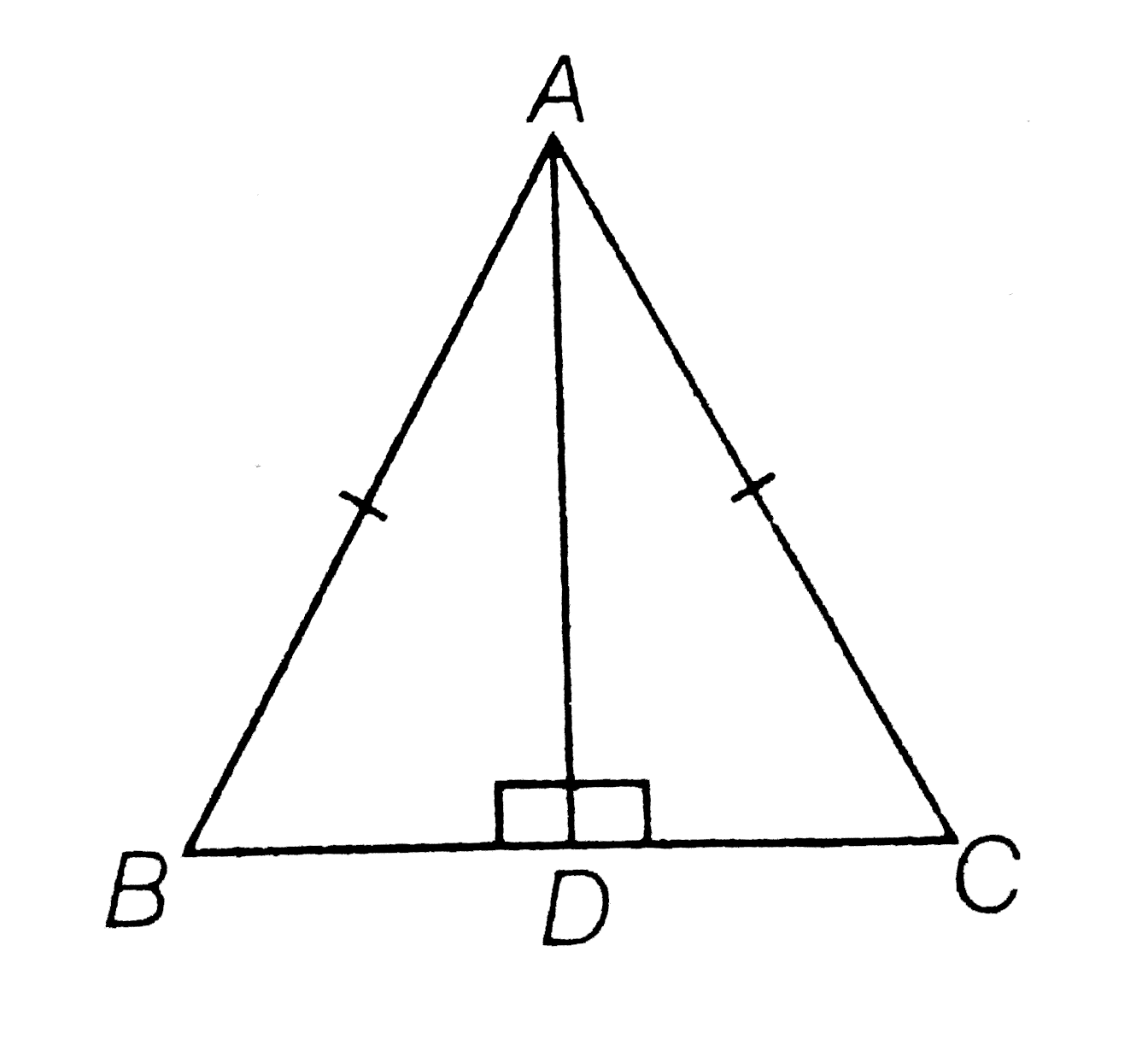
InΔABDandΔACD,we have
AB=AC [ Given]
∠B=∠C [∵ AB=AC ]
and ∠ADB=∠ADC
Therefore ΔABD≅ΔACD [by AAS congruence rule]
So , ∠BAD=∠CAD [byCPCT]
What is the defect in the above argument ?
View SolutionIn the adjoining figure, ΔABC is an isosceles triangles in which AB=AC and AD is the bisector of ∠A. Prove that :
(i) ΔADB≅ΔADC
(ii) ∠B=∠C
(iii) BD=DC
(iv) AD⊥BC

View SolutionIn figure, AB∣∣DC and AB=DC. (i)IsΔACD≅ΔCAB? (ii)State the three pairs of matching parts used to answer (iii) Which angle is equal to∠CAD ? (iv) Does it follow from (iii) that A D || B C ?
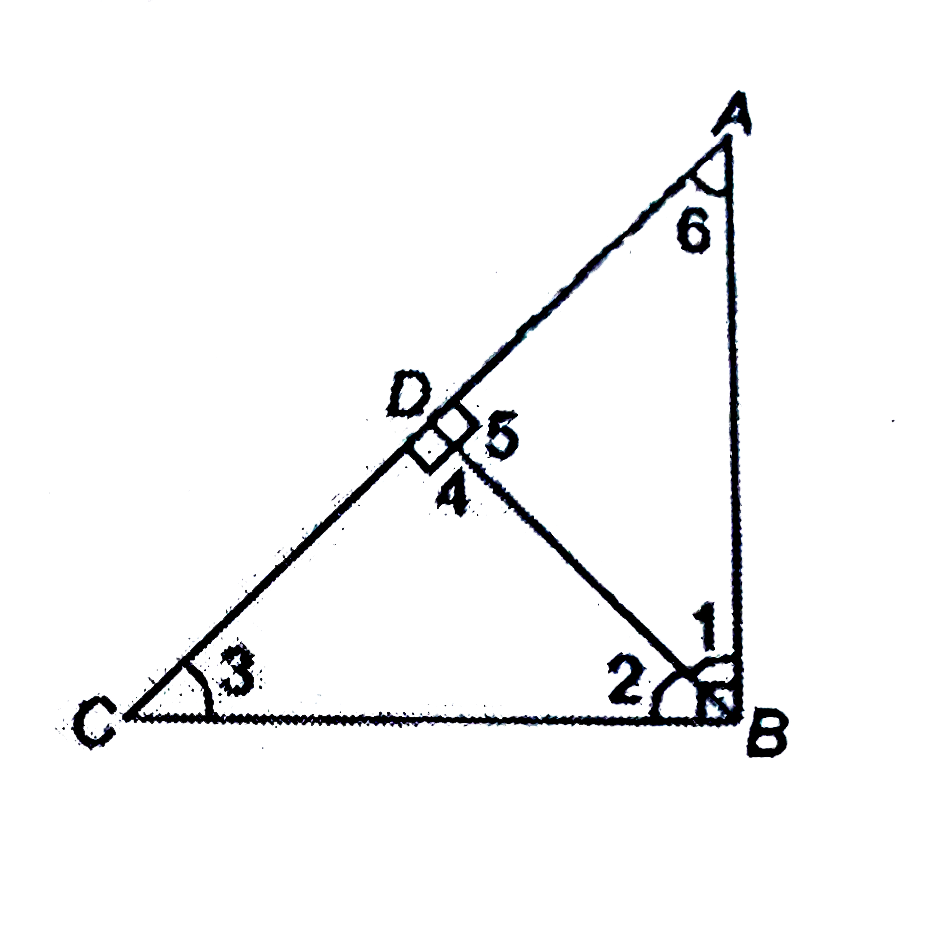
View SolutionIn the given figure if ∠B=90∘ and BD is perpendicular to AC then
prove that:
(i)△ADB~△BDC(ii)△ADB~△ABC
(iii) △BDC~△ABC(iv)BD2=AD×DC
(v) AB2=AD×AC(vi)BC2=CD×AC
(vii) AB2+BC2=AC2
View SolutionIn Fig.39, AO=OB and∠A=∠B. (i) IsΔAOC≅ΔBOD? (ii) State the matching pair you have used, which is not given in the question. (iii) Is it true to say that∠ACO=∠BDO?
View SolutionΔABCand ΔDBCare two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig. 7.39). If AD is extended to intersect BC at P, show that (i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC

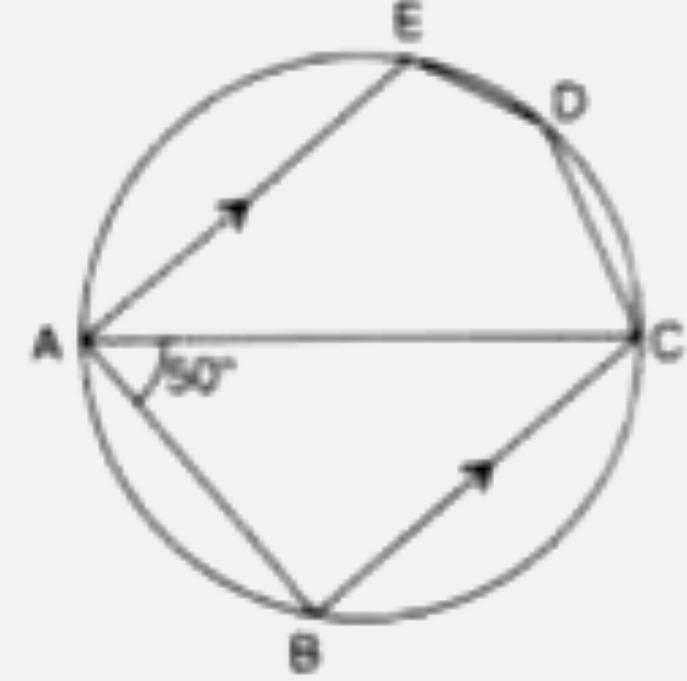
View SolutionIn the given figure, ABCDE is a pentagone inscribed in a circle such that AC is a diameter and side BC//AE. If ∠BAC=50∘, find giving reasons :
(i) ∠ACB
(ii) ∠EDC
(iii) ∠BEC
Hence prove that BE is also a diameter.
View Solution
