A particle executing linear SHM has velocities at dis"tan"ce , respectively from the mean position. The angular velocity of the particle is
The correct Answer is:B
Topper's Solved these Questions
Similar Questions
A particle executing SHM has velocities v1,v2 and accelerations f1,f2 at two of its positions A and B on the same side of the equilibrium position. Find the angular frequency of the SHM.
View SolutionA particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
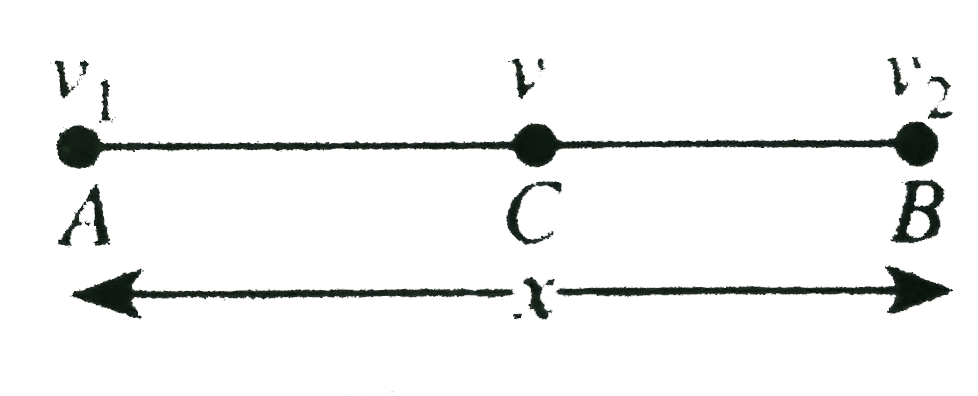 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
A particle executing linear S.H.M. has velocities v1 and v2 at distances x1 and x2 respectively from the mean position. The angular velocity of the particle is
A ⎷x21−x22v22−v21B ⎷v22−v21x21−x22C ⎷x21+x22v22+v2D ⎷v22+v21x22+x21 - Question 2 - Select One
A particle is executing a linear S.H.M. v1 and v2 are as speeds at dis"tan"ces x1 and x2 from the equilibrium position. What is its ampiltude of oscillation ?
AA= ⎷v21x22−v22x21v21−v22BA= ⎷v21−v22v21x22−v22x21CA=v21x22−v22x21v21−v22DA= ⎷v21x21−v22x22v21−v22 - Question 3 - Select One
A particle is executing a linear SHM. v1 and v2 are its velocities at dis"tan"ce x1 and x2 from the equilibrium. What is its period of oscillation ?
AT=2π ⎷x22−x21v21−v22BT=2π ⎷v21−v22x22−x21CT=2π(v1−v2)(x2−x1)DT=2π ⎷(x2−x1)2(v1−v2)2
A particle executing harmonic motion is having velocities v1 and v2 at distances is x1 and x2 from the equilibrium position. The amplitude of the motion is
View SolutionA particle executing a linear SHM has velocities of 8 m/s 7 m/s and 4 m/s, respectively at three points at dis"tan"ces of x m, (x+1) m and (x+2)m from the mean position. What is the maximum velocity of the particle ?
View SolutionA particle is executing SHM along a straight line. Its velocities at distances x1 and x2 from the mean position are v1 and v2, respectively. Its time period is
View SolutionA particle is executing S.H.M. Ifu1 and u2 are the velocitiesof the particle at distancesx1 andx2 from the mean position respectively, then
View SolutionThe periodic time of a particle executing a linear S.H.M. is 2πω. If its velocity at a dis"tan"ce b from the mean position is √3bω, then the path length of the particle is
View Solution

