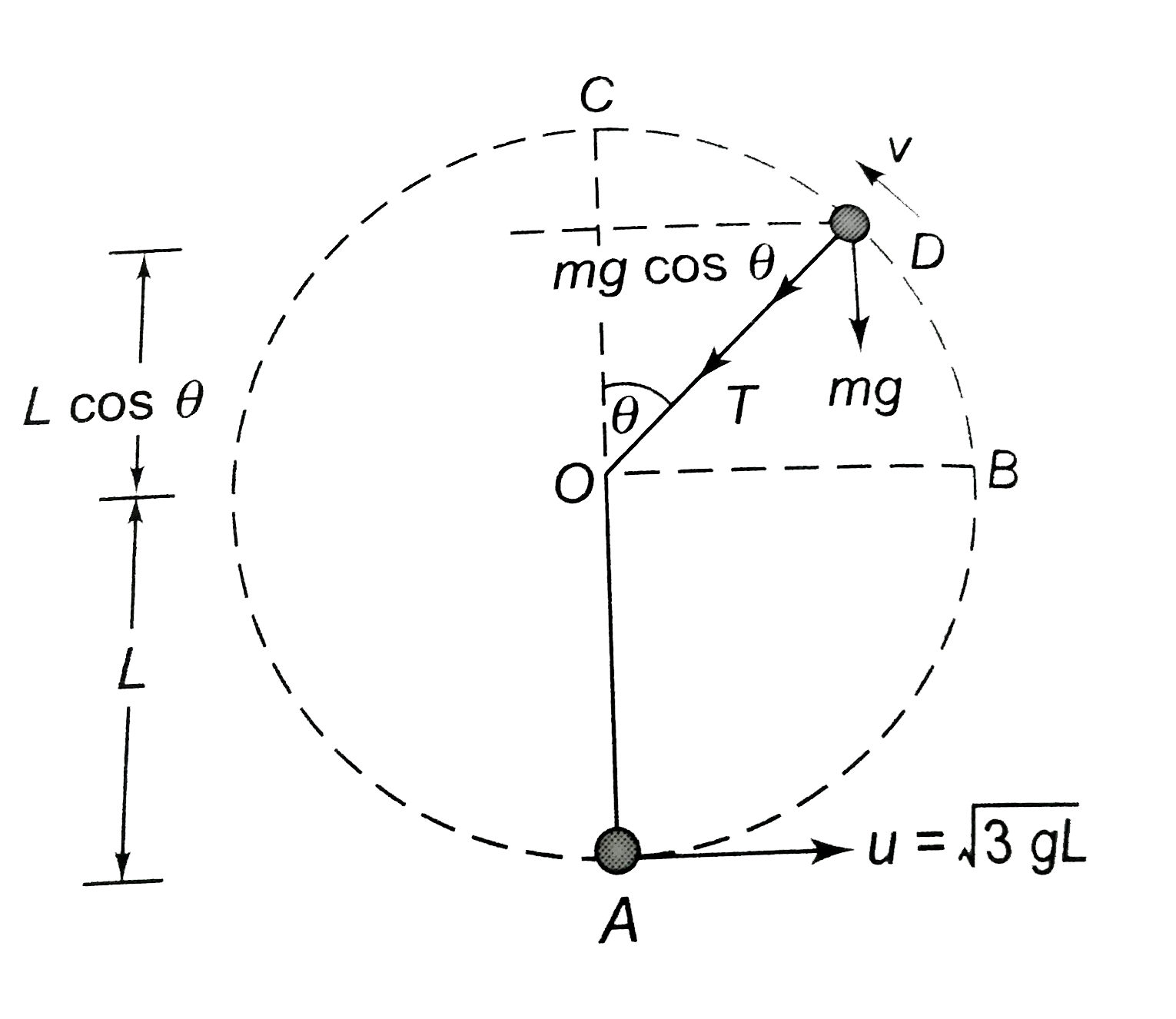
The bob of a simple pendulum is given a sharp hit impart it a horizontal speed of $\sqrt{3 g L}$ . Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. $L$ is length of the string.

Video Solution

Text Solution

Verified by Experts

Here velocity at the lowest point $μ = \sqrt{3 g L}$
$\sqrt{2 g L} < t - \sqrt{5 g L}$ , the tension in the sting will be zero before velocity and it will leave circular path between $B$ and $C$ and then it will move as a free particle on a parabolic path.
$v^{2} = u^{2} - 2 g h$
$v^{2} = u^{2} - 2 g L (1 + cos θ)$ .....(i)
$T + m g cos θ = \frac{m v^{2}}{L}$ .....(ii)
When the string becomes slack
$T = 0$ ....(iii)
Using (i),(ii)and (iii), we have
$g L cos θ = u^{2} - 2 g L (1 + cos θ)$
$= 3 g L - 2 g L (1 + cos θ)$
$cos θ = 3 - 2 - 2 cos θ$
$cos θ = \frac{1}{3} \Rightarrow θ = {cos}^{- 1} (\frac{1}{3})$
After $D$ , the particle moves on a parabolic path
$H_{max} = \frac{v^{2} {sin}^{2} θ}{2 g} = \frac{v^{2} (1 - {cos}^{2} θ)}{2 g}$
$= \frac{\frac{g L}{3} (1 - \frac{1}{9})}{2 g} = \frac{L}{6} \times \frac{8}{9} = \frac{4 L}{27}$
maximum height attained by the bob above the point of suspension
$O = L cos θ + H_{max} = \frac{L}{3} + \frac{4 L}{27} = \frac{13 L}{27}$

Updated on:21/07/2023