The bob of pendulum is project with horizontal velocity of √3gf I is the length of string. Find the angular displacement of string before it becomes slack.

π−cos−11/3
cos−12/3
π−cos−12/3
cos−11/3
The correct Answer is:A
Step by step video & image solution for The bob of pendulum is project with horizontal velocity of sqrt(3gf) I is the length of string. Find the angular displacement of string before it becomes slack. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
16 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-I)
3 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
The bobo of a pendulum at rest is given a sharp hit to impart a horizontal velocity u where l is the length of the pendulum. Find the angle rotated by the string before it becomes slack.
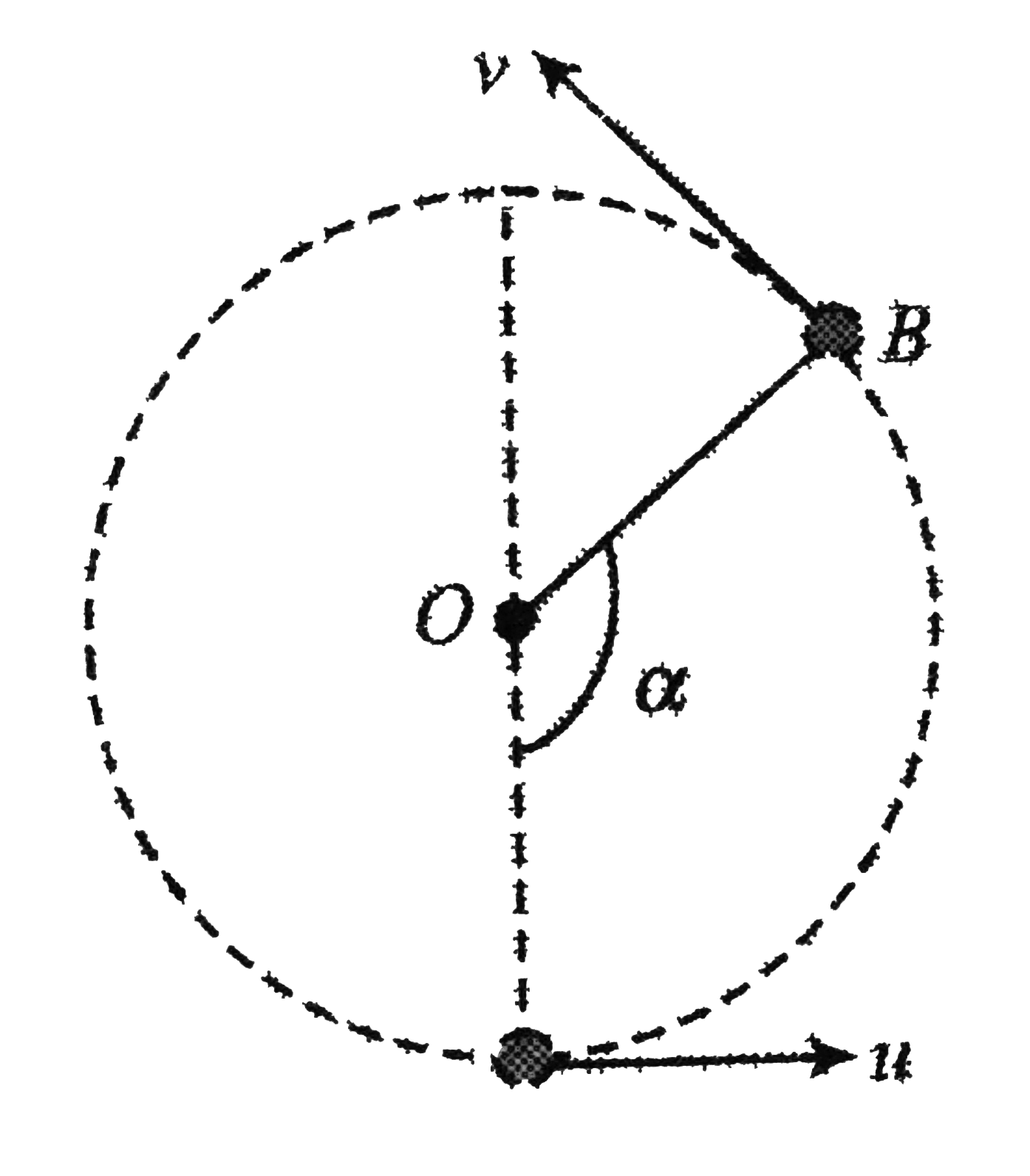
View SolutionThe bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of √3gl. Find the angle rotated by the string before it becomes slack.
View Solution
Knowledge Check
The period of the pendulum is directly proportional to the square root of the length of the string. The period of such a pendulum with string of length 16 cm is 52 seconds. Find the length of the string if the period is 65 seconds :
A(A) 4.5 cmB(B) 5 cmC(C) 6 cmD(D) none of theseThe period of the pendulum is directly proportional to the square root of the length of the string. The period of such a pendulum with string of length 16 cm is 52 seconds. Find the length of the string if the period is 65 seconds
A4.5 cmB5 cmC6 cmDNone of these
The bob of a pendulum at rest is given a sharp hit to impat a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with teh upward vertical.
View SolutionThe bob of a simple pendulum is given a sharp hit impart it a horizontal speed of √3gL. Find an angle made by the string with the upper verticle before it becomes slack. Also, calculate the maximum height attained by the bob above the point of suspension. L is length of the string.
View SolutionBob of a simple pendulum of length L is projected horizontally with a speed of u=√4gh, , from the lowest position. Find the distance of the bob from vertical line AB, at the moment its tangential acceleration becomes zero.
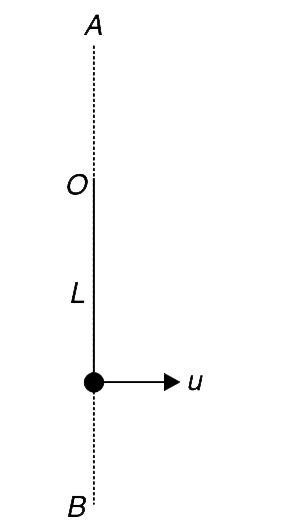
View SolutionA body is suspended by a string of length l is projected with horizontal velocity V such that it does not complete the motion in vertical circles.Then the height at which tension becomes is zero is given by?
View SolutionA heavy particle hangs from a point O, by a string of length a. It is projected horizontally with a velocity u=√(2+√3)ag . The angle with the downward vertical, string makes where string becomes slack is :
View SolutionA simple pendulum has a bob of mass m and string of length R. The bob is projected from lowest position giving it a horizontal velocity just enough for it to complete the vertical circle. Let the angular displacement of the pendulum from its initial vertical position be represented by θ. Plot the variation of kinetic energy (kE) of the bob and the tension (T) in the string with θ. Plot the graph for one complete rotation of the pendulum.
View Solution
