Theorem 8.10 : The line drawn through the mid-point of one side of a triangle, parallel to another side bisects the third side.
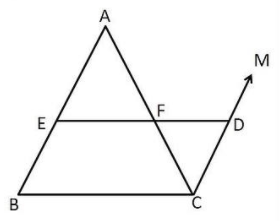
In quadrilateral EBCD
ED∣∣BC (given)
EB∣∣CD
Since both pairs of opposite sides are parallel, EBCDisparal≤logram
Since opposite sides of parallelogram are equal,
EB=DC
But, E is midpoint of AB,
EB=EA=DC
Now, EB∣∣DC with transversal ED
∠AEF=∠CDF (alternate angles)
In △AEF and △CDF
∠AFE=∠CFD (vertically opposite angles)
∠AEF=∠CDF
AE=DC
By AAS congruency, △AEF and △CDF are congruent.
Thus, AF=CF
F is midpoint of AC.
Topper's Solved these Questions
- BOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 9.11videos
NCERT THEOREMS
- BOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 9.21videos
NCERT THEOREMS
- BOOK - NCERTCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 8.91videos
NCERT THEOREMS
- BOOK - NCERTCHAPTER - LINES AND ANGLESEXERCISE - SOLVED EXAMPLES8videos
LINES AND ANGLES
- BOOK - NCERTCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.42videos
NUMBER SYSTEMS
Similar Questions
The line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
View SolutionUsing Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
 View Solution
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionThe line drawn through the mid-point of one side of a triangle is parallel to another side and intersects the third side at its mid-point.
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View Solutionprove that the line segment joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionThe line segment joining the mid-points of any two sides of a triangle is parallel to the third side of a triangle.
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View SolutionThe line segment joining the mid-points of any two sides of a triangle in parallel to the third side and equal to half of it.
View Solution

