Two point charges having charge +Q, –q and mass M, m respectively are separated by a distance L. They are released from rest in a uniform electric field E. The electric field is parallel to line joining both the charges and is directed from negative to positive charge. For the separation between particles to remain constant, the value of L is
$(K = \frac{1}{4 π \in_{0}})$

A

$\sqrt{\frac{(M + m) K Q q}{E (q M + Q m)}}$

B

$\sqrt{\frac{(M + m) K Q q}{E (q m + Q M)}}$

C

$\sqrt{\frac{m m K Q q}{E (q M + Q m)}}$

D

$\sqrt{\frac{m m K Q q}{E (Q M + q m)}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

In order to maintain constant separation the particles must have the same acceleration Assuming the system of both charges to acceleration towards left.Applying Newton's second law to the left particle we get

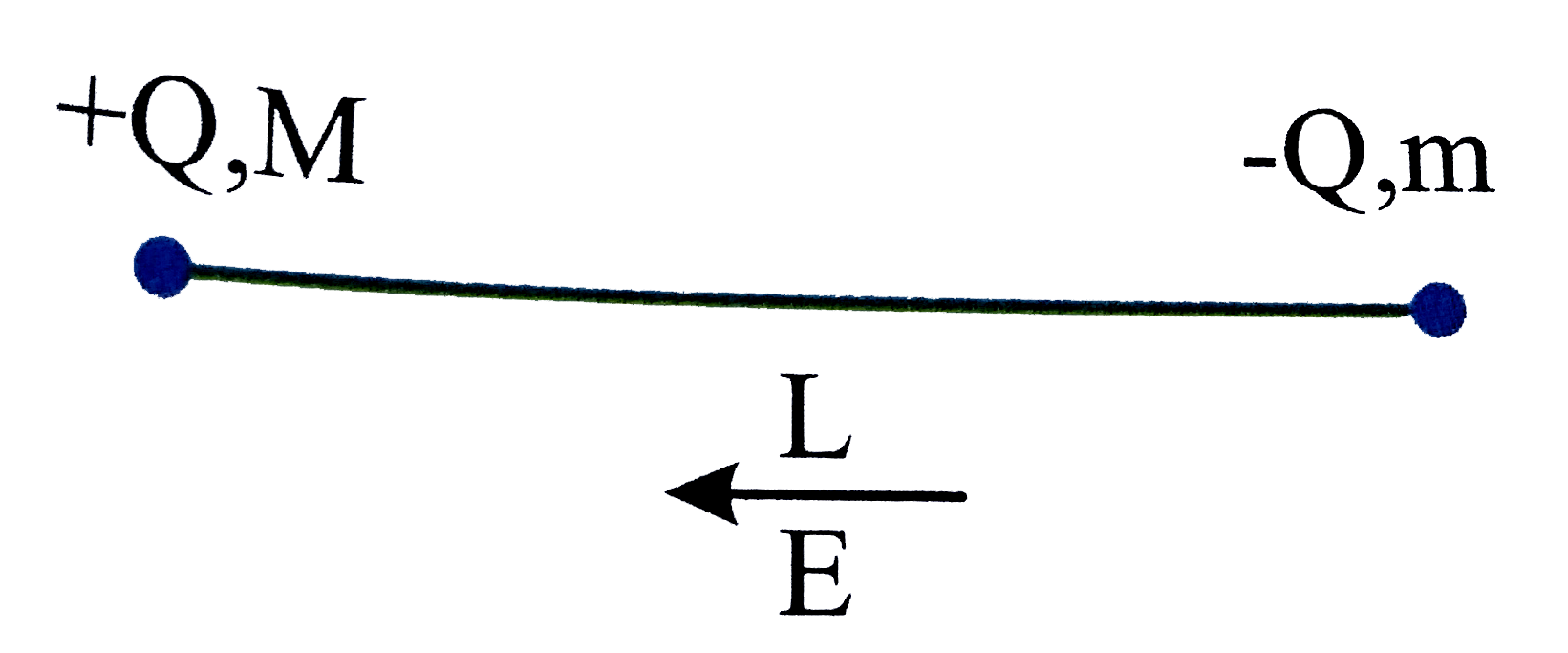
$Q E - \frac{K Q q}{L^{2}} = M a ... ... . (1)$
Under given condition the acceleration of both charges should be and should also be equal to acceleration of centre of mass of both the charges.
$a = \frac{F_{net}}{total mass} = \frac{(Q - q) E}{m + M} ... . . (2)$
$L = \frac{\sqrt{(M + m) K Q q}}{E (q M + Q m)}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge.
Aremains a constant because the electric field is uniform.
Bincrease because the charge moves along the electric field.
Cdecreases because the charge moves along the electric field.
Ddecreases because the charge moves opposite to the electric field.
Question 2 - Select One
A positively charged particle is released from rest in an uniform electric field. The electric potential energy of the charge
Aremains a constant because the electric field is uniform.
Bincreases because the charge moves along the electric field.
Cdecreases because the charge moves along the electric field.
Ddecreases because the charge moves opposite to the electric field.
Question 3 - Select One
A protons of mass $m$ charge $c$ is released form rest in a uniform electric field of strength $E$ .The time taken by it to travel a distance $d$ in the field is
A $\sqrt{\frac{2 d e}{m E}}$
B $\sqrt{\frac{2 d m}{E e}}$
C $\sqrt{\frac{2 d E}{m e}}$
D $\sqrt{\frac{2 d E e}{d m}}$

NARAYNA-ELECTROSTATICS AND GAUSS LAW-Level V

Home
Ask Doubt
Profile