In Fig. if ST∣∣QR . Find PS . (FIGURE)
To find PS in the given triangle where ST∥QR, we will use Thales' theorem, which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.
1. Identify the Given Information:
- We have two parallel lines: ST and QR.
- The segments on one side of the triangle are PT and TR, and we know their lengths.
- We need to find the length of segment PS.
2. Write Down the Known Lengths:
- From the problem, we know:
- PT=3cm
- TR=3cm
- QS=3cm
3. Apply Thales' Theorem:
- According to Thales' theorem, the following proportion holds:
PTTR=PSQS
4. Substitute the Known Values into the Proportion:
- Substitute the known lengths into the equation:
33=PS3
5. Simplify the Equation:
- The left side simplifies to 1:
1=PS3
6. Solve for PS:
- To find PS, multiply both sides by 3:
PS=3×1=3cm
Final Answer:
Thus, the length of PS is 3cm.
- We have two parallel lines: ST and QR.
- The segments on one side of the triangle are PT and TR, and we know their lengths.
- We need to find the length of segment PS.
- From the problem, we know:
- PT=3cm
- TR=3cm
- QS=3cm
- According to Thales' theorem, the following proportion holds:
PTTR=PSQS
- Substitute the known lengths into the equation:
33=PS3
- The left side simplifies to 1:
1=PS3
- To find PS, multiply both sides by 3:
PS=3×1=3cm
Thus, the length of PS is 3cm.
Topper's Solved these Questions
Similar Questions
In Fig. 4.230, each of PA, QB, RC and SD is perpendicular to l . If AB=6cm , BC=9cm , CD=12cm and PS=36cm , then determine PQ, QR and RS . (FIGURE)
View SolutionIn Fig. 4.238, S and T are points on the sides PQ and PR respectively of PQR such that PT=2cm , TR=4cm and ST is parallel to QR . Find the ratio of the areas of PST and PQR . (FIGURE)
View SolutionIn Fig. 4.90, if POS~ROQ , prove that PSQR . (FIGURE)
View SolutionIn Fig. 6.31, if PQ∣∣ST,∠PQR=110∘ and∠RST=130∘, find ∠QRS.
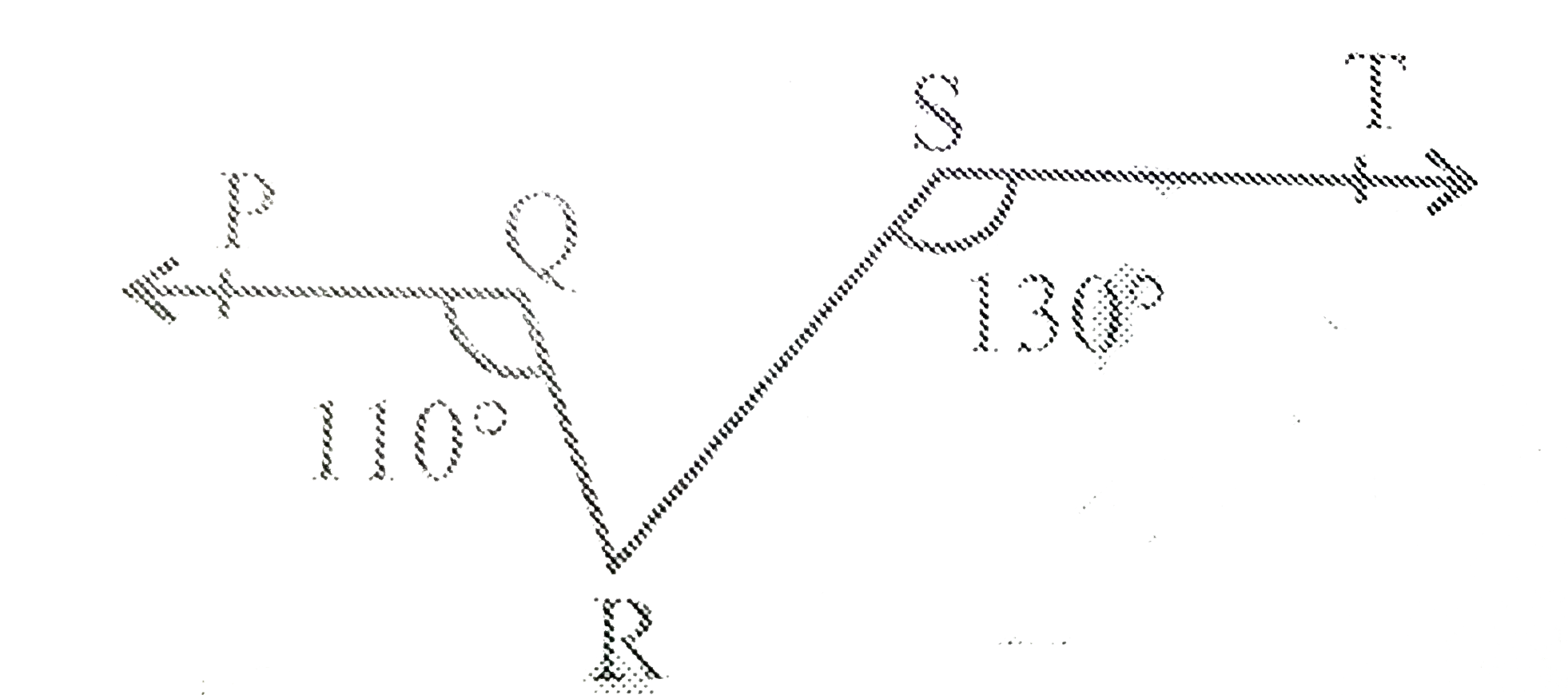
View SolutionIn Fig. 6.43, if PQ⊥PS,PQ∣∣SR,∠SQR=28∘ and ∠QRT=65∘, then find the values of x and y.

View SolutionIn Fig. 4.90, if PSQR , prove that POS~ROQ . (FIGURE)
View SolutionIn the given Fig. , if QTPR=QRQS and ∠1=∠2 . Prove that PQS~TQR . (FIGURE)
View SolutionIn Fig. 15.77, PQRS is a square of side 4 cm. Find the area of the shaded region. (FIGURE)
View SolutionIn Fig 7.51, PR > PQand PS bisects ∠QPR. Prove that ∠PSR >∠PSQ.

View SolutionIn Fig. 6.42, if lines PQ and RS intersect at point T, such that ∠PRT=40∘,∠RPT=95∘ and ∠TSQ=75∘, find ∠SQT.

View Solution