ABC is a triangle in which ∠B=2∠C.D is a point on BC such that AD bisects ∠BAC and AB=CD. Prove that ∠BAC=72∘
To solve the problem, we will follow these steps:
Step 1: Define the Angles
Let angle C = y. According to the problem, angle B = 2y. Therefore, we can express the angles in terms of y:
- Angle B = 2y
- Angle C = y
Step 2: Use the Angle Sum Property
In triangle ABC, the sum of the angles is 180 degrees. Therefore:
∠A+∠B+∠C=180∘
Substituting the values we have:
∠A+2y+y=180∘
This simplifies to:
∠A+3y=180∘
Thus, we can express angle A as:
∠A=180∘−3y
Step 3: Analyze the Angle Bisector
Since AD bisects angle BAC, we have:
∠BAD=∠CAD=12∠A=12(180∘−3y)=90∘−3y2
Step 4: Set Up Triangle Relationships
Since AB = CD, we can use the properties of triangles to analyze triangle ABD and triangle ACD. We know:
- AD is the angle bisector.
- AB = CD.
Step 5: Apply the Angle Bisector Theorem
By the angle bisector theorem, we have:
ABAC=BDDC
Since AB = CD, we can denote BD as x and DC as x (because D is a point on BC). Thus:
ABAC=xx=1
This implies that:
AB=AC
This means triangle ABC is isosceles with AB = AC.
Step 6: Set Up the Equation
Since triangle ABC is isosceles and we have:
∠B=2yand∠C=y
From the isosceles triangle property, we know:
∠A=180∘−3y
Setting up the equation:
2y=y(from the isosceles property)
Step 7: Solve for y
Now, we can substitute back into the equation:
180∘−3y+2y+y=180∘
This simplifies to:
180∘−3y+3y=180∘
This is always true, confirming our relationships.
Step 8: Find the Value of y
Now, we can find the value of angle BAC:
∠BAC=180∘−3y
We know that:
∠B=2yand∠C=y
So we can express:
∠BAC=180∘−3y=72∘
Thus:
3y=108∘⟹y=36∘
Finally, substituting back:
∠B=2y=72∘
Conclusion
We have proved that:
∠BAC=72∘
---
Let angle C = y. According to the problem, angle B = 2y. Therefore, we can express the angles in terms of y:
- Angle B = 2y
- Angle C = y
In triangle ABC, the sum of the angles is 180 degrees. Therefore:
∠A+∠B+∠C=180∘
Substituting the values we have:
∠A+2y+y=180∘
This simplifies to:
∠A+3y=180∘
Thus, we can express angle A as:
∠A=180∘−3y
Since AD bisects angle BAC, we have:
∠BAD=∠CAD=12∠A=12(180∘−3y)=90∘−3y2
Since AB = CD, we can use the properties of triangles to analyze triangle ABD and triangle ACD. We know:
- AD is the angle bisector.
- AB = CD.
By the angle bisector theorem, we have:
ABAC=BDDC
Since AB = CD, we can denote BD as x and DC as x (because D is a point on BC). Thus:
ABAC=xx=1
This implies that:
AB=AC
This means triangle ABC is isosceles with AB = AC.
Since triangle ABC is isosceles and we have:
∠B=2yand∠C=y
From the isosceles triangle property, we know:
∠A=180∘−3y
Setting up the equation:
2y=y(from the isosceles property)
Now, we can substitute back into the equation:
180∘−3y+2y+y=180∘
This simplifies to:
180∘−3y+3y=180∘
This is always true, confirming our relationships.
Now, we can find the value of angle BAC:
∠BAC=180∘−3y
We know that:
∠B=2yand∠C=y
So we can express:
∠BAC=180∘−3y=72∘
Thus:
3y=108∘⟹y=36∘
Finally, substituting back:
∠B=2y=72∘
We have proved that:
∠BAC=72∘
Topper's Solved these Questions
Similar Questions
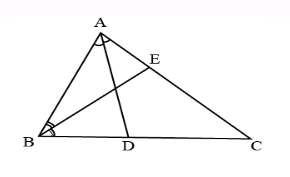
In Figure, ABC is a triangle in which ∠B=2∠C . D is a point on side BC such that AD bisects ∠BAC and AB=CD.BE is the bisector of ∠B . The measure of ∠BAC is
(a) 720
(b) 730
(c) 740
(d) 960
 View Solution
View SolutionABC is a triangle in which ∠B =2 ∠C.D is a point on BC such that AD bisects ∠BAC and AB=CD . Prove that ∠BAC=720.
View SolutionABC is a triangle. D is a point on AB such that D=14AB and E is a point on AC such that AE=14AC. Prove that DE=14BC
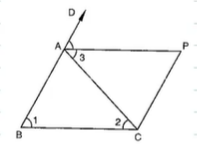
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
 View Solution
View SolutionABC is a triangle in which D is the mid-point of BC and E is the mid-point of AD. Prove that area of △BED=14areaof△ABC. GIVEN : A ABC,D is the mid-point of BC and E is the mid-point of the median AD. TO PROVE : ar(△BED)=14ar(△ABC).
View SolutionABCD is a parallelogram. E is a point on BA such that BE=2 EA and F is a point on DC such that DF=2 FC. Prove that AE CF is a parallelogram whose area is one third of the area of parallelogram AB CD.
View SolutionABCD is a parallelogram. P is a point on AD such that AP=13 AD and Q is a point on BC such that: CQ=13BC. Prove that AQCP is a parallelogram.
View SolutionABC is a triangle in which D is the mid-point of BC ,E andF are mid-points of DC and AE respectively. If area of ABC is 16cm2, find the area of DEF
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X Prove that AD=2AB
View SolutionLet ABC be an isosceles triangle in which AB=AC If D,E,F be the mid-points of the sides BC, CA and AB respectively, show that the segment AD and EF bisect each other at right angles.
View Solution

