In Figure, ABCD, ABFE and CDEF
are parallelograms. Prove that
ar(ADE)=ar(BCF)
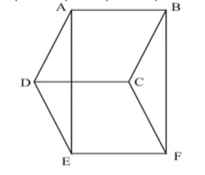
Step by step video & image solution for In Figure, A B C D ,\ A B F E\ a n d\ C D E F are parallelograms. Prove that a r( A D E)=a r( B C F) by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
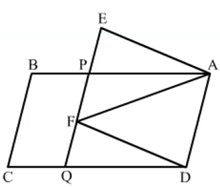
In Figure ABCDandAEFD are two parallelograms. Prove that: PE=FQ

View SolutionIn Figure, ABCDandAEFD are two parallelograms? Prove that: ar(APE):ar(PFA)=ar(QFD):ar(PFD)
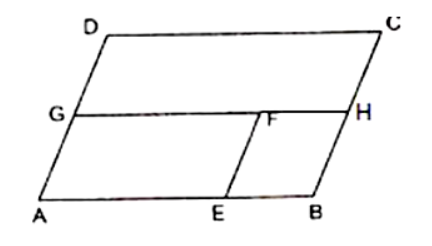
View SolutionIn Figure, ABCD and AEFG are two parallelograms. If ∠C=580, find ∠F
View SolutionIf Figure, CD AE and CYBA Name a triangle equal in area of CBX Prove that ar ( ZDE)= ar ( CZA) Prove that ar (BCZY)=ar ( EDZ)
View SolutionIn Figure, AD and BE are medians of ABC and BE∣∣DF . Prove that CF=14AC
Consider △ABC Since AD is Median
⇒BD=BC⋯(1)
Since BE is Median⇒AE=EC⋯(2)
Consider △BECSince BE∣∣DF
By Basic Proportionality theorm, ⇒BDDC=EFFC
From (1)⇒CF=EF
⇒CF=12AE
From (2)⇒CF=14AC
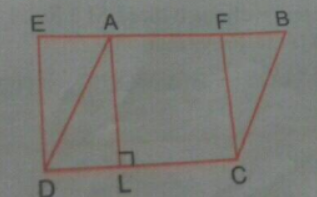
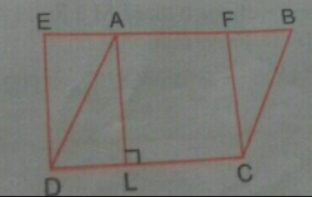
View SolutionIn Figure, ABCD is a parallelogram and EFCD is a rectangle. Also AL⊥DC. Prove that : ar(ABCD)=ar(EFCD) ar(ABCD)=DC×AL
View SolutionIn Fig. 71, if ABCD and CDEF, find ∠ACE
View SolutionIn the following figure, ABCD is a parallelogram and EFCD is a rectangle. Also, AL⊥DC. Prove that
(i) ar(ABCD)=ar(EFCD)
(ii) ar(ABCD)=DC×AL.
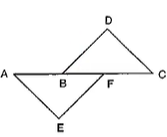
View SolutionIn Figure, it is given that AB=CF, EF=BD and ∠AFE= ∠CBD. Prove that AFE= ≅CBD
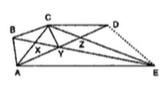
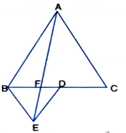
View SolutionIn Figure, ABC and BDE are two equilateral triangls such that D is the mid-point of BC.AE intersects BC in F. Prove that: ar ( BFE)= 2 ar (EFD)
View Solution
