In Figure ABCD is a cyclic quadrilateral. If ∠BCD=1000and ∠ABD=700, find ∠ADB
To solve the problem, we will use the properties of cyclic quadrilaterals and the angles involved. Let's go through the solution step by step.
Step 1: Identify the angles in the cyclic quadrilateral
We have a cyclic quadrilateral ABCD where:
- ∠BCD=100∘
- ∠ABD=70∘
Step 2: Use the property of cyclic quadrilaterals
In a cyclic quadrilateral, the sum of the opposite angles is 180∘. Therefore, we can find ∠ABC using:
∠ABC+∠BCD=180∘
Substituting the known value:
∠ABC+100∘=180∘
∠ABC=180∘−100∘=80∘
Step 3: Analyze triangle ABD
Now, we will consider triangle ABD. The angles in a triangle sum up to 180∘. Therefore, we can write:
∠ABD+∠ADB+∠DAB=180∘
Substituting the known values:
70∘+∠ADB+80∘=180∘
Step 4: Solve for ∠ADB
Now, we can simplify the equation:
70∘+80∘+∠ADB=180∘
150∘+∠ADB=180∘
Subtracting 150∘ from both sides gives:
∠ADB=180∘−150∘=30∘
Conclusion
Thus, the measure of ∠ADB is 30∘.
Final Answer
∠ADB=30∘
---
We have a cyclic quadrilateral ABCD where:
- ∠BCD=100∘
- ∠ABD=70∘
In a cyclic quadrilateral, the sum of the opposite angles is 180∘. Therefore, we can find ∠ABC using:
∠ABC+∠BCD=180∘
Substituting the known value:
∠ABC+100∘=180∘
∠ABC=180∘−100∘=80∘
Now, we will consider triangle ABD. The angles in a triangle sum up to 180∘. Therefore, we can write:
∠ABD+∠ADB+∠DAB=180∘
Substituting the known values:
70∘+∠ADB+80∘=180∘
Now, we can simplify the equation:
70∘+80∘+∠ADB=180∘
150∘+∠ADB=180∘
Subtracting 150∘ from both sides gives:
∠ADB=180∘−150∘=30∘
Thus, the measure of ∠ADB is 30∘.
∠ADB=30∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
In a cyclic quadrilateral ABCD∠A+∠B+∠C+∠D=?
AA)90∘BB)360∘CC)180∘DD)120∘If ABCD is a cyclic quadrilateral with ∠A=50∘,∠B=80∘, then Cand∠D are
A100∘,130∘B115∘,115∘C110∘,120∘D130∘,100∘
ABCD is a cyclic quadrilateral such that ∠ADB=300and ∠DCA=800, the ∠DAB= 700 (b) 1000 (c) 1250 (d) 1500
View SolutionIn a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC=300, ∠BDC=100and ∠CAB=700, Find: ∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB
Given that
∠ABC=302,∠ABC=∠ADC=300
[We know that measure of opposite angles are equal in a parallelogram]
∠BDC=100
∠CAB=700
∠BDA=∠ADB=∠ADC–∠BDC
302–102=202
∠DAB=1800–300=1500
∠ADB=∠DBC=200 (alternate angles)
∠BCD=∠DAB=1500
[we know, opposite angles are equal in a parallelogram]
∠DBA=∠BDC=100
[we know, Alternate interior angles are equal]
InΔABC
∠CAB+∠ABC+∠BCA=1800
[since, sum of all angles of a triangle is 180^0]
700+300+∠BCA=1800
∠BCA=1800–1000=800
∠DAB=∠DAC+∠CAB=700+800=1500
∠BCD=1500 (opposite angle of the parallelogram)
∠DCA=∠CAB=700
In ΔDOC∠BDC+∠ACD+∠DOC=1800
[since, sum of all angles of a triangle is 180^0]
100+700+∠DOC=1800
∠DOC=1800−800
∠ACB=800
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
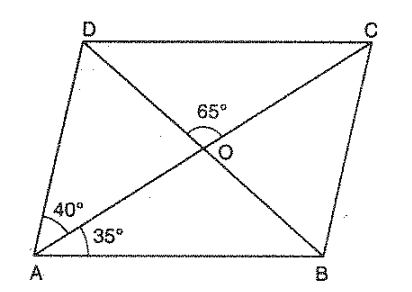
View SolutionIn Figure, AB is a diameter of the circle such that ∠A=350and ∠Q=250, find ∠PBR
View SolutionIn Figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD=1460, find ∠AOB
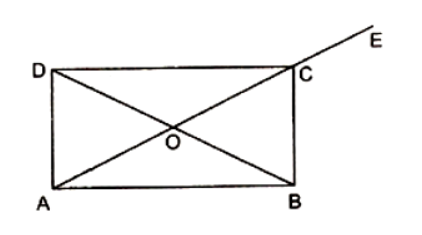
View SolutionABCD is a cyclic quadrilateral whose diagonals AC and BD intersect at P. If AB=DC, prove that:
(i)PAB ≅PDC
(ii)PA=PD and PC=PB (iii) AD∣∣BC
View Solution
