A ring attached with a spring is fitted in a smooth rod. The spring is fixed at the outer end of the ord. The mass of the ring is 3kg& spring constant is 300N/m. The ring is given a velcoity 'V' towards the outer end of the rod. And the rod is set to be rotating with an angular velcity ω. Then ring will move with constant speed with respect to the rod if :
 Natural length of spring is same as that of length of rod.
Natural length of spring is same as that of length of rod.
angular velocity of rod is increased continuously.
ω=10rad/s
angular velocity of rod is decreased continuously.
constant velocity of ring is not possible.
The correct Answer is:B
For the ring to move in a circle at constant speed the net force on it should be zero. Here spring force will provide the necessar centripetal force.
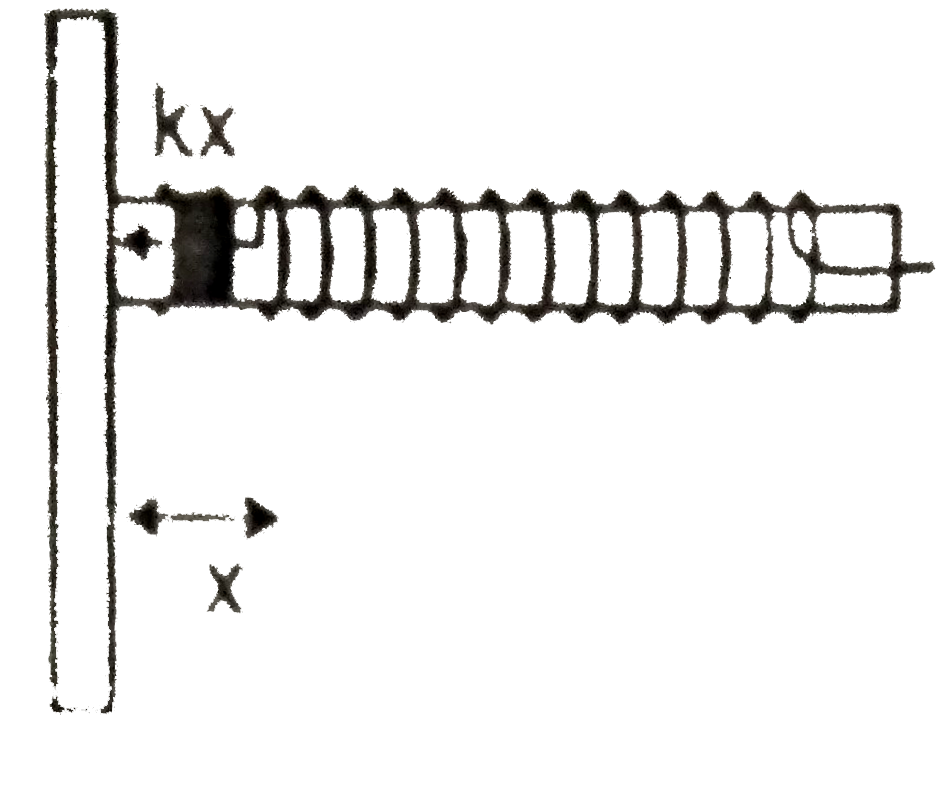
∴Kx=mxω2
⇒ω=√km=√3003=10rad/sec.Ans
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 484videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 496videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 466videos
DAILY PRACTICE PROBLEMS
- BOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise54videos
CURRENT ELECTRICITY
- BOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems9videos
ELASTICITY AND VISCOCITY
Similar Questions
Knowledge Check
- Question 1 - Select One
A particle of mass m is fixed to one end of a massless spring of spring constant k and natural length l0. The system is rotated about the other end of the spring with an angular velocity ω ub gravity-free space. The final length of spring is
Amω2l0kBmω2l0k−mω2Ckl0k−mω2Dmω2l0k+mω2 - Question 2 - Select One
A thin circular ring of mass m and radius R is rotating about its axis perpendicular to the plane of the ring with a constant angular velocity ω. Two point particleseach of mass M are attached gently to the opposite end of a diameter of the ring. The ring now rotates, with an angular velocityω2. Then the ratiomM is
A1B2C12D√2 - Question 3 - Select One or More
A rod OA of length l is rotating (about end O) over a conducting ring in crossed magnetic field B with constant angular velocity ω as shown in figure.
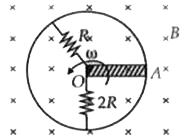 ACurrent flowing through the rod is 3Bωl24RBMagnetic force acting on the rod is 3B2ωl34RCTorque due to magnetic force acting on the rod is 3B2ωl48RDMagnitude of external force that acts perpendicularly at the end of the rod of maintain the constant angular speed is 3B2ωl38R
ACurrent flowing through the rod is 3Bωl24RBMagnetic force acting on the rod is 3B2ωl34RCTorque due to magnetic force acting on the rod is 3B2ωl48RDMagnitude of external force that acts perpendicularly at the end of the rod of maintain the constant angular speed is 3B2ωl38R

A ring of mass m is attached to a horizontal spring of spring constant k and natural length l0. The other end of the spring is fixed and the ring can slide on a horizontal rod as shown. Now the ring is shifted to position B and released Find the speed of the ring when the spring attains its natural length.View SolutionOne end of a spring is fixed at point O of a smooth fixed horizontal circular ring of rdius R=2m. A bead having mass 0.3 kg is pivoted on the ring and the other end of spring is connected to it. The natural length of the spring is R and spring constant is k=10N/m.
AB=6R5 and line OB is the diameter of the ring. If the normal reaction on bead due to the ring ag A is 0, what is the speed of the bead at A.
 View Solution
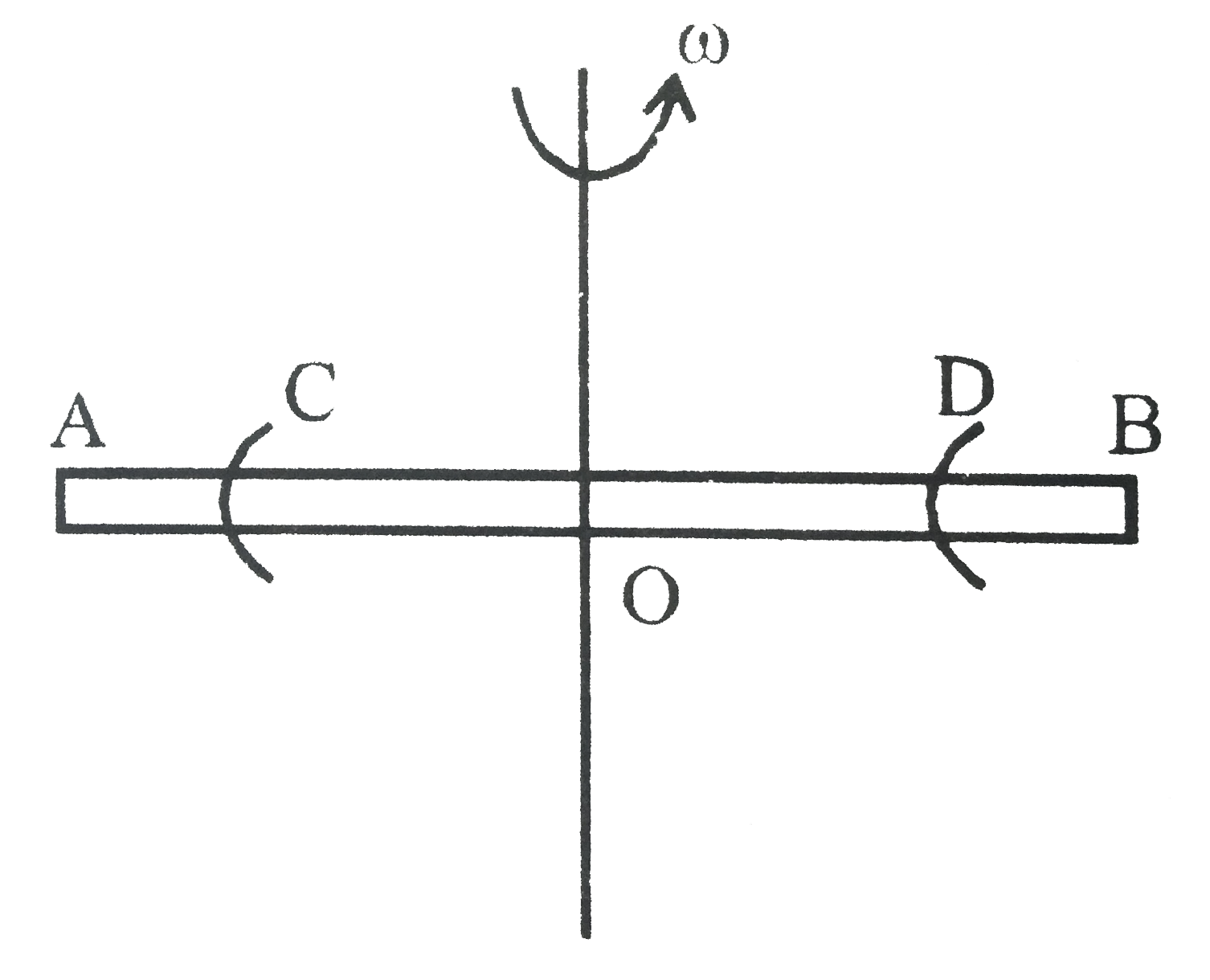
View SolutionA rigid horizontal smooth rod AB of mass 0.75kg and length 40cm can roate freely about a fixed vertical axis through its mid point O. Two rings each of mass 1kg are initially at rest at a distance of 10cm from O on either side of the rod. The rod is set in rotatiob with an angular velocity of 30 radians per second. Find the velocity of each ring along the length of the rod in m/s when they reach the ends of the rod.
 .View Solution
.View SolutionA rod AB of mass m and length L is rotating in horizontal plane about one of its ends which is pivoted. The constant angular speed with which the rod is rotating is ω. The force applied on the rod by the pivot is
View SolutionThe figure shows mass m moves with velocity u. Find the velocity of ring at that moment. Ring is restricted to move on smooth rod.
 View Solution
View Solution

