The circular vertical section of the fixed track shown is smooth with radius $r = 0.9 c m$ and the horizontal straight section is rough with $μ = 0.1$ . A block of mass $1 k g$ is placed at point $' Q'$ and given a horizontal velocity of $\sqrt{3} m / s$ towards the spring. Distance $Q S = 40 c m$ and maximum compression in the spring is $10 c m$ during the motion $(g = 10 m / s^{2}) :$

A

The force constant of the spring is $200 N / m$

B

The velcoity with which block returns to point $' Q'$ is $1 m / s$

C

At point $P$ its velocity will be $0.8 m / s$

D

At point $P$ , the normal reaction on the block is less than $55 N$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C

$Q \to S$
$- μ m g \times S = 0 = 0 + \frac{1}{2} k {(0.1)}^{2} - \frac{1}{2} \times 1 \times {(\sqrt{3})}^{2}$
$- 0.1 \times 1 \times 10 \times 0.5 = \frac{k}{200} - \frac{3}{2}$
$k = 200 N / m$
$Q \to S \to Q$
$- μ m g \times 0.5 \times 2 = \frac{1}{2} m v_{Q}^{2} - \frac{1}{2} m u^{2}$
$- 1 = \frac{1}{2} \times 1 \times v_{Q}^{2} - \frac{1}{2} \times 1 \times 3$
$v_{Q} = 1 m / s$
$v_{P}^{2} = v_{Q}^{2} - 2 (g) (2 r)$
$v_{P} = \sqrt{1^{2} - 4 \times 10 \times \frac{0.9}{100}} = \frac{8}{10} = 0.8 m / s$
$N_{P} = \frac{m v_{P}^{2}}{r} - m g = \frac{1 \times 64 \times 100}{100 \times 0.9} - 10 = \frac{55}{0.9} N$ .

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

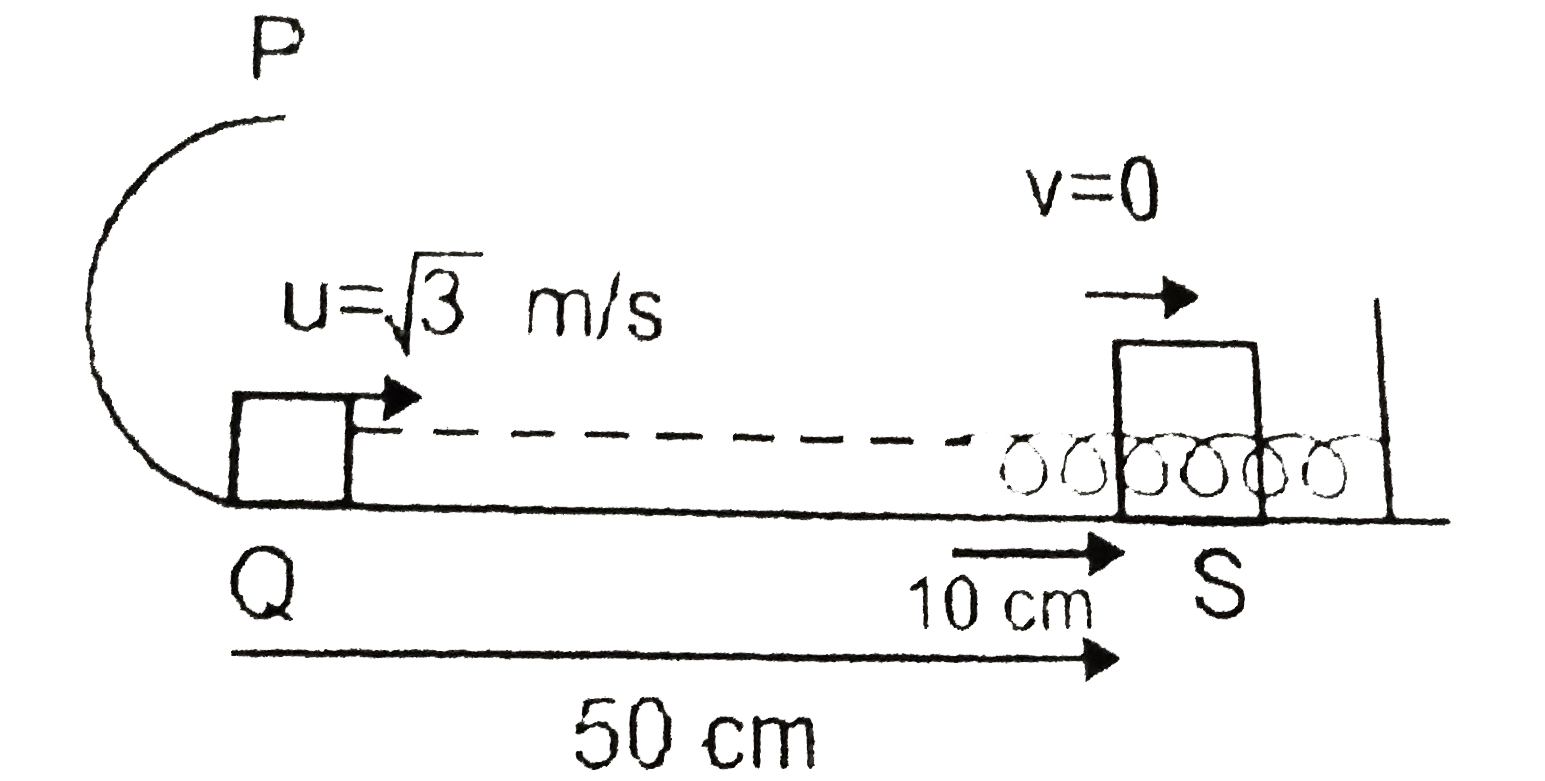
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A block of mass m moving with velocity $v_{0}$ on a smooth horizontal surface hits the spring of constant k as shown. Two maximum compression in spring is

A $\sqrt{\frac{2 m}{k}} v_{0}$
B $\sqrt{\frac{m}{k}} . v_{0}$
C $\sqrt{\frac{m}{k}} . v_{0}$
D $\frac{m}{2 k} . v_{0}$
Question 2 - Select One
Two blocks of masses $1 k g$ and $3 k g$ are moving with velocities $2 m / s$ and $1 m / s$ , respectively , as shown. If the spring constant is $75 N / m$ , the maximum compression of the spring is

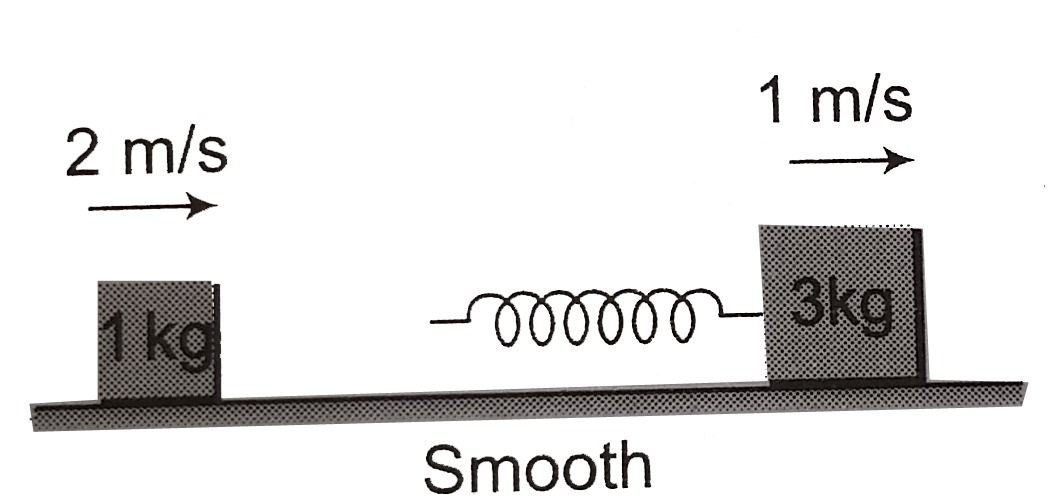A $5 c m$
B $2 c m$
C $3 c m$
D $4 c m$
Question 3 - Select One
A block of 10 g slides on smooth horizontal surface with 20 m/s towards a spring of spring constant 100 N/m placed horizontally (as shown in figure). The maximum compression in spring is

A2 m
B0.2 m
C20 m
D $\frac{4}{3} m$

RESONANCE-DAILY PRACTICE PROBLEMS-dpp 53

Home
Ask Doubt
Profile