The small particle of mass m is given an initial high velocity in the horizontal plane and winds its cord around the fixed vertical shaft of radius 1m. All motion occurs essentially in the horizontal plane. If the angular velocity of the cord is 0.8 rad/s when the distance from the particle to the tangency point is 5m, determine the angular velocity ω (in rad//s) of the cord after it has turned through an angle 1 rad.

The correct Answer is:1
ω=ω01−aθ/r∘,T=mr∘ω∘θ
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
A uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?
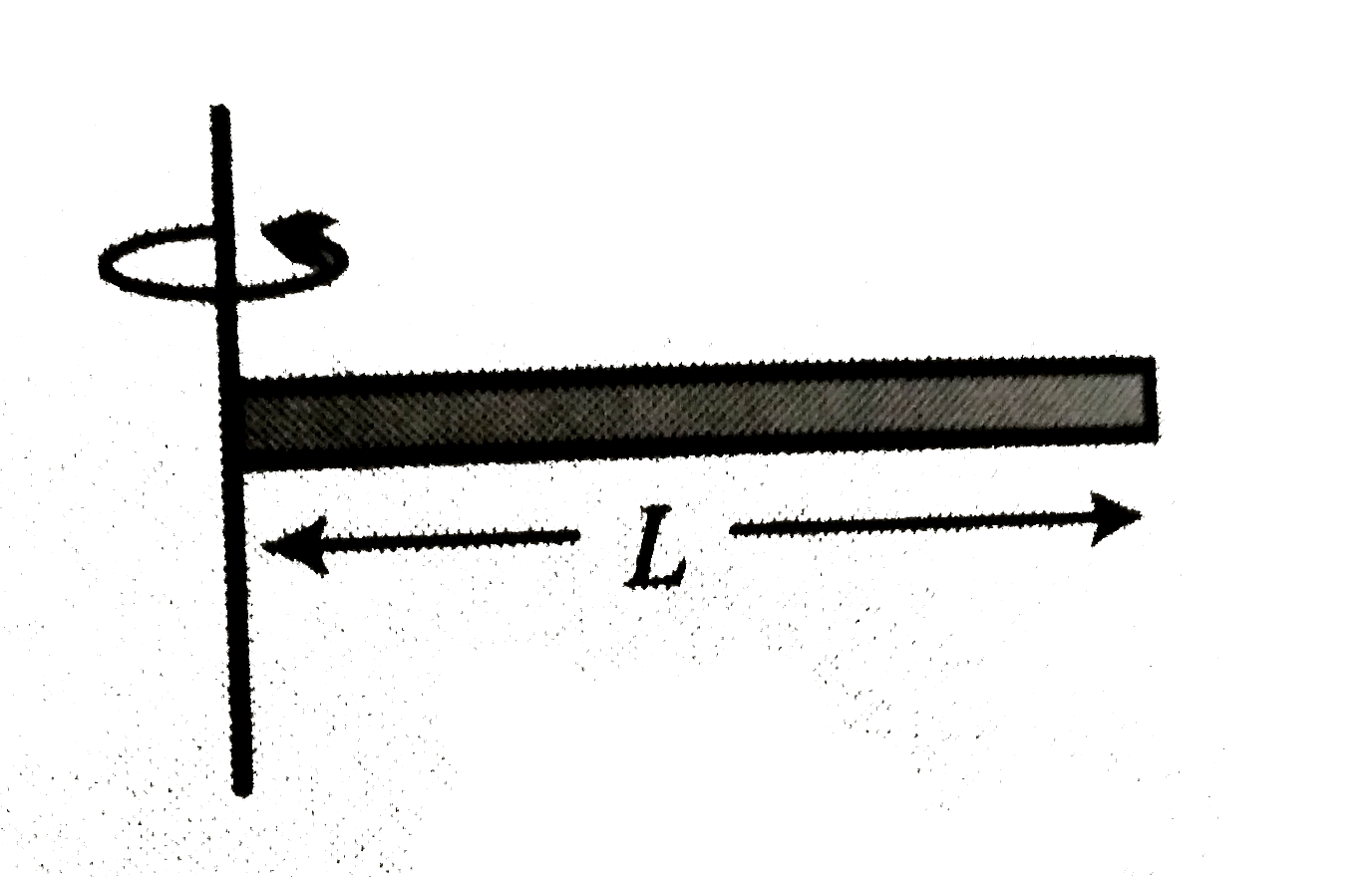
View SolutionA uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?

View Solution
Knowledge Check
A small particle of mass m is given an initial high velocity in the horizontal plane and winds its cord around the fixed vertical shaft of radius a. All motion occurs essentially in horizontal plane. If the angular velocity of the cord is when the distance from the particle to the tangency point is r0, then the angular velocity of the cord after it has turned through an angle is.
 Aω=ω0Bω=ar0ω0Cω=ω01−ar0θDω=ω0θ
Aω=ω0Bω=ar0ω0Cω=ω01−ar0θDω=ω0θA particle is in circular motion in a horizotantal plane. It has angular velocity of 10πrad/s at the end of 2 s and angular velocity 15 πrad/s at the end of 4s. The angular acceleration of particle is
A5πrad/s2B2.5πrad/s2C6πrad/s2D16πrad/s2The angular momentum of a body with mass (m) moment of inertia (I) and angular velocity (ω) rad/s is equal to
AIωBIω2CIωD1ω2
A disc of radius 10cm is moving with its centre's velocity =1m/s rightwards,on a flat horizontal surface.At a certain moment,its angular velocity is, 20rad/s ,anticlockwise.Then, the distance of a point on the disc (from its centre) whose instantaneous velocity is zero is
View SolutionA mass of 2 kg is rotating on a circular path of radius 0.8 m with angular velocity of 44rads−1. If the radius of the path becauses 1.0 m, what will be the value of angular velocity?
View SolutionThe angular momentum of a body with mass (m) moment of inertia (I) and angular velocity (ω) rad/s is equal to
View SolutionA particle of mass 2 kg is on a smooth horizontal table and moves in a circular path of radius 0.6 m. The height of the table from the ground is 0.8 m. If the angular speed of the particle is 12 rad s−1 , the magnitude of its angular momentum about a point on the ground right under the centre of the circle is
View SolutionA ring of radius 0.5 m and mass 10 kg is rotating about its diameter with angular velocity of 20 rad/s. Its KE is :-
View Solution
