Column 1: real valued function, Column-2: domain of the function, Column 3: range of the function. Match the following Column(s) (where [.] denotes the greatest integer function and {.} denotes fractional part function)
$Column 1: real valued function, Column-2: domain of the function, Column 3: range of the function. Match the following Column(s) (where [.] denotes the greatest integer function and {.} denotes fractional part function) Which of the following combination is correct?$
Which of the following combination is correct?

A

(IV)(i)(P)

B

(III)(ii)(S)

C

(IV)(i)(Q)

D

(II)(iii)(S)

Text Solution

Verified by Experts

The correct Answer is:C

(I) $\frac{{(3 - 1)}^{3}}{3} \leq {| x - 1 |}^{3} + {| x - 3 |}^{3} < \infty$
(II) $u = \sin (I n (\frac{\sqrt{4 - x^{2}}}{1 - x})) + \cos (I n (\frac{\sqrt{4 - x^{2}}}{1 - x}))$
$∴ 0 \leq \frac{\sqrt{4 - x^{2}}}{1 - x} < \infty$
$- \infty < I n (\frac{\sqrt{4 - x^{2}}}{1 - x}) < \infty$
So, $- \sqrt{2} \leq u \leq \sqrt{2}$
(III) Let $| x + 5 | = t, g (t) \in (0, \frac{1}{2}] - {\frac{1}{4}}$
$g (t) = \frac{(t - 2)}{(t - 2) (t + 2)}$
(IV) $θ \leq \cos^{- 1} x \leq π$
$- \infty < I n (\cos^{- 1} x) \leq I n π < 2$

Updated on:21/07/2023

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Column -1 : real valued function, Column -2: continuity of the function, Column - 3: differentiability of the function, Match the following Column(s)

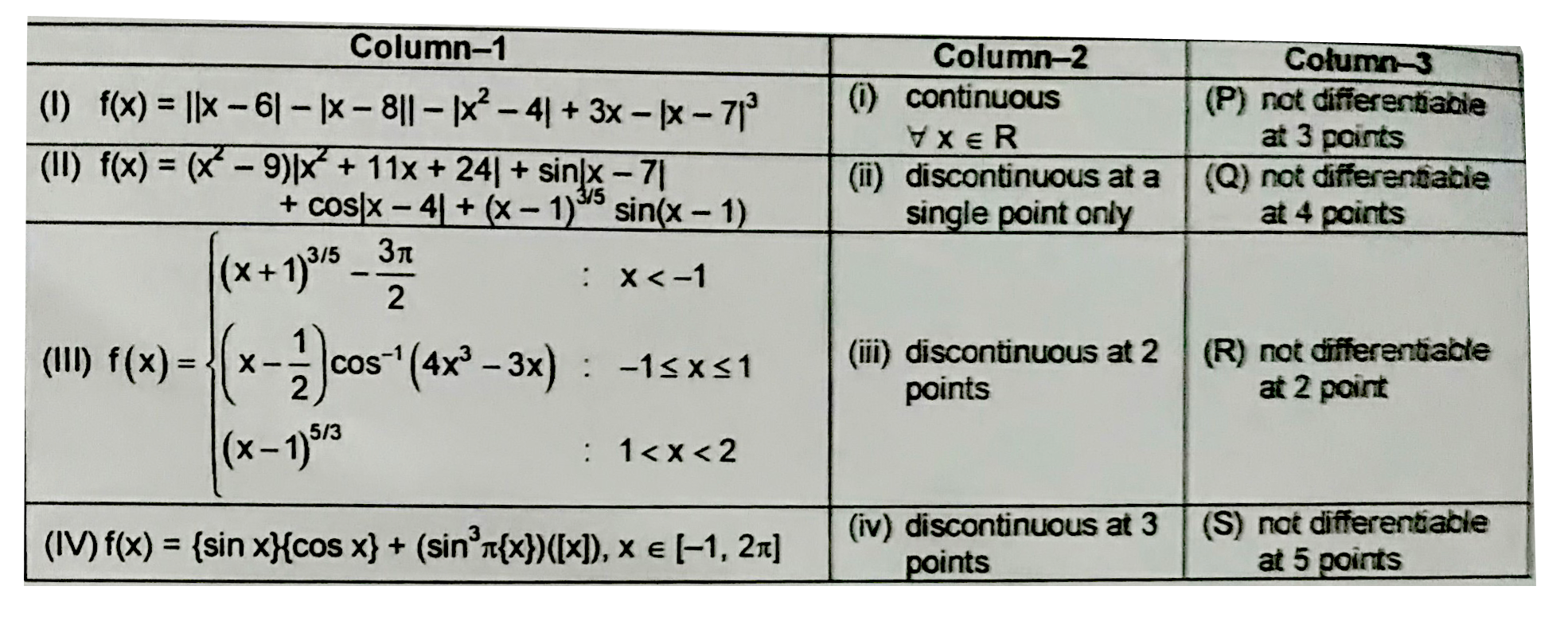
(Whre [.] denotes the greatest integer function and {.} fractional at function)
Which of the following combination combination is correct?
A(II)(iii)(S)
B(III)(I)(P)
C(II)(ii) $(R)$
D(III)(i) $(R)$
Column -1 : real valued function, Column -2: continuity of the function, Column - 3: differentiability of the function, Match the following Column(s)

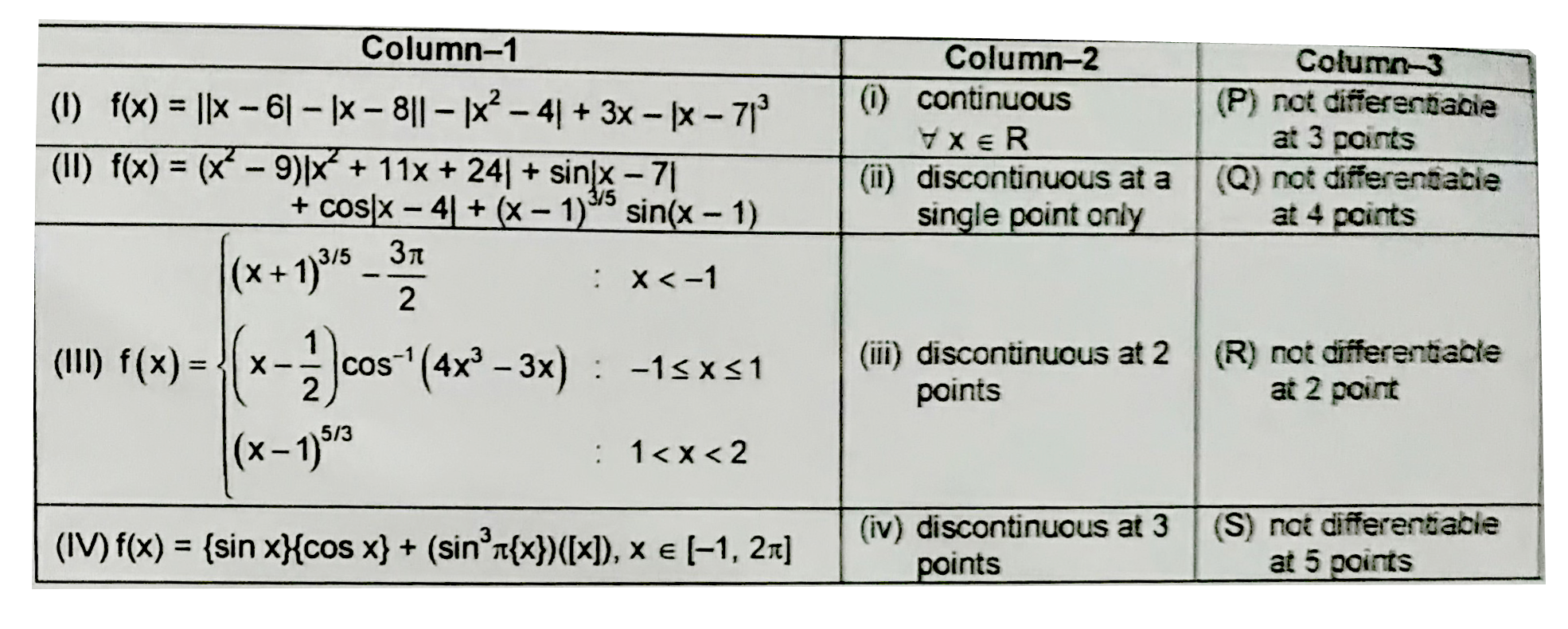
(Whre [.] denotes the greatest integer function and {.} fractional at function)
Which of the following combination combination is correct?
A(I)(i) $(R)$
B(III)(ii) $R$
C(IV)(iv)(P)
D(I)(i)(Q)
Column -1 : real valued function, Column -2: continuity of the function, Column - 3: differentiability of the function, Match the following Column(s)

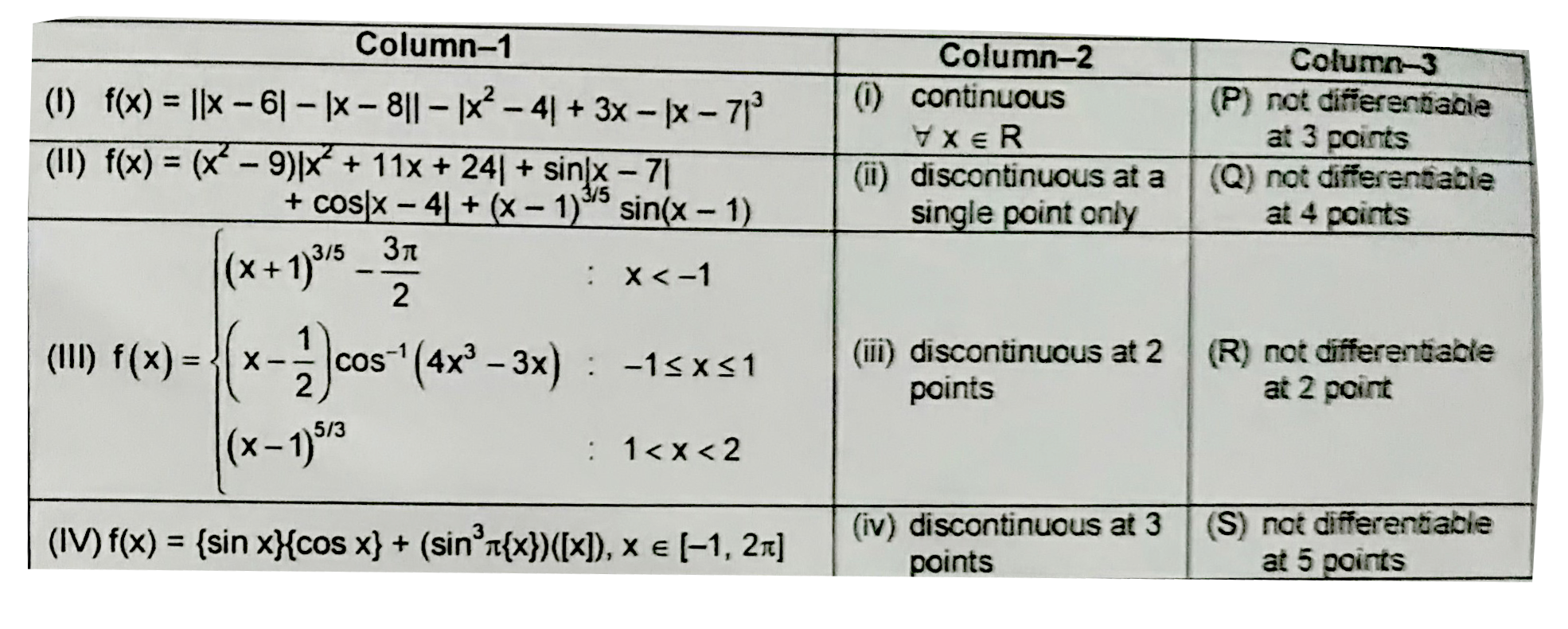
(Whre [.] denotes the greatest integer function and {.} fractional at function)
Which of the following is correct?
A(I)(i)(S)
B(IV)(i)(S)
C(II)(ii)(S)
D(II)(ii)(P)

FIITJEE-TEST PAPERS-MATHEMATICS

Home
Ask Doubt
Profile