A uniform thin rod AB of mass M and length l attached to a string OA of length l2 is placed on a smooth horizontal plane and rotates with angular velocity omega around a vertical axis through O. A peg P is inserted in the plane in order that on striking it the bar will come exactly to rest

Location of peg for rod coming to rest is r=5l6
Location of peg for rod coming to rest is r=3l4
Location of peg for rod coming to rest is r=1312
Location of peg for rod coming to rest is r=2l3
The correct Answer is:C
MVcmL2+Icmω
MωL2+ML212ω
I0=1312ML2ω
By impulse momentum theorem
∫Ndt=mV
By angular Impulse
r∫Ndt=mVl+Ml212VL
rMV=1312MVL
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - PHYSICS
18 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHS
18 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 physics
63 Videos
- TEST PAPER 4BOOK - ALLENCHAPTER - TEST PAPER 4EXERCISE - PHYSICS
45 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
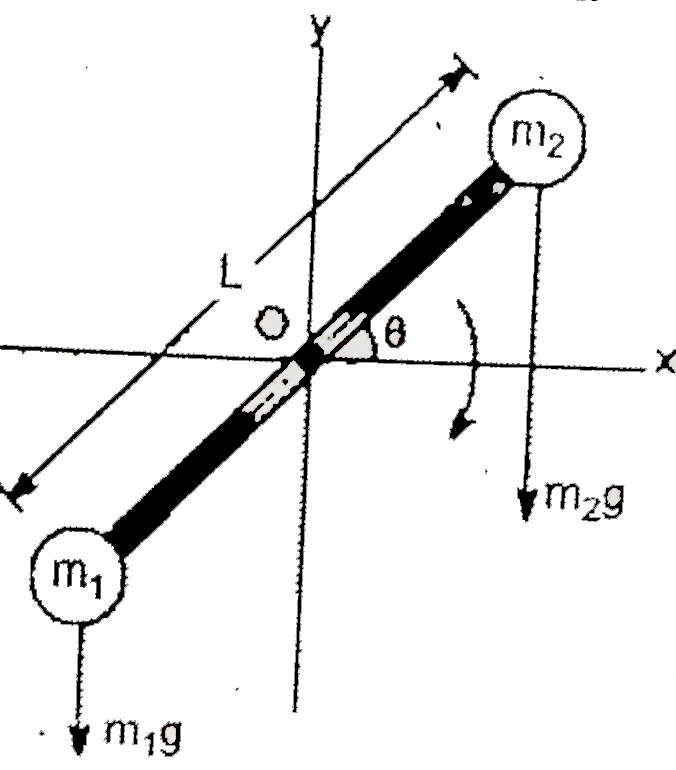
A uniform rod of mass M and length L with two particles m and m2 attached to its ends, is pivoted at its centre. The system rotates in a vertical plane with angular velocity ω.
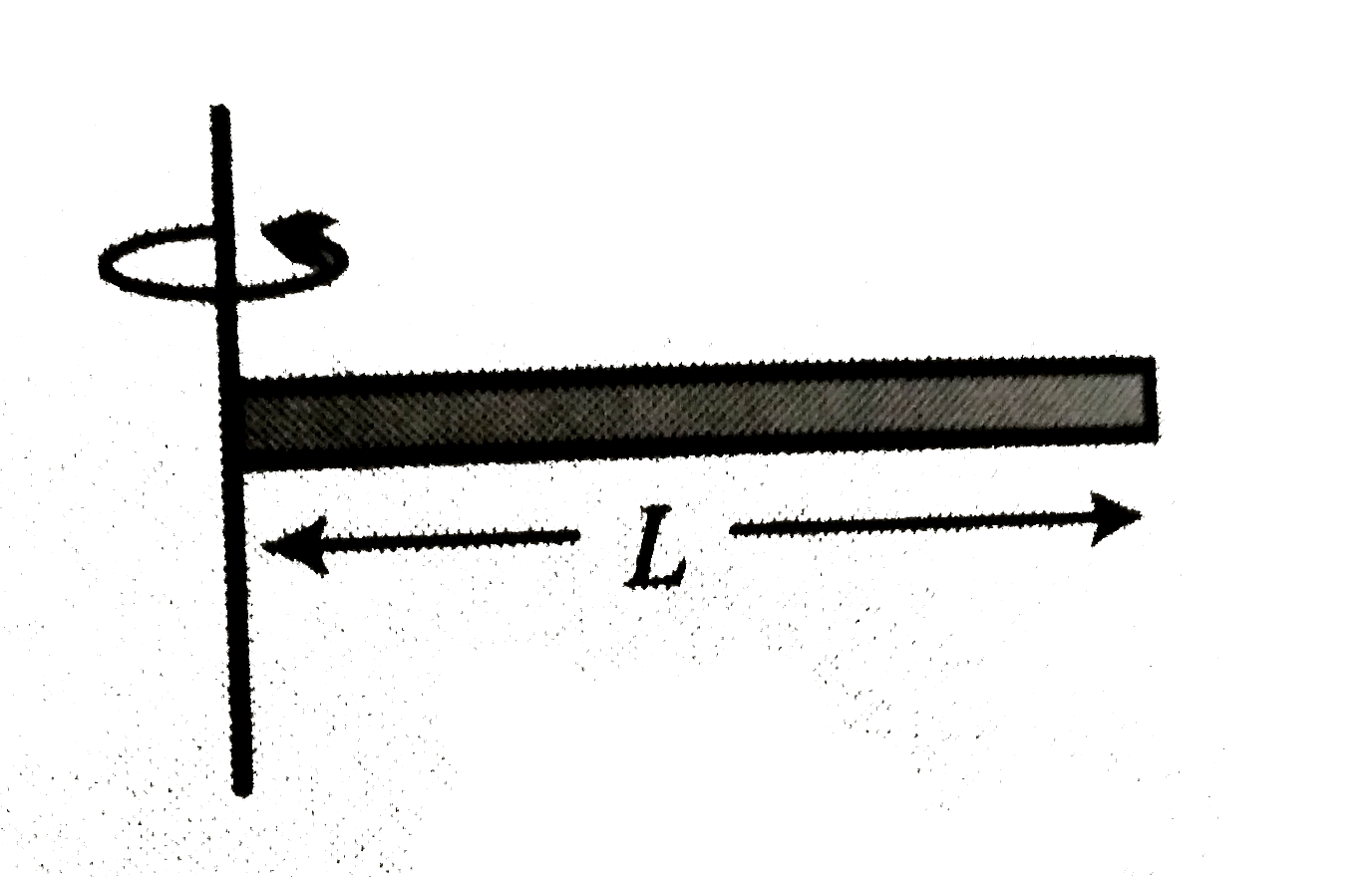
View SolutionA uniform of mass m and length L is tied to a vertical shaft. It rotates in horizontal plane about the vertical axis at angular velocity ω. How much horizontal force does the shaft exert on the rod?
View Solution
Knowledge Check
A uniform thin rod AB of mass M and length l attached to a string OA of length =12 is supported by a smooth horizontal plane and rotates with angular velocity ω around a vertical axis through O. A peg P is inserted in the plane in order that on striking it the rod will come to rest.
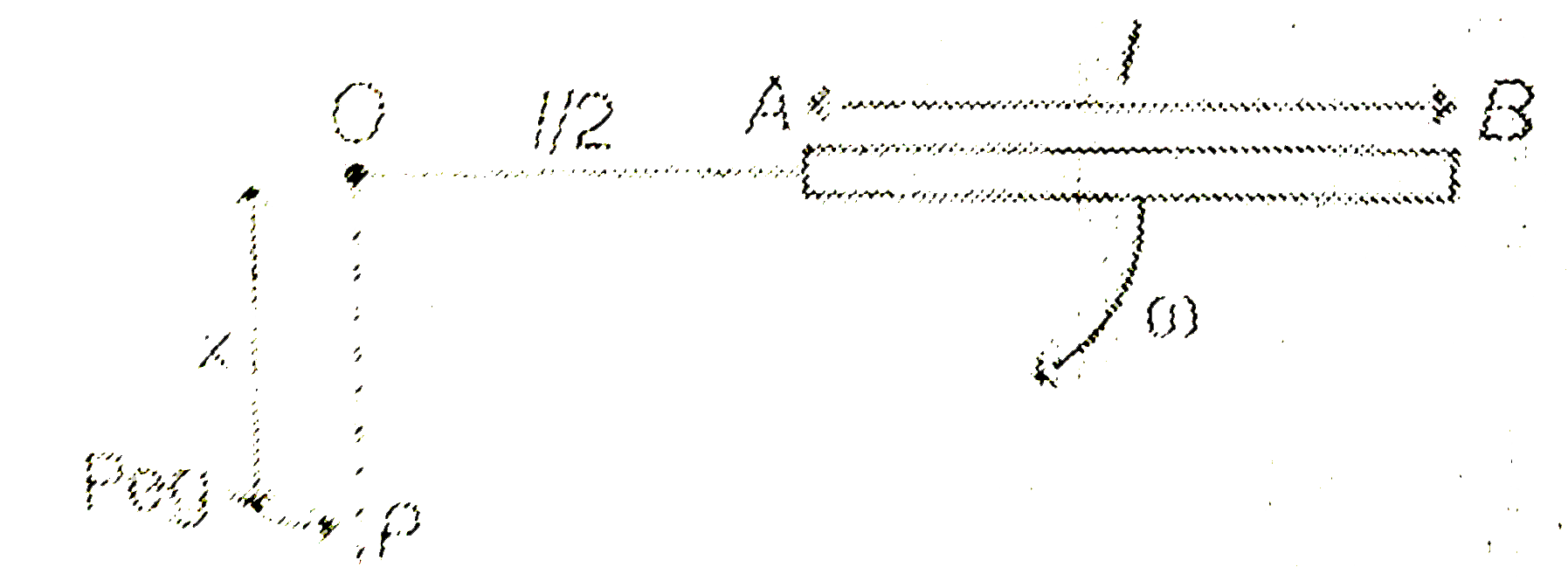 Amagnitude of angular momentum of rod about O is 43l2ωBMagnitude of tension in string is M/ω2CLocation of peg for rod coming to rest is x=1312lDmagnitude of angular impulse by peg on the rod is 43l2ω
Amagnitude of angular momentum of rod about O is 43l2ωBMagnitude of tension in string is M/ω2CLocation of peg for rod coming to rest is x=1312lDmagnitude of angular impulse by peg on the rod is 43l2ωA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A12mω2xB12mω2(1−x2l)C12mω2l(1−x2l2)D12mω2l[1−xl]A uniform stick of length l and mass m lies on a smooth table. It rotates with angular velocity ω about an axis perpendicular to the table and through one end of the stick. The angular momentum of the stick about the end is
Aml2ωBml2ω3Cml2ω12Dml2ω6
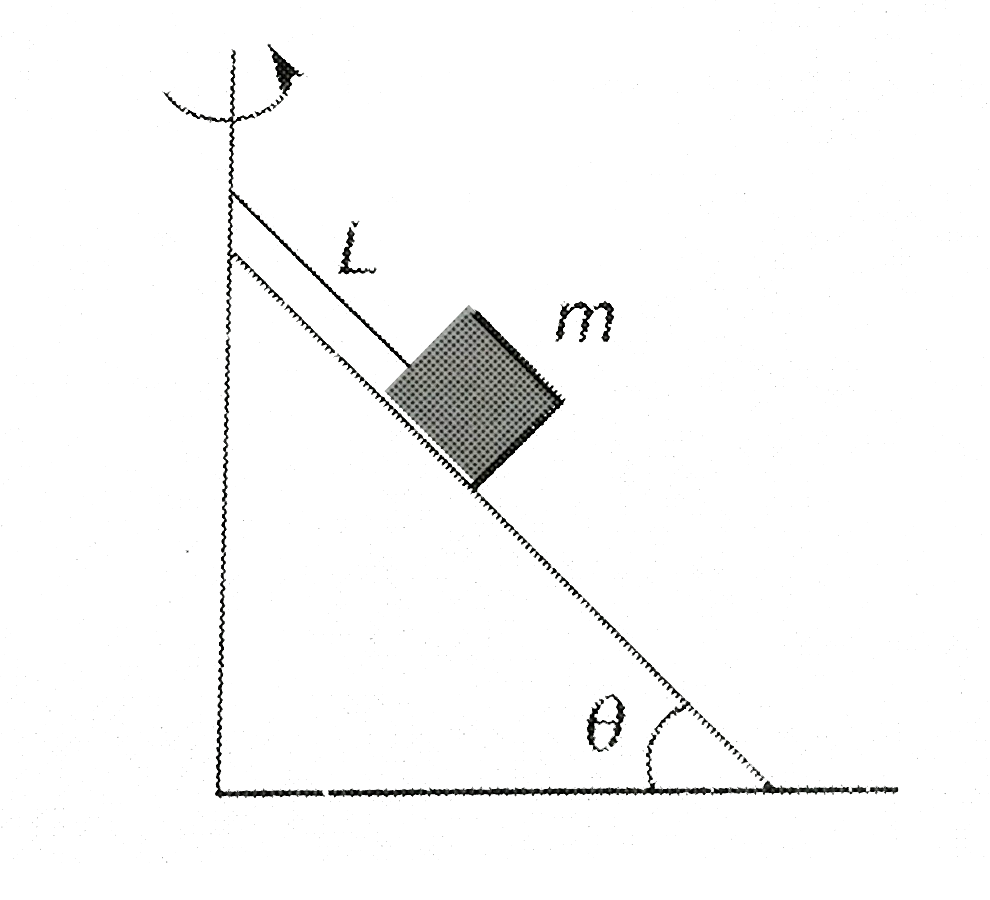
A small block of mass m is tied to the top of a smooth inclined plane with the help of a string of length L as shown in the figure. The inclined plane of inclination θ with horizontal is rotated with an angular velocity ω about a verticle axis passing through the end of the string fixed to the plane.
(a) Find the maximum value of ω so that the block maintains contact with the inclined plane.
(b) Find the ratio of the tension in the string and the normal reaction between the block and the plane.
View SolutionA thin uniform copper rod of length l and cross-section area A and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. The elongation of the rod will be
View SolutionA uniform thin rod of length l and mass m is hinged at a distance l/4 from one of the end and released from horizontal position as shown in Fig. The angular velocity of the rod as it passes the vertical position is

View Solution
A uniform rod of legth L is free to rotate in a vertica plane about a fixed horizontal axis through B. The rod begins rotating from rest. The angular velocity ω at angle θ is given as
View SolutionA uniform rod of mass m length l is attached to smooth hinge at end A and to a string at end B as shown in figure. It is at rest. The angular acceleration of the rod just after the string is cut is :

View Solution
