Two particles of masses M and m (M gt m) are connected by a light string of length πR. The string is hung over a fixed circular frame of radius R.
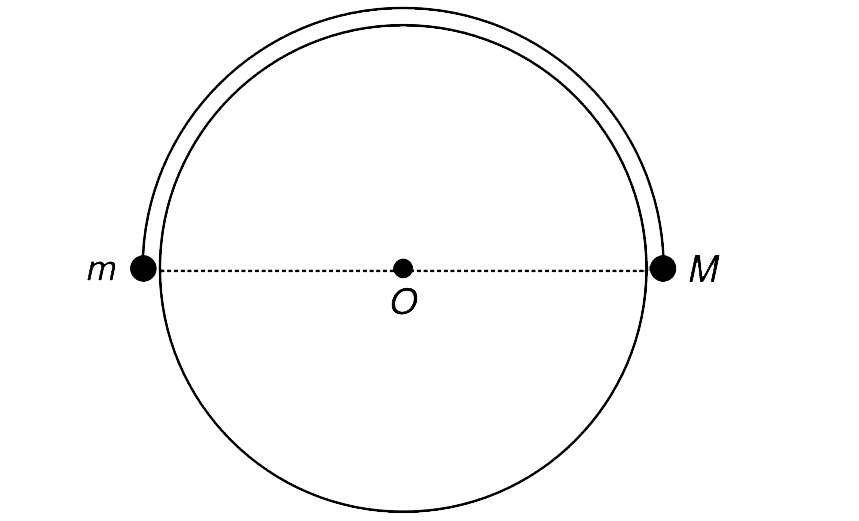
Initially the particles lie at the ends of the horizontal diameter of the circle (see figure). Neglect friction. (a) If the system is released, and if m remains in contact with the circle, find the speed of the masses when M has descended through a distance Rθ(θ<π). (b) Find the reaction force between the frame and m at this instant. (c) Prove that m1 will certainly remain in contact with the frame, just after the release, if 3m gt M.
The correct Answer is:(a) √2gR(Mθ−msinθ)M+m
(b) mgM+m[(M+3m)sinθ−2Mθ]
Step by step video, text & image solution for Two particles of masses M and m (M gt m) are connected by a light string of length piR. The string is hung over a fixed circular frame of radius R. Initially the particles lie at the ends of the horizontal diameter of the circle (see figure). Neglect friction. (a) If the system is released, and if m remains in contact with the circle, find the speed of the masses when M has descended through a distance R theta (theta lt pi). (b) Find the reaction force between the frame and m at this instant. (c) Prove that m1 will certainly remain in contact with the frame, just after the release, if 3m gt M. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
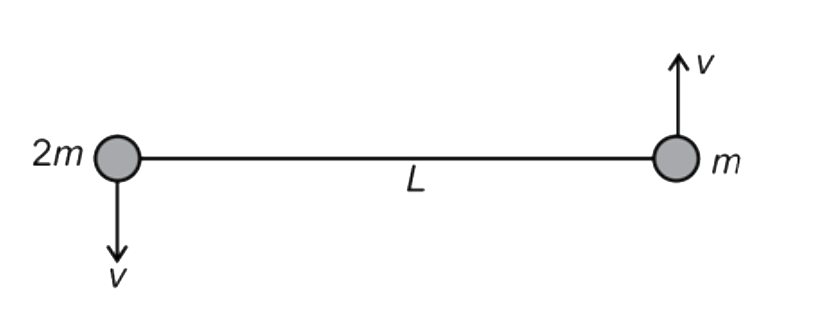
Two particles of mass m and 2m are connected by a string of length L and placed at rest over a smooth horizontal surface. The particles are then given velocities as indicated in the figure shown. The tension developed in the string will be
 View Solution
View SolutionTwo particles of masses m1 and m2 are connected by a light and inextensible string which passes over a fixed pulley. Initially, the particle m1 moves with velocity v0 when the string is not taut. Neglecting friction in all contacting surface, find the velocities of the particles m1 and m2 just after the string is taut.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One or More
Two masses M and m(M>m) are joined by a light string passing over a smooth light pulley.
 AThe acceleration of each block is (M−mM+m)gBThe tension in the string is 2MmgM+mCThe centre of mass of the 'M plus m' system moves down with an acceleration of g(M−mM+m)2DThe tension in the string by which the pulley is attached to the rodd is (M+m)g
AThe acceleration of each block is (M−mM+m)gBThe tension in the string is 2MmgM+mCThe centre of mass of the 'M plus m' system moves down with an acceleration of g(M−mM+m)2DThe tension in the string by which the pulley is attached to the rodd is (M+m)g - Question 2 - Select One
Two particles of masses m and M(M>m) are connected by a cord that passes over a massless, frictionless pulley. The tension T in the string and the acceleration a of the particles is
AT=2mM(M−m)g,aMm(M+m)gBT=2mM(M+m)g,a=(M−mM+m)gCT=(M−mM+m)g,a=(2mM(M+m))gDT=(Mm(M+m)g,a=(2mM(M+m))g - Question 3 - Select One or More
Two masses m and M(m<M) are joined by a light string passing over a smooth and light pulley (as shown)
 Athe acceleration of each mass is (M−mM+m)gBthe tension in the string connecting masses is (2MmM+m)gCthe thrust acting on the pulley is (4MmM+m)gDNone of the above
Athe acceleration of each mass is (M−mM+m)gBthe tension in the string connecting masses is (2MmM+m)gCthe thrust acting on the pulley is (4MmM+m)gDNone of the above
A particle of mass m is suspended from a ceiling through a string of length L. The particle moves in a horizontal circle of radius r such that r =L√2. The speed of particle will be :
View SolutionA particle of mass m is suspended from the ceiling through a string of length L. The particle moves in a horizontal circle of radius r. The speed of the particle is
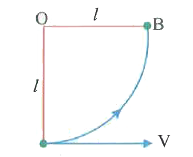
View SolutionA particle of mass m is suspended from a string of length l fixed to the point O. What velocity should be imparted to the particle in its lowermost position so that the string is just able to reach the horizontal diameter of the circle?
 View Solution
View SolutionTwo beads of mass 2m and m, connected by a rod of length l and of negligible mass are free to move in a smooth vertical circular wire frame of radius l as shown. Initially the system is held in horizontal position ( Refer figure )
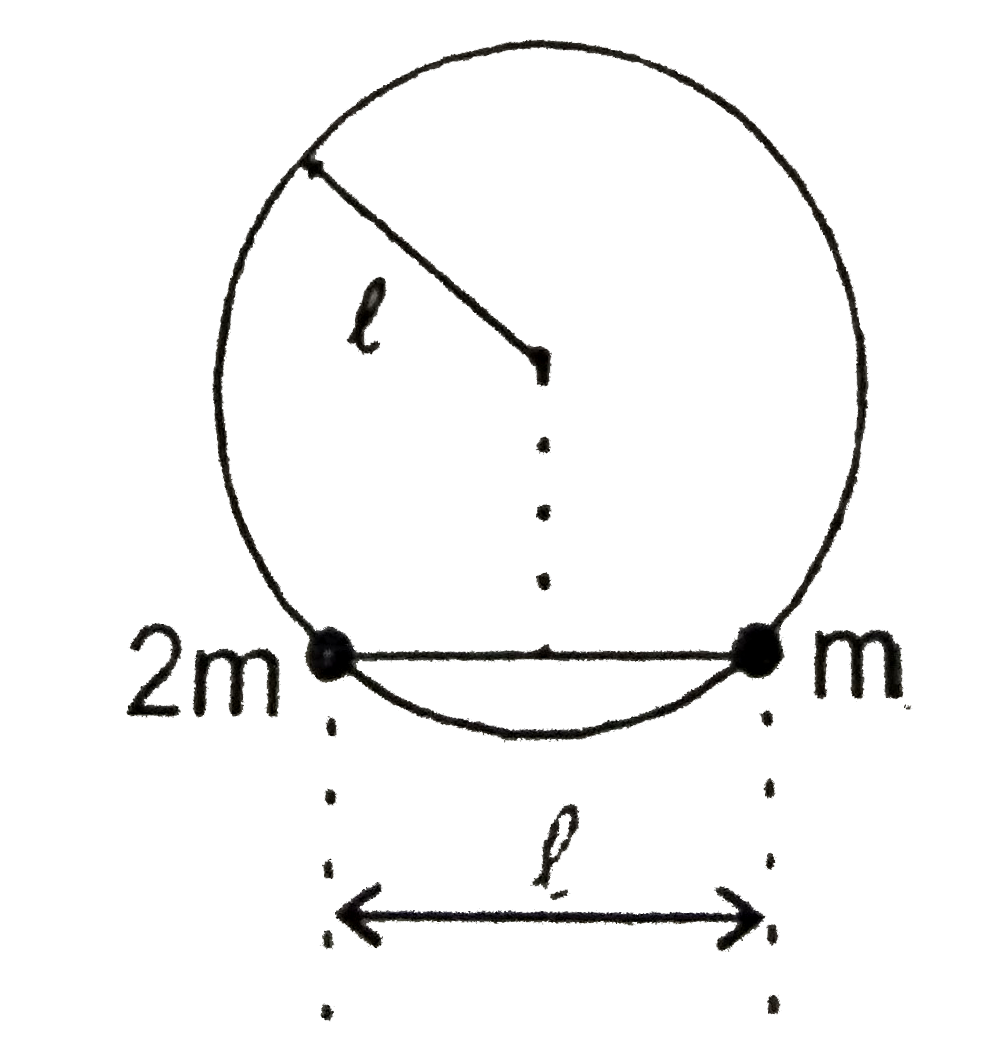
If the rod is replaced by a massless string of length l and the system is released when the string is horizontal then :View SolutionA paricle of mass m is tied to a light string of length L and moving in a horizontal circle of radius r with speed v as shown. The forces acting on the particle are
 View Solution
View Solution

