A hexagonal pencil of mass M and sides length $a$ has been placed on a rough incline having inclination $θ$ Friction is large enough to prevent sliding. If at all the pencil moves, during one full rotation each of its 6 edges, in turn, serve as instantaneous axis of rotation.
(a) Show that for $θ > 30^{\circ}$ the pencil cannot remain at rest.
(b) For inclination of incline $θ < 30^{\circ}$ the pencil will not roll on its own. A sharp impulse J is given to the pencil parallel to the incline at its upper edge (see figure). Friction does not allow the pencil to slide but it begins to rotate about the edge through A with initial angular speed $ω_{0}$ . Find $ω_{0}$ . Moment of inertia of the pencil about its edge is I.
(c) Find minimum value of J so that the pencil will turn about A, and B will land on the incline.
(d) If kinetic energy acquired by the pencil just after the impulse is $K_{0}$ , find its kinetic energy just before edge B lands on the incline

Text Solution

Verified by Experts

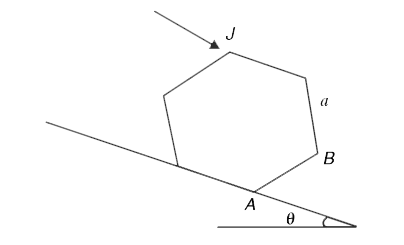
The correct Answer is:(b) $ω_{0} = \frac{\sqrt{3} J a}{I}$
(c) $ω = \frac{\sqrt{2 M g a}}{I} (1 - cos (30^{\circ} - θ)))$
(d) $K = K_{0} + M g a sin θ$

Updated on:21/07/2023

A hollow sphere of mass m and radius R is rolling downdard on a rough inclined plane of inclination $θ$ . If the coefficient of friction between the hollow sphere and incline is $μ$ , then
AFriction opposes its translation
BFriction supports rotation motion
COn decreasing $θ$ , frictional force decreases
DAll of these
A block of mass $m$ rests on a fixed incline plane of inclination $θ$ with horizontal Assume friction is large enough to make the block stationary. Then choose correct alternative (s)
AMaximum value of horizontal component of friction is mg
Bangle $θ$ at which horizontal component of friction is maximum is $\frac{π}{4}$
CMaximum value of horizontal component of friction is $\frac{m g}{2}$
DAngle $θ$ at which horizontal component of friction is maximum is $\frac{π}{3}$
A solid cylinder of mass $m$ and radius $r$ is rolling on a rough inclined plane of inclination $θ$ . The coefficient of friction between the cylinder and incline is $μ$ . Then.
Africtional force is always $μ m g cos θ$
Bfriction is a dissipative force
Cby decreasing $θ$ , frictional force decreases
Dfrictiona opposes translation and supports rotation.