A disc of radius R stands at the edge of a table. If the given a gentle push and it beings to fall. Assume that the disc does not slip at A and it rotates about the point as it falls. The falling disc hits the edge of another table placed at same height as the first one at a horizontal distance of √2R. Imagine that the disc hits the edge B and rotates (up) about the edge
(a) Find the speed of the centre of the disc at the instant just before it hits the edge B.
(b) Find the angular speed of the disc about B just after the hit.
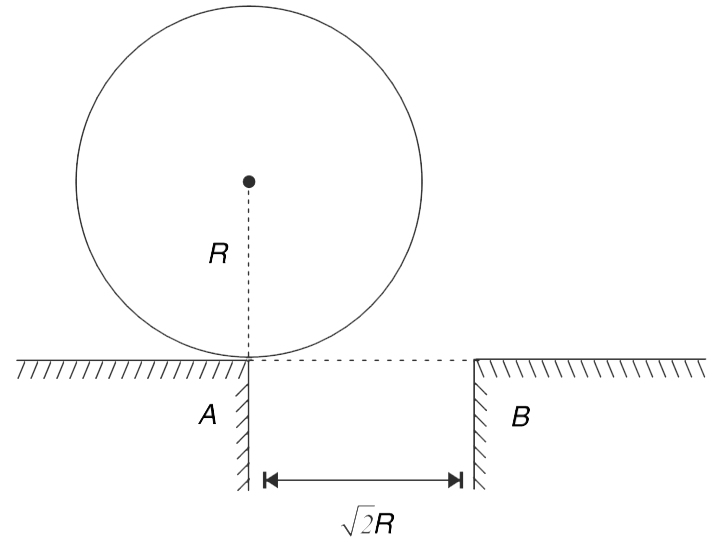
The correct Answer is:
ω=13
⎷⎛⎜
⎜⎝2√2(√2−1)3gR⎞⎟
⎟⎠
Step by step video, text & image solution for A disc of radius R stands at the edge of a table. If the given a gentle push and it beings to fall. Assume that the disc does not slip at A and it rotates about the point as it falls. The falling disc hits the edge of another table placed at same height as the first one at a horizontal distance of sqrt2 R. Imagine that the disc hits the edge B and rotates (up) about the edge (a) Find the speed of the centre of the disc at the instant just before it hits the edge B. (b) Find the angular speed of the disc about B just after the hit. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
A disc of mass M and radius R is kept flat on a smooth horizontal table. An insect of mass m alights on the periphery of the disc and begins to crawl along the edge.
(a) Describe the path of the centre of the disc.
For what value of mM the centre of the disc and the insect will follow the same path ?
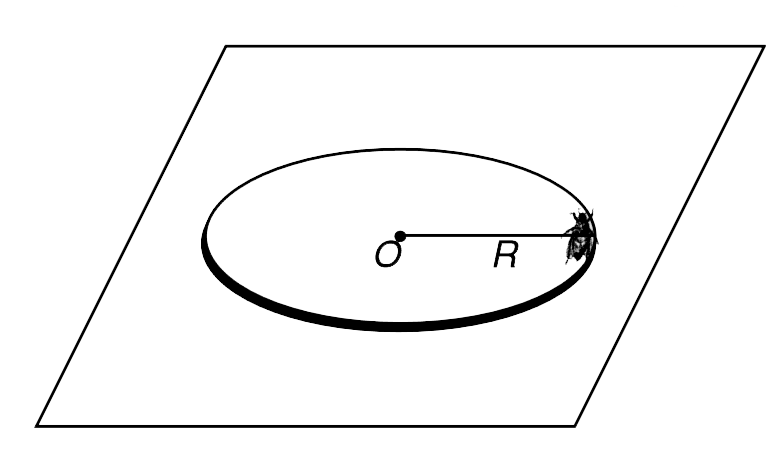 View Solution
View SolutionA small mass m rests at the edge of a horizontal disc of radius R. The coefficient of static friction between the mass and the disc is μ. The disc is rotated about its axis at an angular velocity such that the mass slides off the disc and lands on the floor h metres below. The horizontal "distance of travel of the mass is : √kμRh. Find k.
View Solution
Knowledge Check
- Question 1 - Select One
An ant is sitting at the edge of a rotating disc. If the ant reaches the other end, after moving along the diameter, the angular velocity of the disc will:-
Aremain constantBfirst decreases and then increasesCfirst increases, then decreaseDIincrease continuously - Question 2 - Select One
A uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is
 A49mL2ω224B2mω2L2C124mω2L2D13mL2ω224
A49mL2ω224B2mω2L2C124mω2L2D13mL2ω224 - Question 3 - Select One
A disc of radius r is cut from a larger disc of radius 4r in such a way that the edge of the hole touches the edge of the disc. The centre of mass of the residual disc will be a distance from centre of larger disc :-
Ar5Br4Cr2Dr3
A disc is of mass M and radius r. The moment of inertia of it about an axis tangential to its edge and in plane of the disc or parallel to its diameter is
View SolutionA disc of radis R is cut out from a larger disc of radius 2R in such a way that the edge of the hole touches the edge of the disc. Locate the centre of mass of the residual disc.
View Solution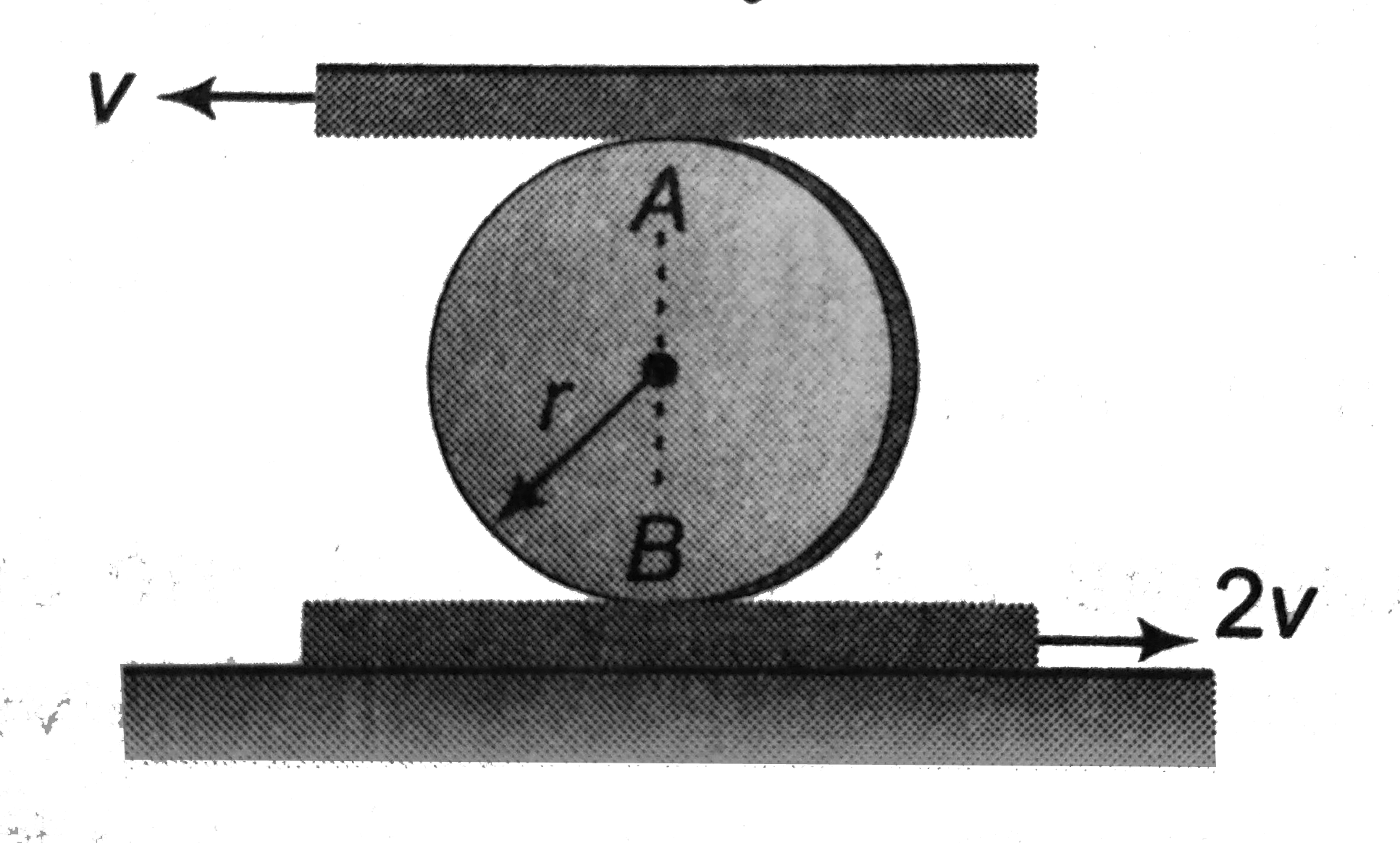
the disc of the radius r is confined to roll without slipping at A and B if the plates have the velocities shown, determine the angular velocity of the disc.View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is
View SolutionThe moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is.
View Solution

