A point charge Q has been placed at a point outside a neutral spherical conductor. The induced charge density at point P on the surface of the conductor is −σ The distance of point P from the point charge Q is 2R (where R is radius of the conductor). Find the magnitude and direction of electric field at a point outside the conductor that is very close to its surface near P.
The correct Answer is:σε0 in radially inward direction.
Topper's Solved these Questions
Similar Questions
A point charge Q is placed outside a hollow spherical conductor of radius R , at a distance r(r>R) from its centre C . The field at C due to the induced charges on the conductor is
View SolutionA point charge +q is held at a distance R from the centre of an uncharged spherical conductor of radius a(a<R). Calculate the potential of the conductor in the presence of q.
View Solution
Knowledge Check
Electric intensity at a point just outside a charged conductor of any shape is
Aσ∈0KBσ2∈0KC2σ∈KDσ22∈KThe electric intensity at a point near a charged conductor of surface charge density σ is
AE=σ/∈0kBE=σ∈0kCE=σ/2∈0kDE=σ2If q is the charge per unit area on the surface of a conductor, then the electric field intensity at a point on the surface is
A(qε0) normal to surfaceB(q2ε0) normal to surfaceC(qε0) tangential to surfaceD(q2ε0) tangential to surface
A point charge +q is held at a distance R from the centre of an uncharged spherical conductor of radius a(a<R). Calculate the potential of the conductor in the presence of q.
View SolutionAt a point inside a charged conductor the
View SolutionA point charge Q is placed outside a hollow spherical conductor of radius R , at a distance r(r>R) from its centre C . The field at C due to the inducted charges on the conductor is
View SolutionElectric field intensity at a point a hollow charged spherical conductor
View SolutionA point charge q1 is placed inside cavity 1 and another point charge q2 is inside cavity 2. A point charge q is placed outside the conductor. For the situation described above, answer the following questions.
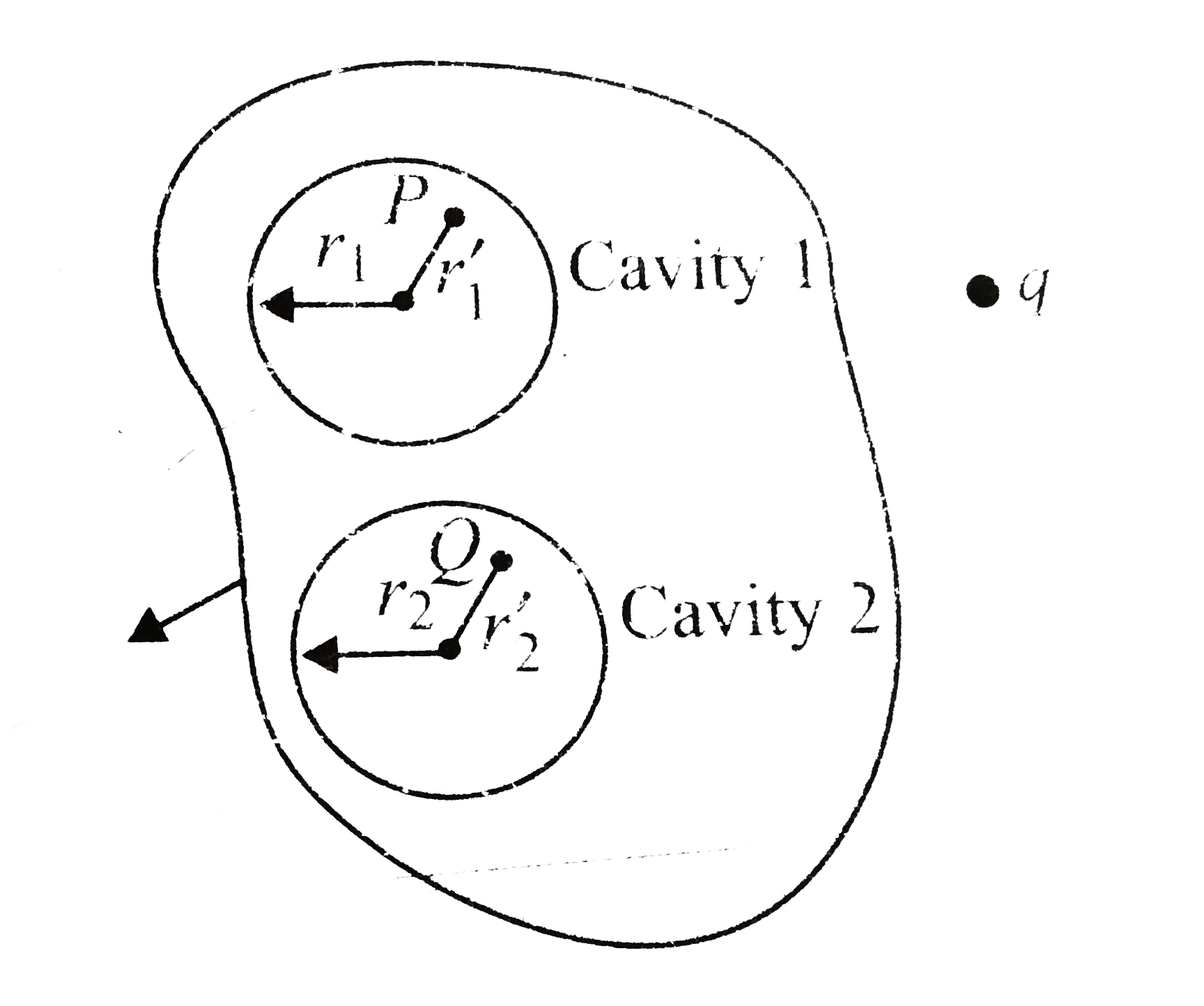
The charge on outer surface of the conductor would be
View Solution
