In the figure shown ABC is a circle of radius a. Arc AB and AC each have resistance R. Arc BC has resistance 2R. A current I enters at point A and leaves the circle at B and C. All straight wires are radial. Calculate the magnetic field at the centre of the circle. Each arc AB, BC and AC subtends at the centre of the circle.

The correct Answer is:
Topper's Solved these Questions
Similar Questions
In the adjoining figure, O is the centre of the circle and AB is its diameter. If AC=8 cm and BC= 6cm, then find the radius of the circle.

View SolutionIn the adjoining figure, O is the centre of the circle and AB is its diameter. If AC=8 cm and BC= 6cm, then find the radius of the circle.

View Solution
Knowledge Check
In a circle of radius r cm, an arc subtends an angle of 60∘ at the centre. The length of the arc will be :
Aπr3cmB2πr3cm2Cπr23cm2Dπr3cm2A uniform wire is bent in the form of a circle of radius R. A current I enters at A and leaves at C as shown in the figure :
If the length ABC is half of the length ADC, the magnetic field at the centre O will be
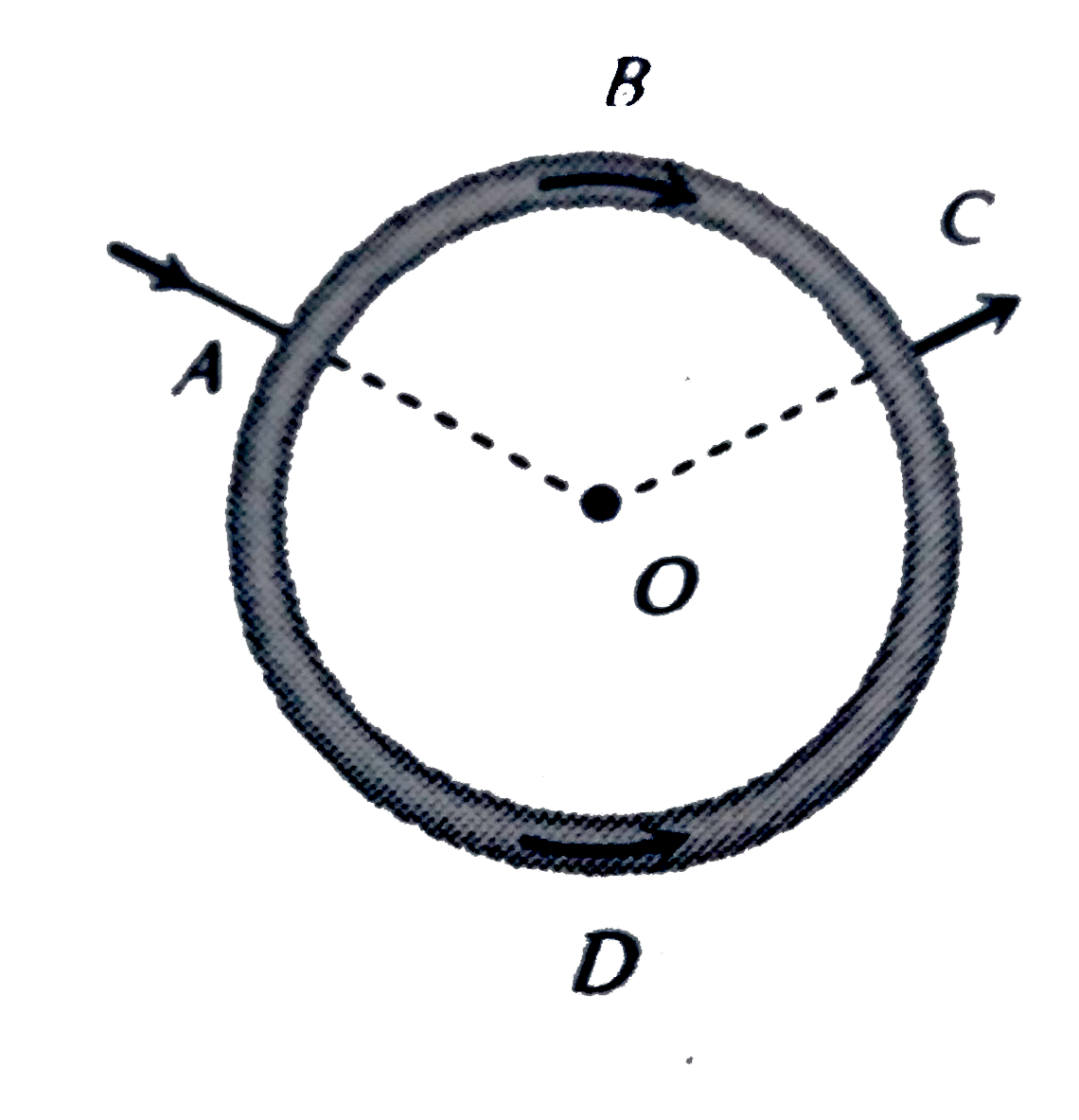 AZeroBμ0I2RCμ0I4RDμ0I6R
AZeroBμ0I2RCμ0I4RDμ0I6RIn the figure, the curved part represents arc of a circle of radius x. If it carries a current I then the magnetic field at the point O is
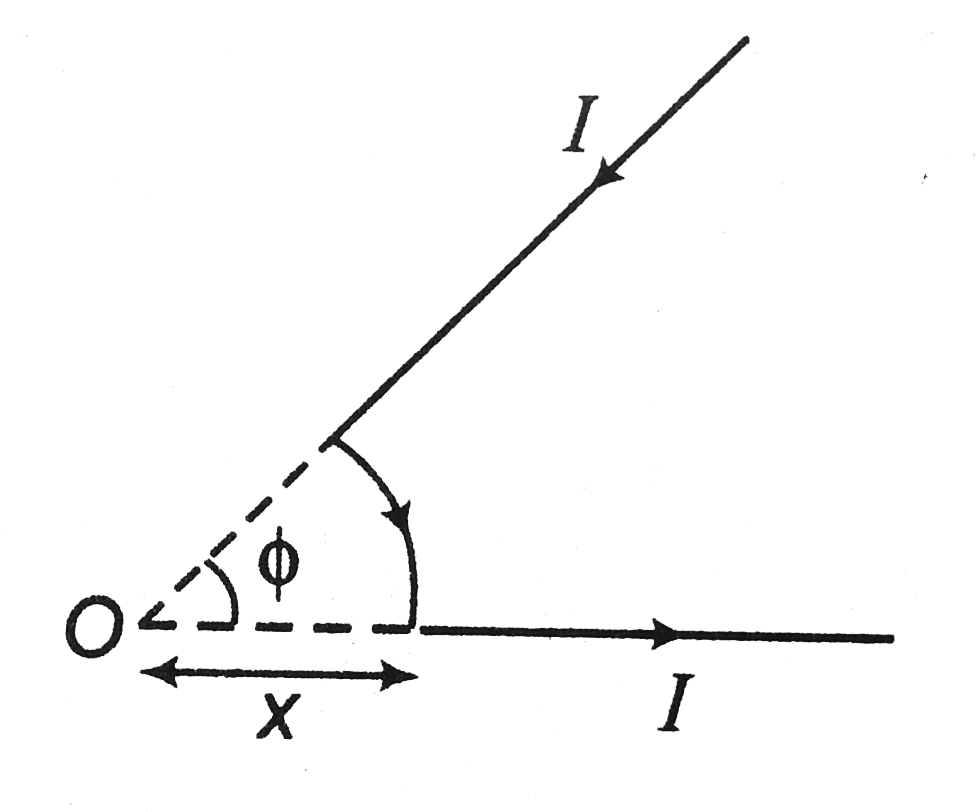 Aμ0Iϕ2πxBμ0Iϕ4πxCμ0Iϕ2xDμ0Iϕ4x
Aμ0Iϕ2πxBμ0Iϕ4πxCμ0Iϕ2xDμ0Iϕ4x
In the figure, the curved part represents arc of a circle of radius x. If it carries a current I then the magnetic field at the point O is

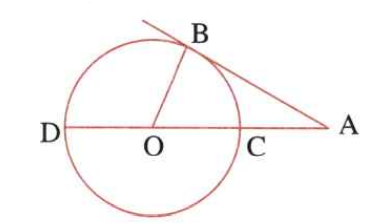
View SolutionIn the given figure, O is the centre of the circle ans AB is a tangent at B. If AB=15 cm and AC=7.5cm, calculate the radius of the circle.
View SolutionA straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View SolutionIn the given 'O' is the centre of the circle, Arc AB=ArcBC=Cd.If∠OAB=48∘, find:
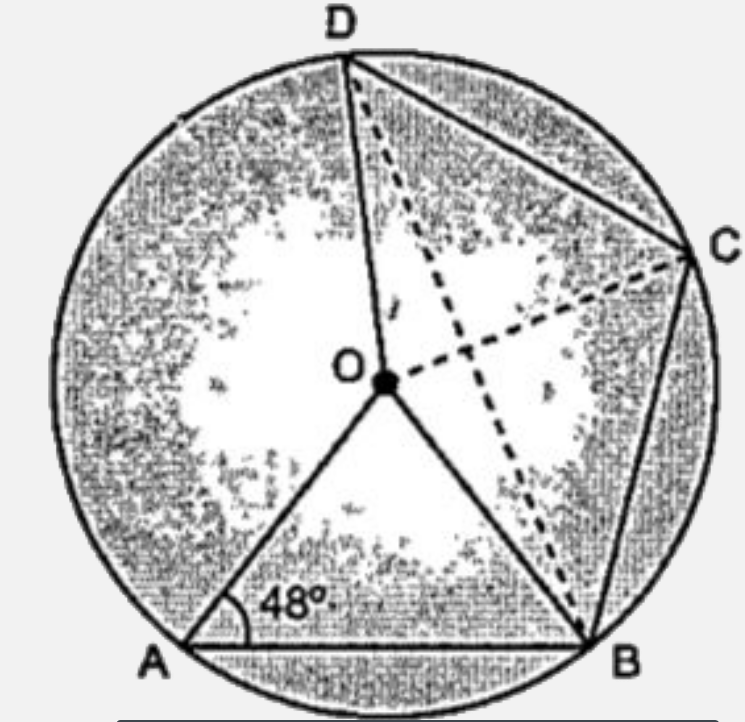
∠OBD
View SolutionIn the given figure, O is the centre of a circle and arc ABC subtends an angle of 130∘ at O. AB is extended to P, Then, ∠PBC is equal to
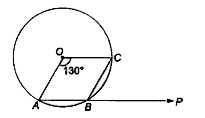
View Solution
