A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom. Match the following:

Column-1 Column-2(A)Velocity at B(P) 7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5mg(T)None
The correct Answer is:A - R, B - Q, C - P, D - T
Step by step video, text & image solution for A particle is suspended from a string of length R. It is given a velocity u= 3 sqrt(gR) at the bottom. Match the following: {:(,"Column-1",," Column-2"),("(A)","Velocity at B",,"(P) 7 mg"),("(B)","Velocity at C",,(Q)sqrt(5gR) ),("(C)","Tension in string at B",,(R)sqrt(7gR)),("(D)","Tension in string at C",,(S) 5 mg),(,,,"(T)None"):} by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View SolutionA particle of mass m is suspended from a string so that it can move in a circular path in vertical plane. It is given v=3√glat the bottom most point. Match the values of tension in string at A,B,C,D
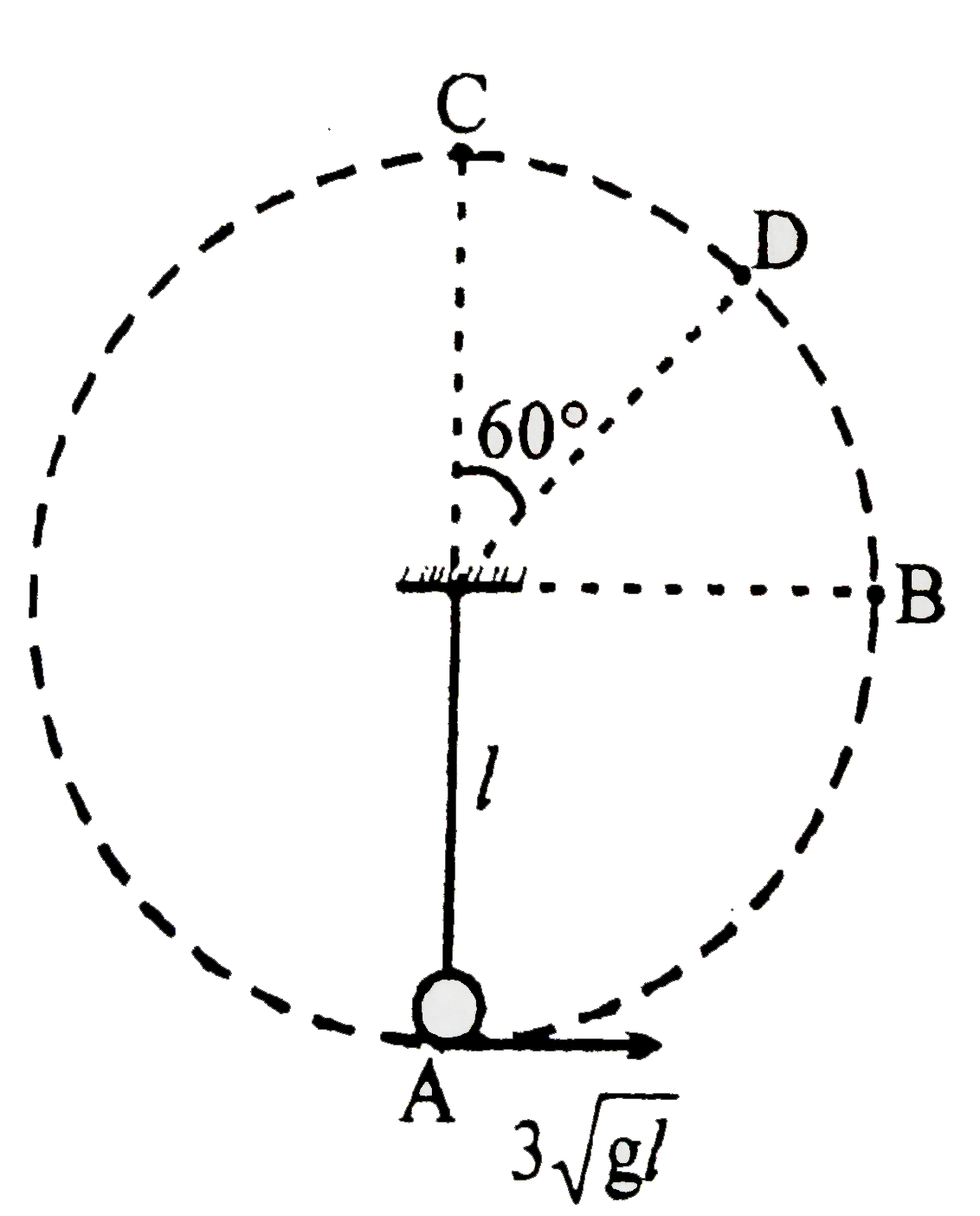
Column IColumn II(A) TA(P) 10 mg ),((B) T_(B),,(Q)7mg),((C) T_(C),,(R)4mg),((D) T_(D),,(S)5mg),(,,(T)5.5mg
View Solution
Knowledge Check
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the lowest point.

column−I column−II(A)Velocity at B (P)7mg(B) Velocity at C (Q)√5qR(C)Tension in string at B(R) (R)√7gR(D)Tension in string atC(S) (S)4mgAA-R, B-Q, C-P, D-SBA-Q, B-R, C-S, D-PCA-R, B-P, C-Q, D-SDA-P, B-S, C-R, D-QMatch the following columns. (for a satellite in circular orbit)
Column-IColumn-II(A)Kinetic energy(p)−GMm2r(B)Potential energy(q)√GMr(C)Total energy(r)−GMmr(D)Orbital speed(s)GMm2rA(A→s,B→r,C→p,D→q)B(A→c,B→s,C→p,D→q)C(A→s,B→r,C→q,D→p)D(A→q,B→r,C→p,D→s)
Sound is travelling in a long tube towards right and the graph of excess pressure variation Vs position (at some instant) is given below.
 Match velocities in column-I with column-II P,Q,R,S,T are medium particles inside the tube.
Match velocities in column-I with column-II P,Q,R,S,T are medium particles inside the tube.
Column-IColumn-II(A)velocity is towards right(P)P(B)velocity is towards left(Q)Q(C)velocity is zero(R)R(D)Speed is maximum(S)S(T)T
View SolutionMatch the following
Column-IColumn-II(A)Even number(p)227(B)Rational number(q)π(C)Irrational number(r)0(D)Real number(s)√2(t)1.¯¯¯¯¯¯234
View SolutionMatch the following:
Column-IColumn-II(A) Root mean square velocity(p)√3Pd(B) Average velocity(q)√3RTm(C) Most probable velocity(r)√8Pπd(D) Velocity possessed by maximum fraction of molecules(s)√2RTm
View SolutionIn the diagram strings, springs and the pulley are light and ideal. The system is in equilibrium with the strings taut (T>0), match the column. Masses are equal.
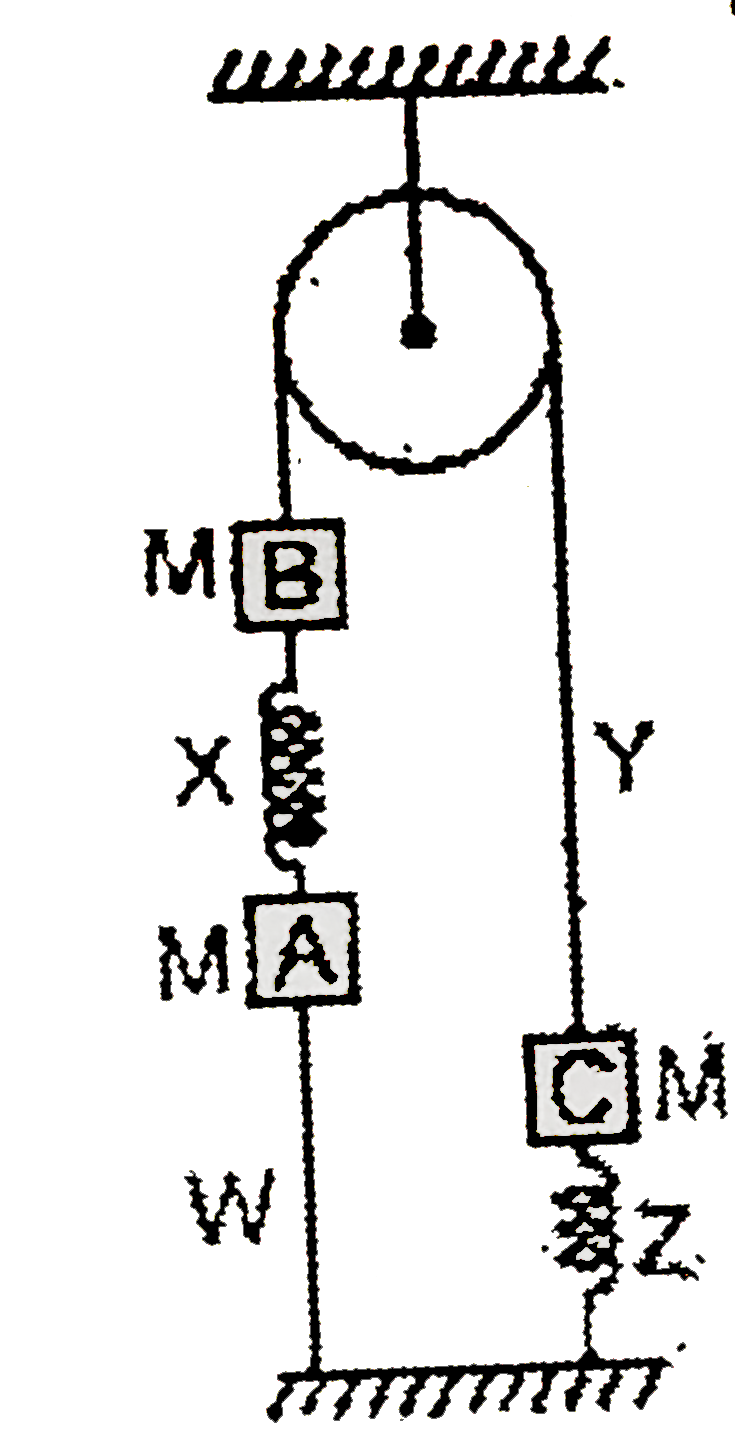
∣∣ ∣ ∣ ∣ ∣ ∣∣Column -1Column -2(A)Just after string W breaks(P)aA=0(B)Just after spring X breaks(Q)aB=0(C)Just after string Y breaks(R)aC=0(D)Just after spring Z breaks(S)aB=aC∣∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionIn y=Asinωt+Asin(ωt+2π3)
∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣Column-IColumn-II(A)Motion(p)is periodic but no SHM(B)Amplitude(q)is SHM(C)Initial phase(r)A(D)Maximum velocity(s)π/3(t)ωA/2(u)None∣∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣∣
View SolutionColumn IColumn II(A)Work(p)[A1/2T−1](B)Moment of inertia(q)[FA1/2](C)Velocity(r)[FA1/2T2]
View Solution