In the figure shown the spring is compressed by 'x0' and released . Two block 'A' and 'B' of masses 'm' and '2m' respectively are attached at the ends of the spring. Blocks are kept on a smooth horizontal surface and released. Find the work done by the spring on 'A' by the time compression of the spring reduced to x02.

The correct Answer is:4
Let the speed of the blocks A and B at the instant compression x04 be vA and vB respectively as shown. (l0 is natural length)
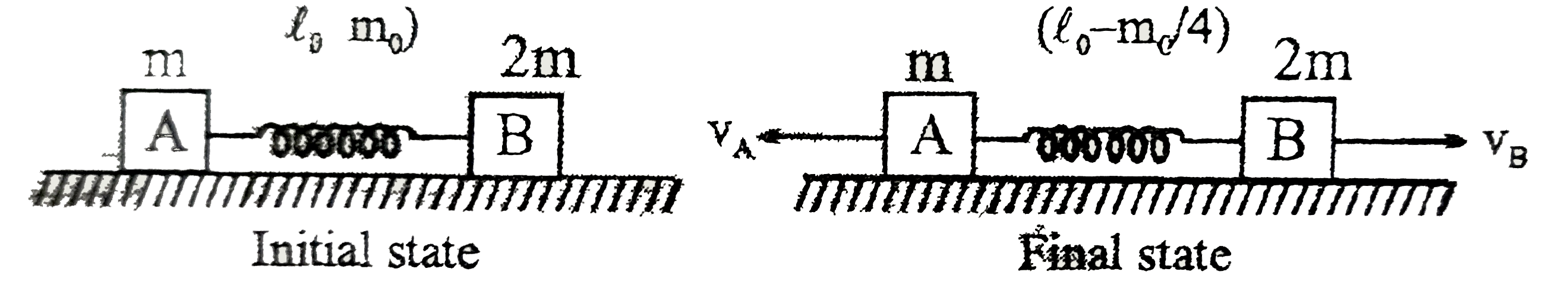
Since, No external force acts on the system in Hz direction, therefore applying linear momentum conservation in Hz direction.
0=m(−vA)+2m(vB) or
vA=2vB......(1)
Now, By conservation of energy,
12kx20=12k(x02)2+12(m)v2A+12(2m)v2B
From (1) and (2) we get
12mv2A=12kx20
Using work energy theorem,
W.d by spring on A =change in K.E. of A
or WS=12mv2A=516kx20
Topper's Solved these Questions
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)32videos
TEST PAPERS
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)3videos
TEST PAPERS
- BOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C4videos
TEST PAPERS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
Two blocks A and B of mass m and 2m respectively are connected by a light spring of force constant k. They are placed on a smooth horizontal surface. Spring is stretched by a length x and then released. Find the relative velocity of the blocks when the spring comes to its natural length
 View Solution
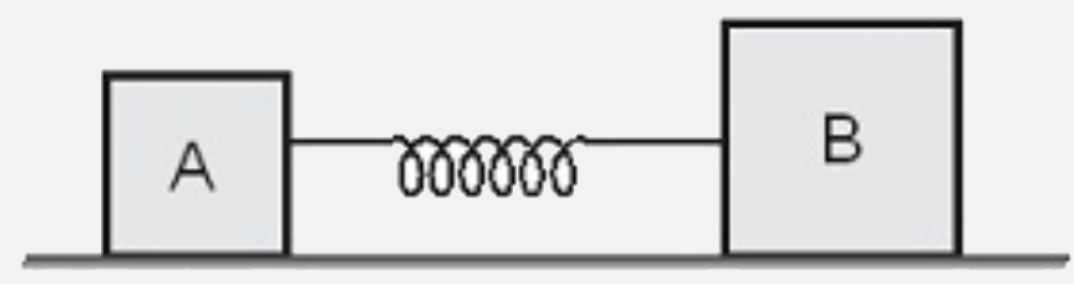
View SolutionTwo blocks A and B of masses m and 2m, respectively , are connected by a massless spring of force constant k and are placed on a smooth horizontal plane. The spring is stretched by an amount x and then released . The relative velocity of the blocks when the spring comes to its natural length is
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. Two maximum compression in spring is
 A√2mkv0B√mk.v0C√mk.v0Dm2k.v0
A√2mkv0B√mk.v0C√mk.v0Dm2k.v0 - Question 2 - Select One
a block of mass 0.1 kg attached to a spring of spring constant400Nm pulled horizontally from x=0 tox1=10mm. Find the work done by the spring force
A2⋅10−2JB2⋅10−6JC3⋅10−6JD4⋅10−2J - Question 3 - Select One
All surfaces shown in figure are smooth. System is released with the spring unstretched. In equilibrium, compression in the spring will be
 Amg√2KB2mgkC(M+m)g√2kDmgk
Amg√2KB2mgkC(M+m)g√2kDmgk
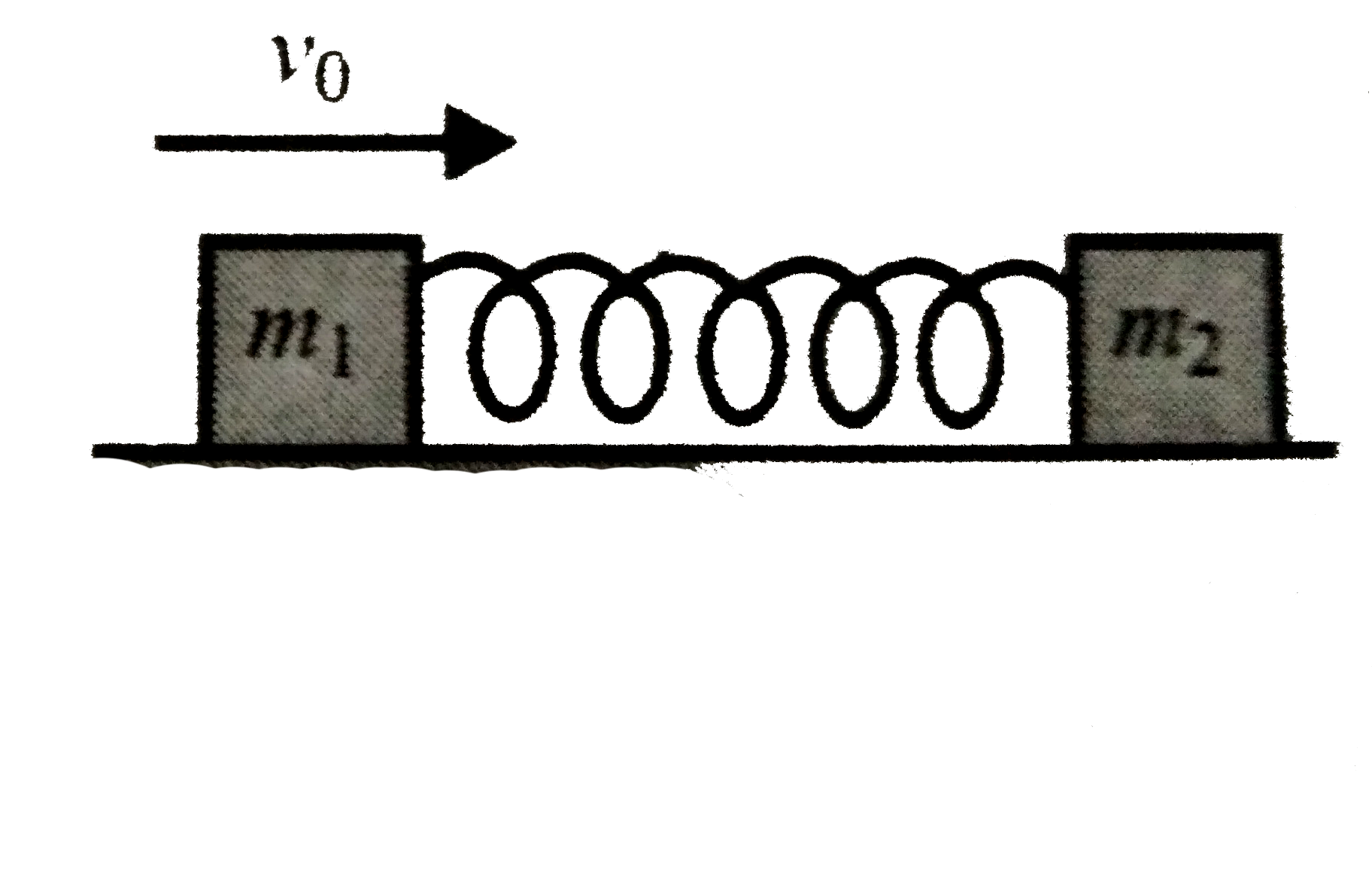
Two smooth blocks of mases m1andm2 attached with an ideal spring of stiffness k and kept on hrozontal surface. If m1 is projected with a horizontal velocity v0. Find the maximum compression of the spring.
 View Solution
View SolutionTwo blocks of masses m and M connected by a light spring of stiffness k, are kept on a smooth horizontal surface as shown in figure. What should be the initial compression of the spring so that the system will be about to break off the surface, after releasing the block m1?
View SolutionAll surfaces shown in figure are smooth. System is released with the spring instretched. In equilibrium, compression in the spring will be
 View Solution
View SolutionTwo blocks of masses 6 kg and 3 kg are attached to the two ends of a massless spring of spring constant 2π2N/m. If spring is compressed and released on a smooth horizontal surface then find the time period (in seconds) of each block.
View SolutionTwo blocks of masses m1 and m2 are connected by a massless spring and placed on smooth surface. The spring initially stretched and released. Then :
View Solution

