Sara correctly solves a system of two lines equations and finds that the system has no solution. If one of the two equation is y6=x4=1, which could be the oher equation in this system?
y=23x+12
y=32x
y=−32x
y=32x+6
The correct Answer is:B
To solve the problem, we need to find the other equation in the system that results in no solution when paired with the given equation. The given equation is:
y6=x4=1
Step 1: Rewrite the given equation
We can break down the given equation into two separate equations:
1. y6=1
2. x4=1
From these, we can solve for y and x:
- From y6=1, we multiply both sides by 6:
y=6
- From x4=1, we multiply both sides by 4:
x=4
Step 2: Identify the condition for no solution
For a system of two linear equations to have no solution, the lines represented by the equations must be parallel. This means they have the same slope but different y-intercepts.
Step 3: Analyze the options
We need to check which of the provided options results in a line that is parallel to the line represented by the given equation.
The line from the given equation can be rewritten in slope-intercept form y=mx+b. We already know that:
y=6(a horizontal line)
Step 4: Check each option
We will check each option to see if it represents a line parallel to y=6:
1. Option A: y=2/3x+12
- This has a slope of 2/3 (not parallel).
2. Option B: y=3/2x
- This has a slope of 3/2 (not parallel).
3. Option C: y=−1/2x+6
- This has a slope of −1/2 (not parallel).
4. Option D: y=6
- This has a slope of 0 (parallel to the given line).
Conclusion
The only equation that results in no solution when paired with the given equation is:
y=6
We can break down the given equation into two separate equations:
2. x4=1
y=6
x=4
For a system of two linear equations to have no solution, the lines represented by the equations must be parallel. This means they have the same slope but different y-intercepts.
We need to check which of the provided options results in a line that is parallel to the line represented by the given equation.
We will check each option to see if it represents a line parallel to y=6:
- This has a slope of 2/3 (not parallel).
2. Option B: y=3/2x
- This has a slope of 3/2 (not parallel).
3. Option C: y=−1/2x+6
- This has a slope of −1/2 (not parallel).
4. Option D: y=6
- This has a slope of 0 (parallel to the given line).
The only equation that results in no solution when paired with the given equation is:
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Ben correctly solves a system of two linear equations and finds that the system has an infinite number of solutions. If one of the two equations is 3(x+y)=6−x, which could be the other equation in this system?
Ay=34x+2By=43xCy=−43x+2Dy=−43x+6 - Question 2 - Select One
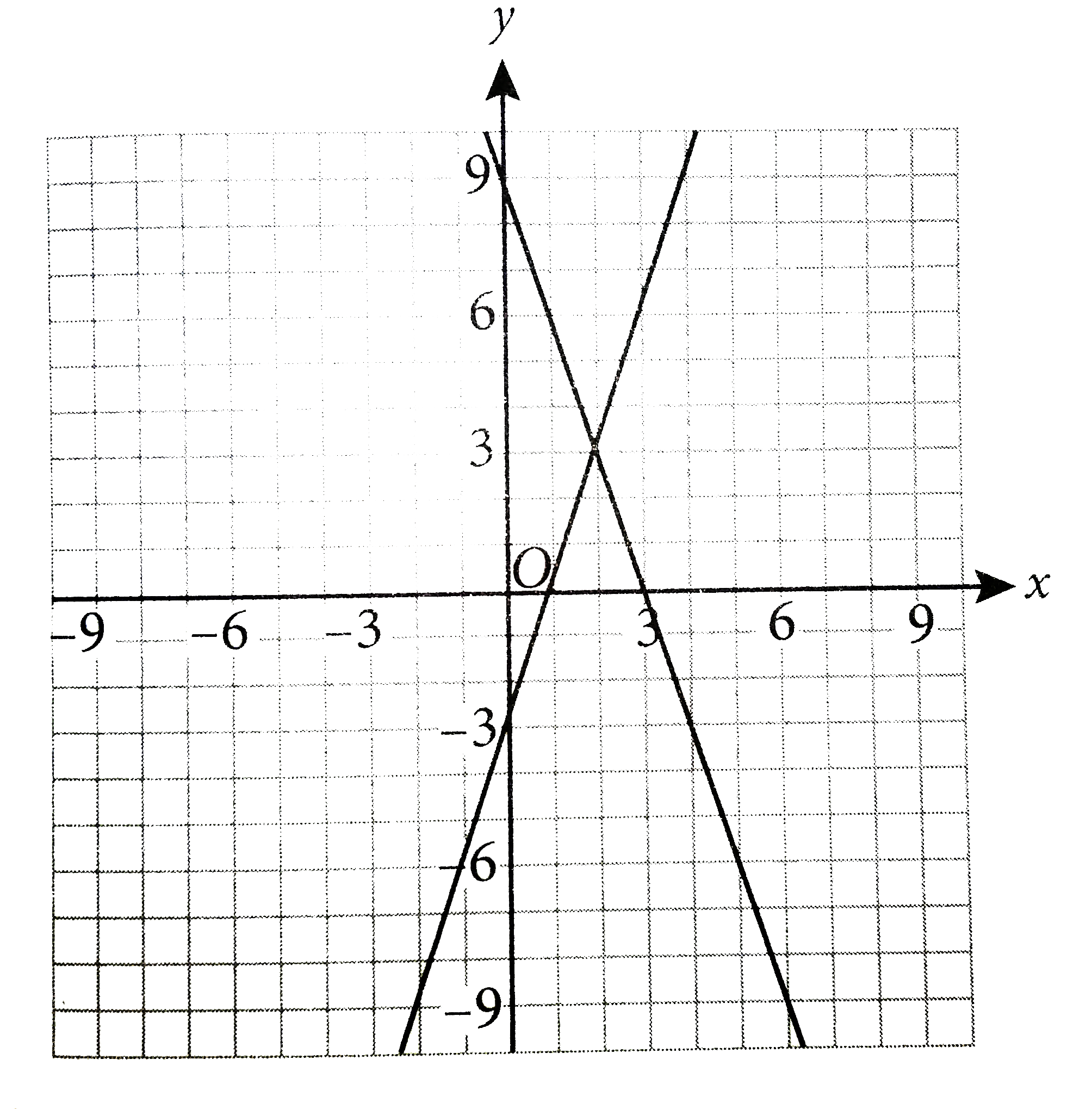
The lines graphed in the xy-plane above represent a system of two linear equations. What is the solution (x,y) to the system?A(-1,-6)B(0,-3)C(2,3)D(3,0) - Question 3 - Select One

The tables above represent data points for two linear equations. If the two equations form a system, what is the x-coordinate of the solution to that system ?A4B6C8D-4
11x−24y=8
kx−36y=5
In the system of equations above, k is a constant. If the system has no solutions, what is the value of k?View SolutionThe system of equations ax−y−z=a−1,x−ay−z=a−1,x−y−az=a−1 has no solution if a is:
View Solution32x−12y=7
kx−6y=4
In the system of linear equation above, is a constant. If the system has no solution, what is the value of k?View SolutionShow that the following systems of linear equations has infinite number of solutions and solve x+2y=5, 3x+6y=15
View SolutionWhich is a solution to the following system of equations ?
y+x2=6x−3
y−x=1View Solution

