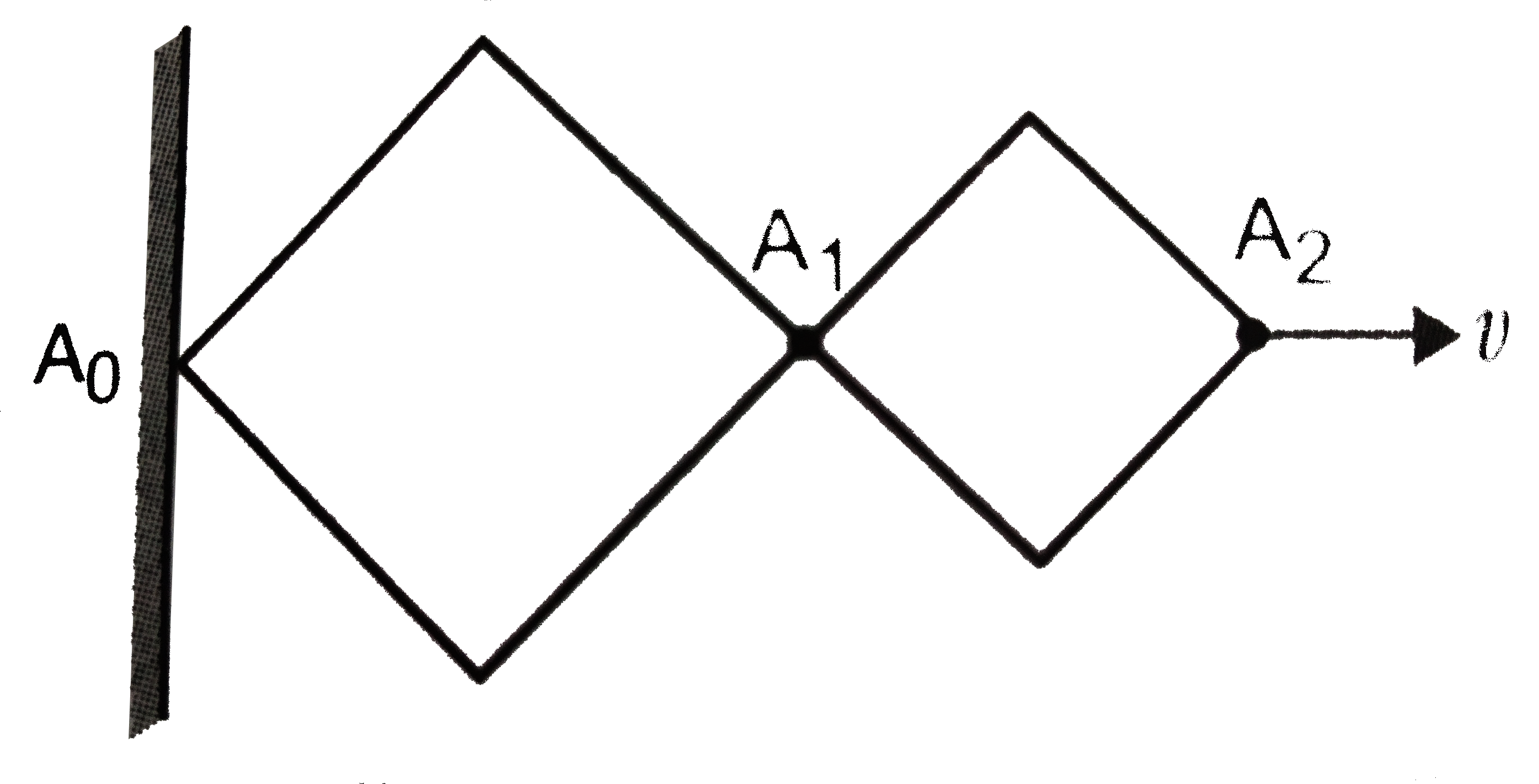
A hinged construction consists of three rhombs with the ratio of sides 3 : 2 : 1

(Fig). Vertex A3 moves in the horizontal direction at a velocity v. Determine the velocities of vertices A1,A2 and B2 at the instant when the angles of the construction are 90∘
Step by step video & image solution for A hinged construction consists of three rhombs with the ratio of sides 3 : 2 : 1 (Fig). Vertex A_(3) moves in the horizontal direction at a velocity v. Determine the velocities of vertices A_(1), A_(2) and B_(2) at the instant when the angles of the construction are 90^(@) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
The given construction as shown in Fig. 2 (HT) .3, consists of two rhombus with the ration 3:2,ThevetexA_2movesInthehorizontaldirectionwithavelocity v.F∈dthevelocityof A_1.
 View Solution
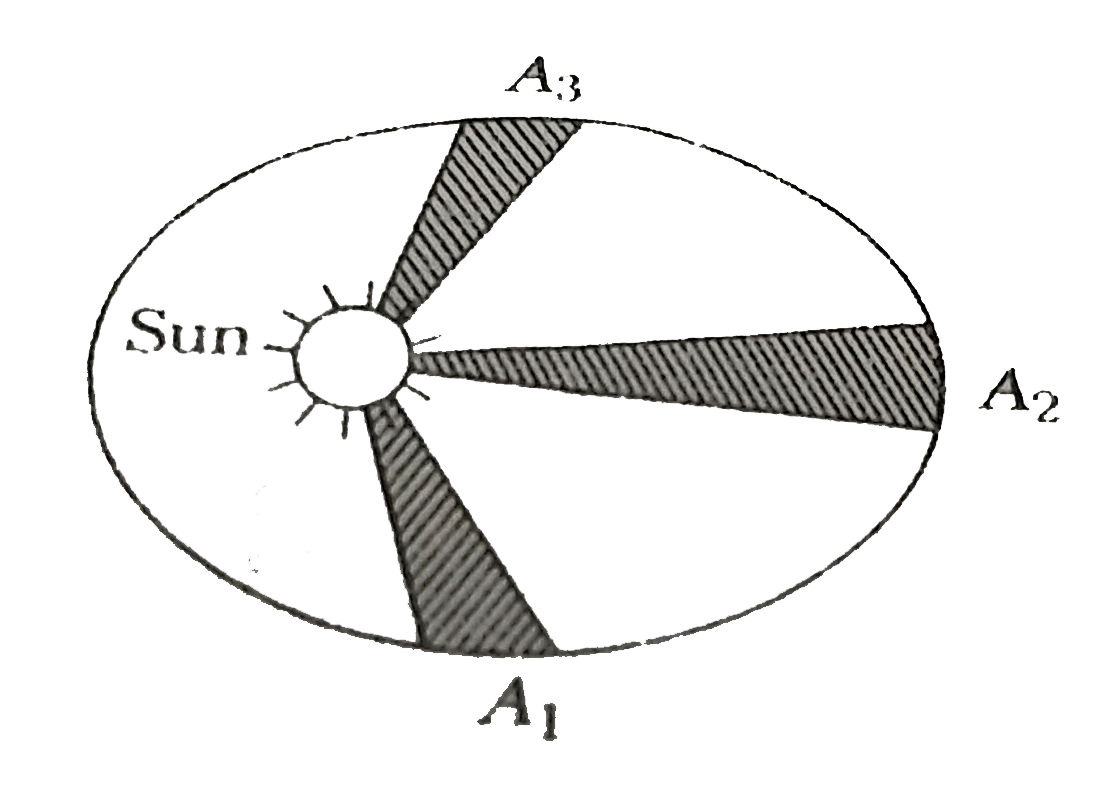
View SolutionA planet moving around sun sweeps area A1 in 2 days, A2 in 3 days and A3 in 6 days, then find the relation between A1,A2andA3
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Three blocks A, B, and C of masses 3M,2M, and M are suspended vertically with the help of spring PQ and TU, and a string RS as shown in fig. If the acceleration of blocks A, B and C is a1,a2 and a3 , respectively, then
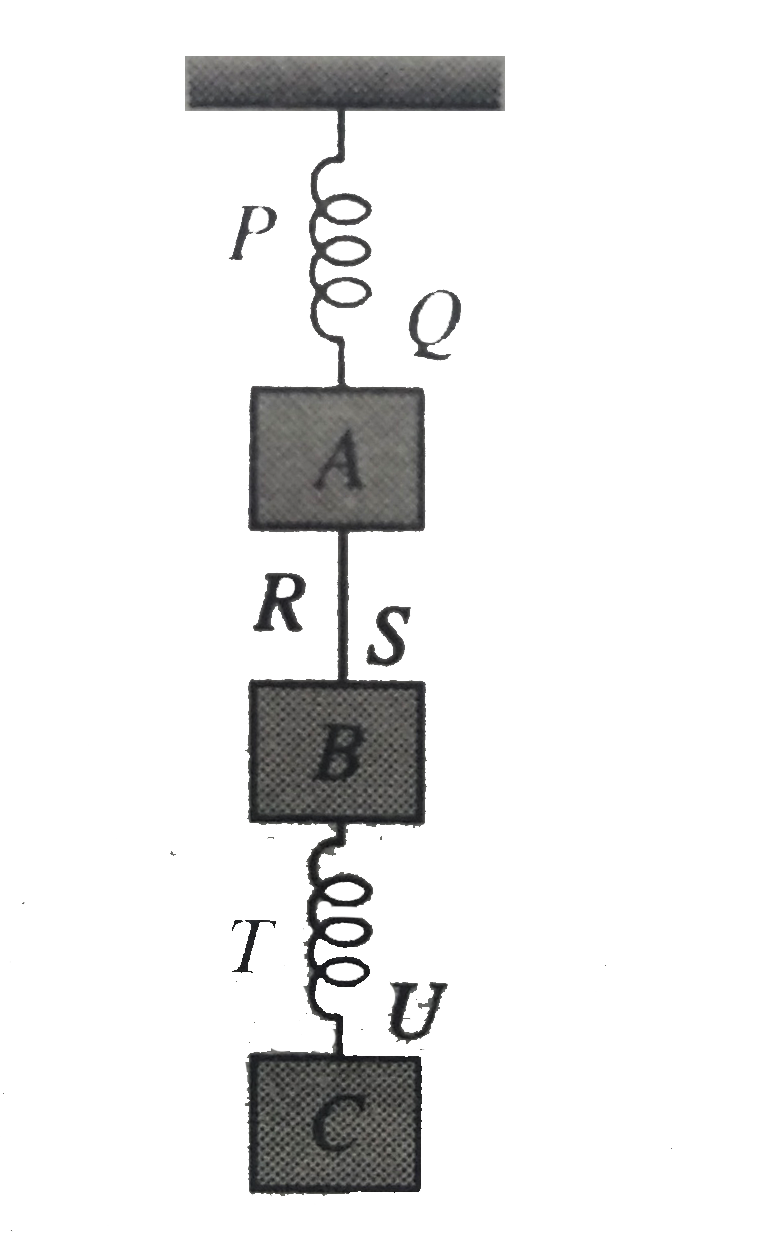
The value of acceleration a1 at the moment string RS is cut isAg, downwardBg, upwardCMore than g, downwardsDZero - Question 2 - Select One
The angular velocities of three bodies in SHM are ω1,ω2,ω3 with their respective amplitudes as A1,A2,A3. If all three bodies have same mass and maximum velocity then
AA1ω1=A2ω2=A3ω3BA1ω21=A2ω22=A3ω23CA21ω1=A22ω2=A22ω2DA21ω21=A22ω22=A23ω23 - Question 3 - Select One

In the arrangement shown above, all surfaces are frictionless. The rod R is constrained to move vertically. The vertically acceleration of R is a1 and the horizontal accelration of the wedge W is a2. The ratio a1/a2 is equal toAtanαBcotαCsinαDcosα
For any three sets A1,A2,A3, let B1=A1,B2=A2−A1 and B3=A3−(A1∪A2), then which one of the following statement is always true
View SolutionIf a1,a2,a3 are the last three digits of 17256 respectively then the value of a2−a1−a3 is equal
View SolutionA1A2A3………A18 is a regular 18 sided polygon. B is an external point such that A1A2B is an equilateral triangle. If A18A1andA1B are adjacent sides of a regular n sided polygon, then n=
View SolutionIf A1,A2,A3 denote respectively the areas of an inscribed polygon of 2n sides , inscribed polygon of n sides and circumscribed poylgon of n sides ,then A1,A2,A3 are in
View SolutionLet a1,a2,a3 be three positive numbers which are in geometric progression with common ratio r. The inequality a3>a2+2a1 holds true if r is equal to
View Solution

