A heavy ball of mass m is tied to a weightless thread of length 1. The friction of the ball against air is proportional to its velocity relative to the air: Flr=μv. A strong horizontal wind is blowing at a constant velocity v. Determine the period T of small oscillations, assuming that the oscillations of the ball attenuate in a time much longer than the period of oscillations.
Step by step video & image solution for A heavy ball of mass m is tied to a weightless thread of length 1. The friction of the ball against air is proportional to its velocity relative to the air: F_(lr) = muv. A strong horizontal wind is blowing at a constant velocity v. Determine the period T of small oscillations, assuming that the oscillations of the ball attenuate in a time much longer than the period of oscillations. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
Two blocks having mass m and 2m and connected by a spring of rigidity k lie on a horizontal plane. Determine the period T of small longitudinal oscillations of the ystem, neglecting friction.
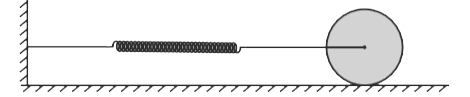
View SolutionA hollow cylindrical shell of radius R has mass M. It is completely filled with ice having mass m. It is placed on a horizontal floor connected to a spring (force constant k) as shown. When it is disturbed it performs oscillations without slipping on the floor.
(a) Find time period of oscillation assuming that the ice is tightly pressed against the inner surface of the cylinder.
(b) If the ice melts into non viscous water, find the time period of oscillations. (Neglect any volume change due to melting of ice)
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One or More
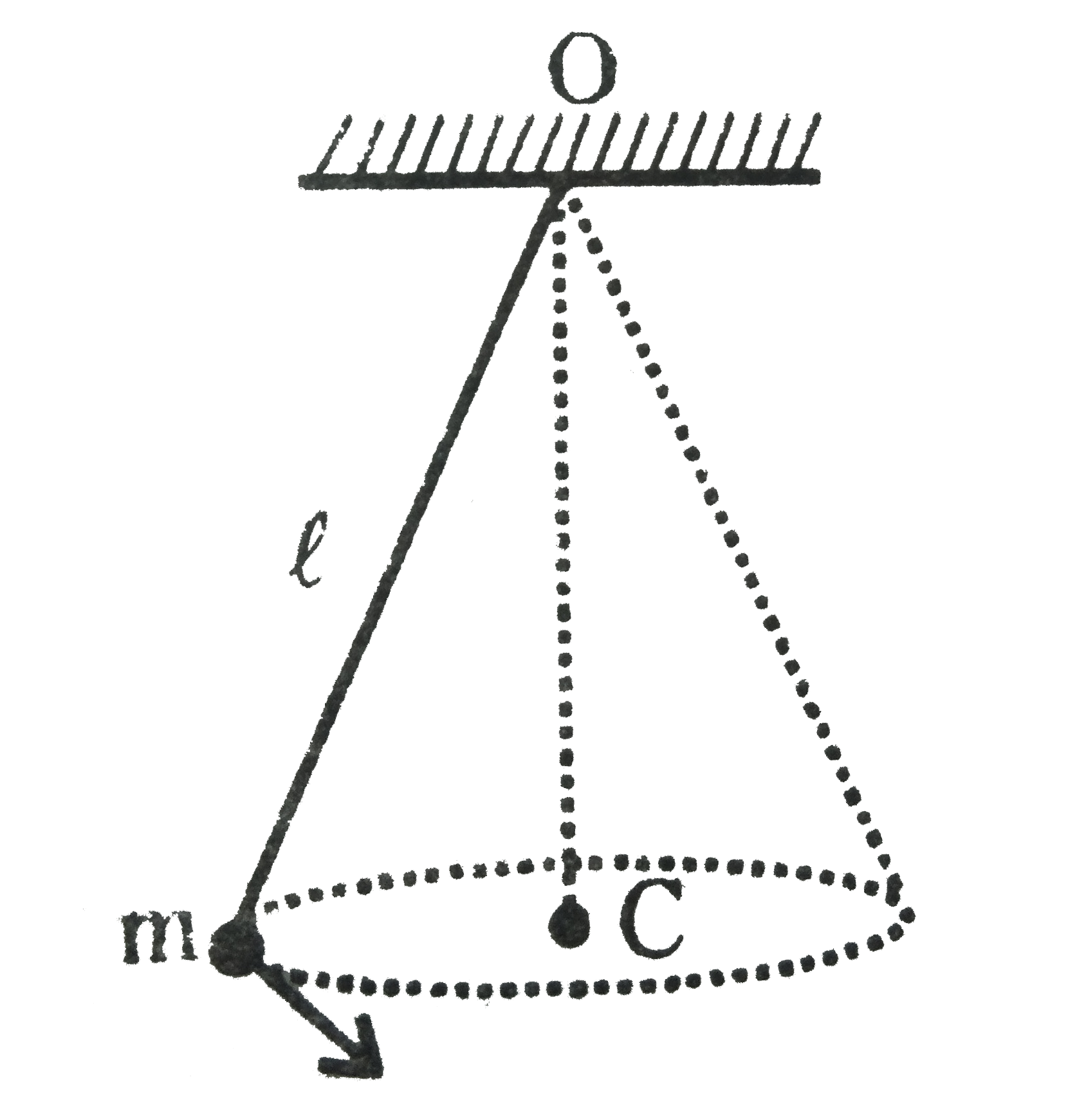
A small ball of mass m suspended from the celling at a point O by a thread of length l moves along a horizontal circle with a constant angular velocity ω.
 .Alinear momentum of system (ball + rod) is conservedBangular momentum about C is conservedCkinetic energy of system (ball + rod) before the collision is equal to kinetic energy of system just after the collisionDlinear momentum of ball is conserved
.Alinear momentum of system (ball + rod) is conservedBangular momentum about C is conservedCkinetic energy of system (ball + rod) before the collision is equal to kinetic energy of system just after the collisionDlinear momentum of ball is conserved - Question 2 - Select One
A solid rubber ball orf density d and radius R falls vertically through air. Assume that the air resistance acting on the ball is F=KRV where K is constant and V is its velocity. Because of this air resistance the ball attains a constant velocity called terminal velocity vT after some time. Then VT
A4πR2dg3KB3K4πR2dgC43πr3dgKDπrdgk
A ball of mass M/2 filled with a gas ( whose mass is M/2) is kept on a frictionless table . A bullet of mass m=M/4 and velocity v0ˆi penetrates the ball , and rests inside at t=0. Assume that the amount of gas emitted during the collision can be neglected. The compressed gas is emitted at a contant velocity v0/2 relative to the ball and at an even rate k ( k is a positive constant ) .
(a) What is the velocity of the ball after the collision with the bullet ?
(b) Find the velocity of the ball v(t) as a function of time. Assume that the emission of gas starts at t=0. What is the final velocity of the ball ?
 View Solution
View SolutionA small ball of mass m suspended from the ceiling at a point O by a thread of length l moves along a horizontal circle with a constant angular velocity ω. Relative to which points does the angular momentum M of the ball remain constant? Find the magnitude of the increment of the vector of the ball's angular momentum relative to the point O picked up during half a revolution.
View SolutionA body of mass m is situated in a potential field U(x) = V0(1−cosax) when U0 and α are constants. Find the time period of small oscillations.
View SolutionA load of mass M is on horizontal rails. A pendulum made of a ball of mass m tied to a weightless inextensible thread is suspended to the load. The load can move only along the rails. Determine the ratio of the periods T2/T1 of small oscillations of the pendulum in vertical planes parallel and perpendicular to the rails.
View SolutionA ball is tied by a weightless inextensible thread to a fixed cylinder of radius r. At the initial moment the thread is wound so that the ball touches the cylinder. Then the ball acquires a velocity v in the raidal direction, and the thread starts unwinding (Fig). Determine the length l of the upwound segment of the thread by the instant of time t, neglecting the force of gravity.
 View Solution
View SolutionA ball of mass m kept at the centre of a string of length L is pulled from centre in perpendicular direction and released. Prove that motion of ball is simple harmonic and determine time period of oscillation
View Solution

