A chain of length L and mass per unit length ρ is piled on a horizontal surface. One end of the chain is lifted vertically with constant velocity by a force P.
P as function of height x of the end above the surface will be ρ(gx+v2)
no energy will loss in this process.
work done by force will be 12ρgL2+ρv2L
loss in energy 12ρgLv2
The correct Answer is:A, C, D
Fext=Vreldmdt+mdvdt
dvdt=0(v is contant)
P=ρxg+ρv2
wP+wg=ΔKE
wP=+L∫0ρxgdx+L∫0(ρdx)v2
wP=ρL2g2+ρLv2
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Subjective
107 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic
70 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
The end of a chain of length L and mass per unit length ρ, which is piled up on a horizontally platform is lifted vertically with a constant velocity u by a variable force F. Find F as a function of height x of the end above platform:
View SolutionA chain of mass m and length L is held at rest on smooth horizontal surface such that a part I of the chain is hanging vertically from the table. If the chain is let go, what is its speed as its end just leaves the horizontal surface?
View Solution
Knowledge Check
A chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B
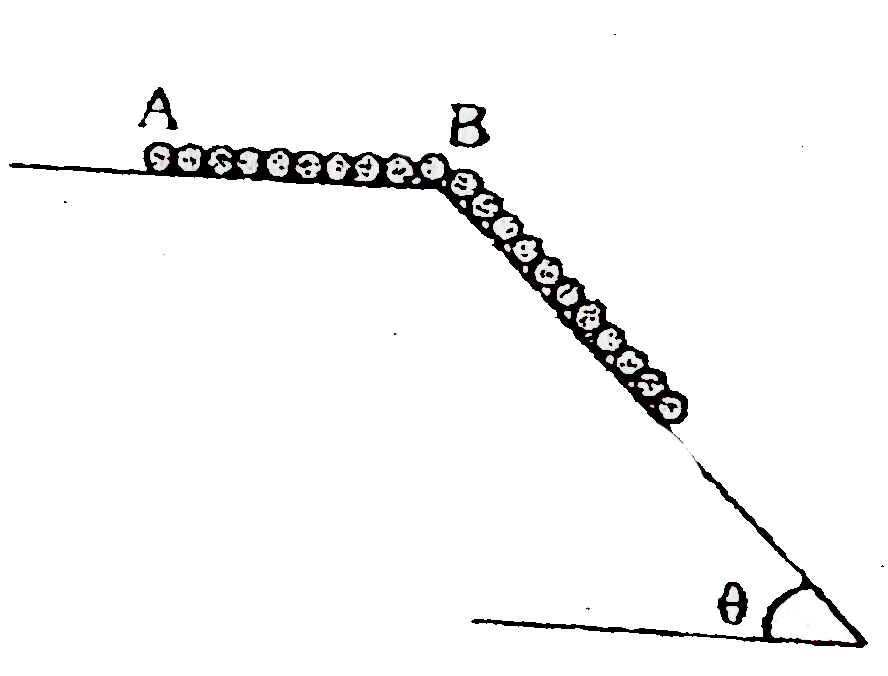 A√2gsinθL(L2−b2)B2√gsinθL(L2−b2)C√gsinθL(L2−b2)D√gsinθ2L(L2−b2)
A√2gsinθL(L2−b2)B2√gsinθL(L2−b2)C√gsinθL(L2−b2)D√gsinθ2L(L2−b2)A uniform flexible chain of mass m and length l hangs in equilibrium over a smooth horizontal pin of neglible diameter. One end of the chain is given a small verticle displacement so that the chain slips over the pin. The speed of chain when it leaves pin is
A√gl2B√glC√2glD√3gl
A chain of length L and mass m is placed upon a smooth surface. The length of BA is (L−b). Calculate the velocity of the chain when its and reaches B

View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
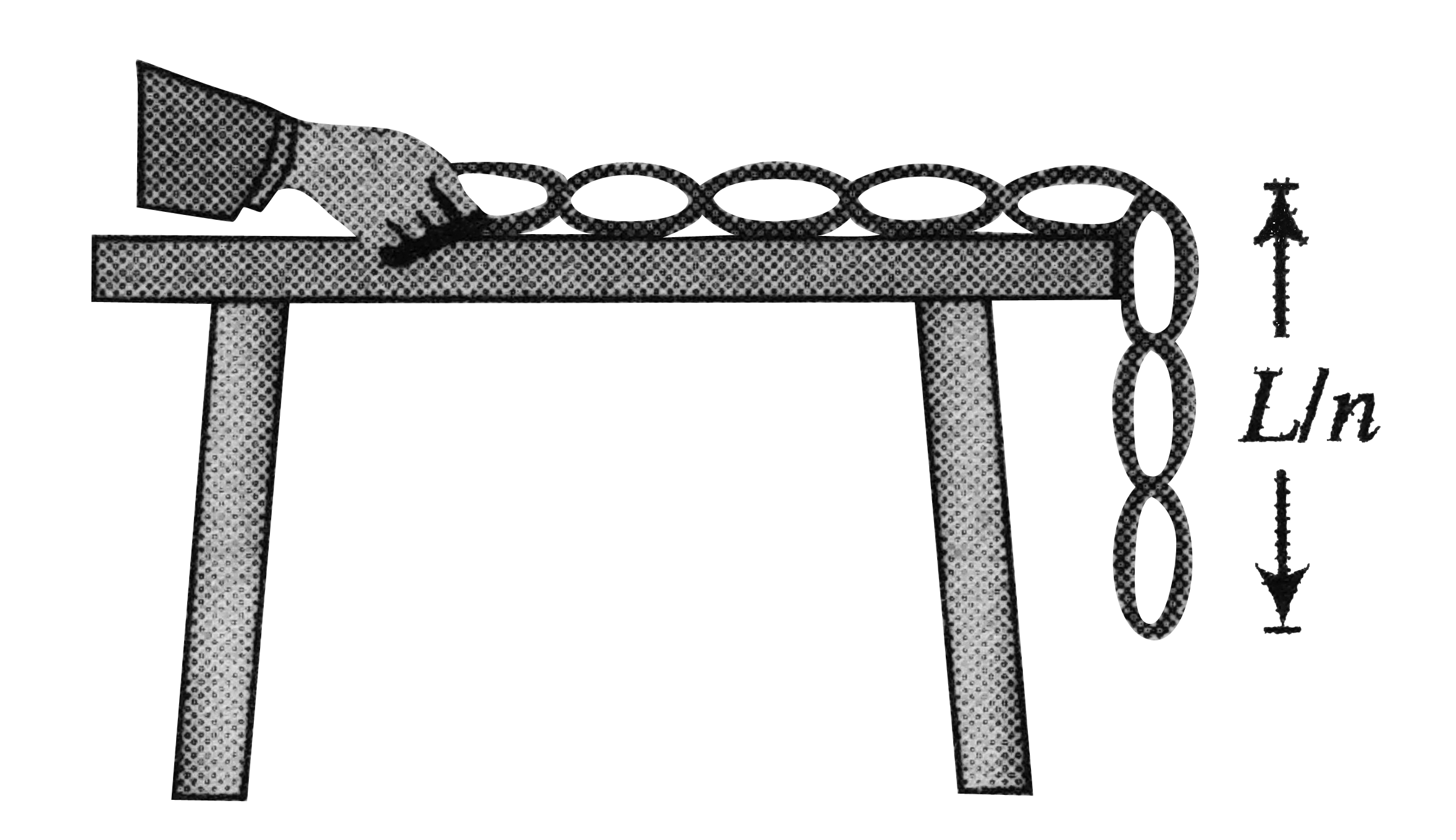
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.

View SolutionA uniform flexible chain of mass m and length l hangs in equilibrium over a smooth horizontal pin of neglible diameter. One end of the chain is given a small verticle displacement so that the chain slips over the pin. The speed of chain when it leaves pin is
View SolutionA chain of length l and mass m lies in a pile on the floor. If its end A is raised vertically at a constant speed v0, express in terms of the length y of chain which is off the floor at any given instant.
(a) The magnitude of the force P applied to end A.
(b) Energy lost during the lifting of the chain.

View SolutionA chain of length l and mass m lies in a pile on the floor. If its end A is raised vertically at a constant speed v0, express in terms of the length y of chain which is off the floor at any given instant.
(a) The magnitude of the force P applied to end A.
(b) Energy lost during the lifting of the chain.

View Solution
