A 10kg solid sphere of radius r=0.8m is rolling without slipping on a horizontal rough surface with 8m/s. The force applied by the right half of the sphere on the left half is 30x. Newton. Find the value of x.
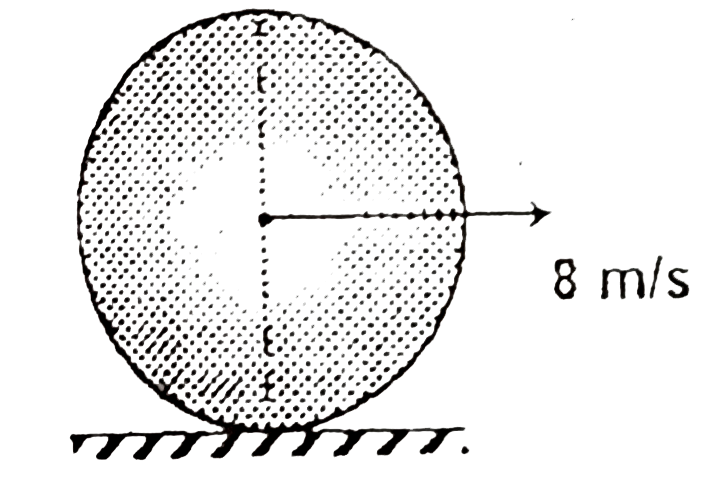
F=(102)(102)×3×0.88
=5×100×0.3=150N

Topper's Solved these Questions
Similar Questions

A solid sphere of mass m and radius R is rolling without slipping as shown in figure. Find angular momentum of the sphere about z-axis.
View SolutionA solid sphere of mass m= 500gm is rolling without slipping on a horizontal surface. Find kinetic energy of a sphere if velocity of centre of mass is 5 cm/sec
View Solution
Knowledge Check
A solid sphere of mass m and radius R rolls without slipping on a horizontal surface such that vc.m.=v0.
AThe kinetic energy of rotation is 15mv20.BThe total kinetic energy is 710mv20.CThe mechanical energy (assume the ground as reference) is mgR+710mv20.DAll options are correct.A solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
A0.014 JB0.028 JC14 JD28 JA solid sphere of mass 1 kg and radius 10 cm rolls without slipping on a horizontal surface, with a velocity of 20 cm/s. The total kinetic energy of the sphere is
A0.014 JB0.028 JC14 JD28 J
A solid sphere of mass 10 kg and diameter 5 cm rolls without slipping on a smooth horizontal surface with velocity 5 cm/s. Its total kinetic energy is
View SolutionA solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?
View SolutionA 1 Kg solid sphere rolls without slipping on a rough horizontal suface as shown in figure. Select incorrect alternative
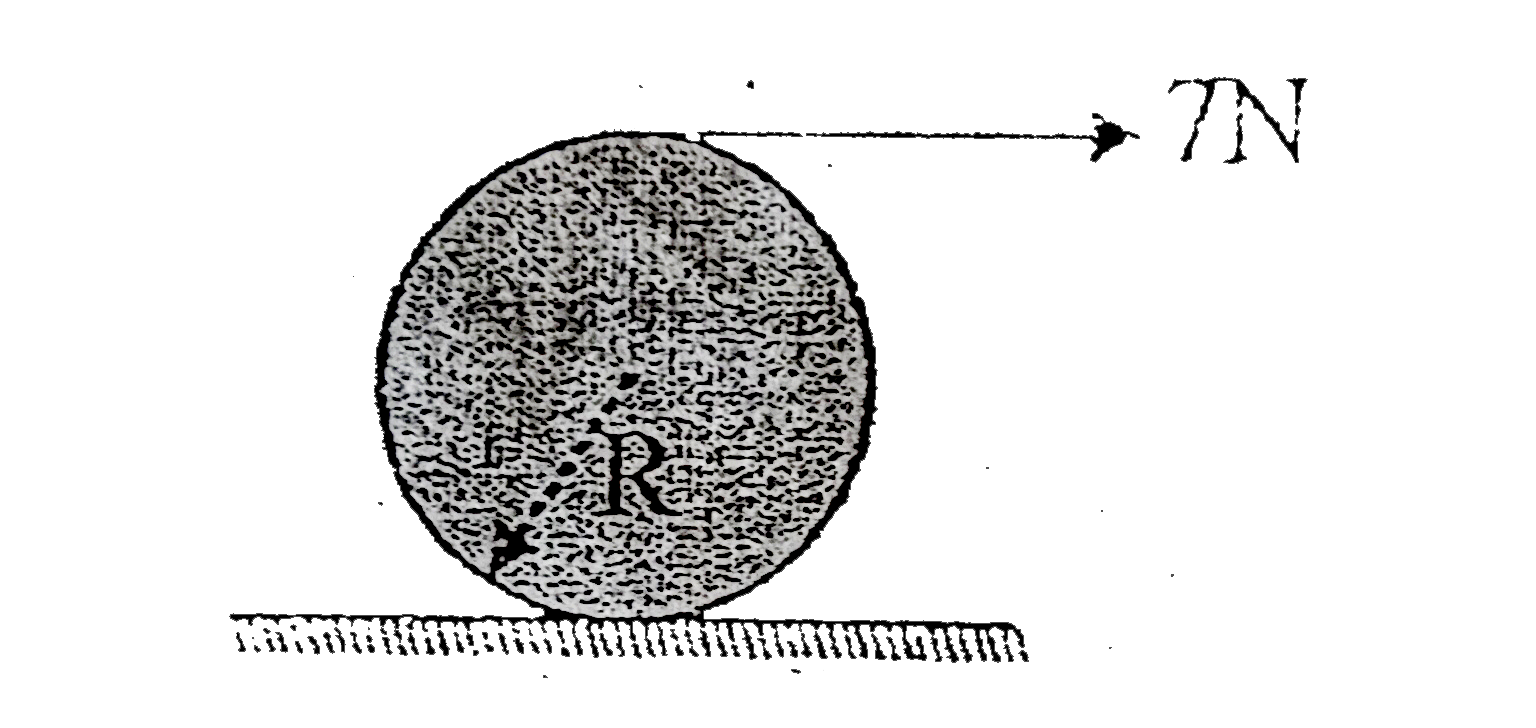
View SolutionA 1kg solid sphere rolls without slipping on a rough horizontal surface under the influence of a 7N force. The force acts tangentially at the highest point of the sphere. Which of the following statements are correct? (7N force acts towards right).
View Solution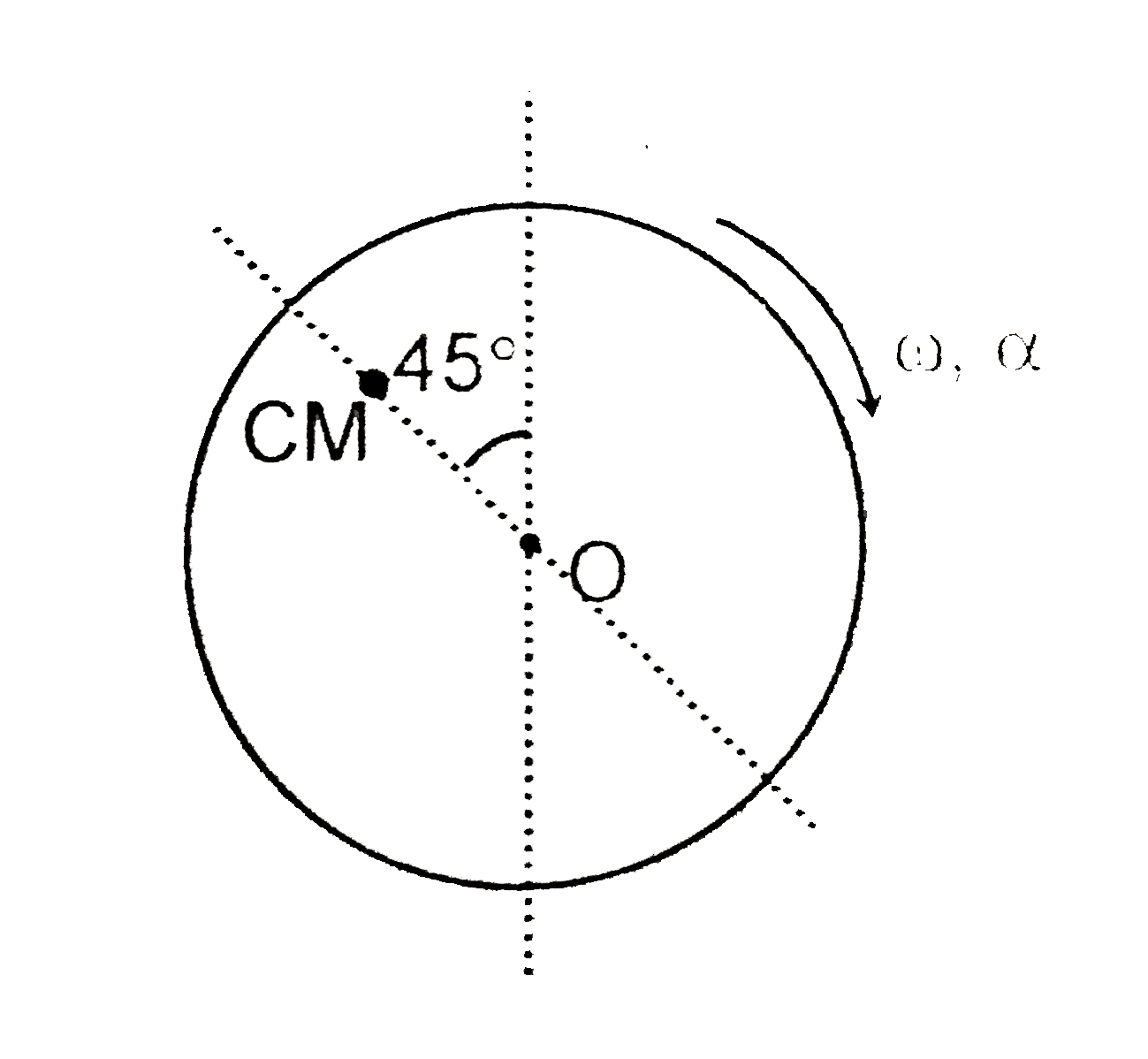
A solid sphere of mass m and radius R is rolling without slipping on a rough horizontal surface with angular acceleration α. Centre of mass of sphere lies at a distance R√2 from the centre of sphere. Find the normal force applied by sphere on the surface at an instant when line joining centre of mass of sphere and centre of sphere makes an angle 45∘ with the vertical and angular velocity of sphere at this instant is ω.
View Solution
