A cube of mass m and density D is suspended from a point P by a spring of stiffness k. The system is kept inside a beaker filled with a liquid of density d. The elongation in the spring, assuming D>d, is :

mgk(1−dD)
mgk(1−Dd)
mgk(1+dD)
None of these
The cube is in equilibrium under the following three forces,
(A) spring force kx, where x= elongation of the spring,
(B) gravitational force w, wieght of the cube =mg
(C) buoyant force Fb (or upward thrust) imparted by the liquid on the cube given as
Fb=Vdg where
V=volume of the immersed portion of the cube. For complete immersion, V= volume of the cube.
For equilibrium of the cube, kx+Fb=mg
⇒x=mg−Fbk=mg−Vdgk, where V=(m/D)
⇒x=mgk(1−dD)

Topper's Solved these Questions
Similar Questions
A cube of mass m and densituy D is suspended from the point P by a spring of stiffness k,
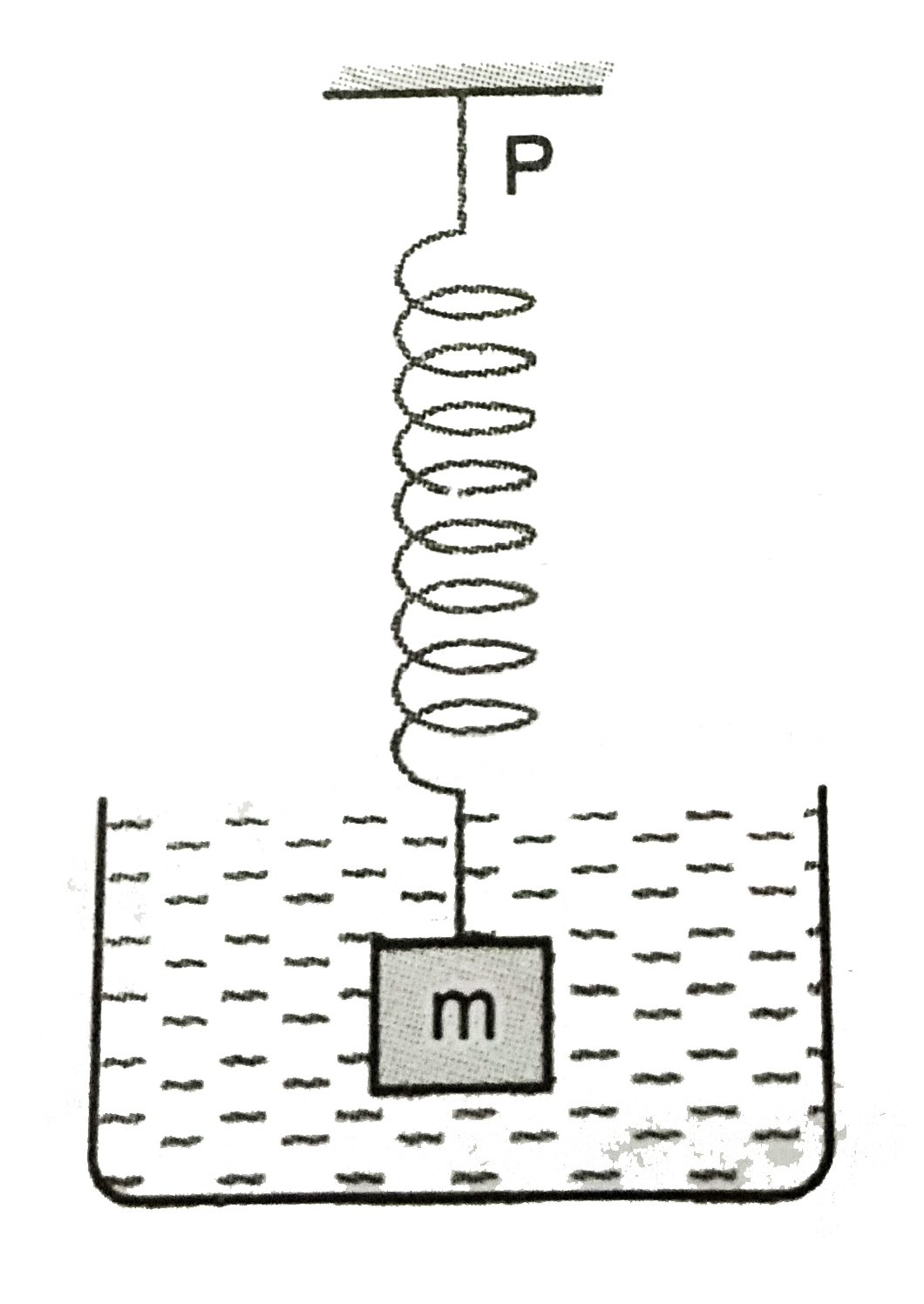
The system is kept inside a beaker filled with a liquid of density d, where D gt d. What is the elongation in the spring ?
View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
View Solution
Knowledge Check
A sphere of density d is let fall in a liquid of density d4. The acceleration of the body will be
Ag4B3g4Cg2DgA disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:
 AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk
AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθkA body of volume V and density d is completely immersed in a liquid of density p. Then the apparent weight of the body will be:
AVdgBVρgCV(ρ−d)gDV(d−ρ)g
A vessel filled with water is kept on a weighing pan and the scale adjusted of zero. A block of mass M and density ρ is suspended by a masselss spring of spring constant k. This block is submerged inside into the water in the vessel. What is the reading of the scale /
View SolutionA block of mass m and relative density y(<1) si attached to an ideal spring of constant K. The system is initially at rest and at equilibrium. If the container acceleration upwards with a0, find the increase in the elongation of the spring in equilibrium.
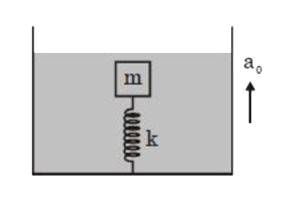
View SolutionA rod mass (M) hinged at (O) is kept in equilibrium with a spring of stiffness (k) as shown in figure. The potential energy stored in the spring is .

View Solution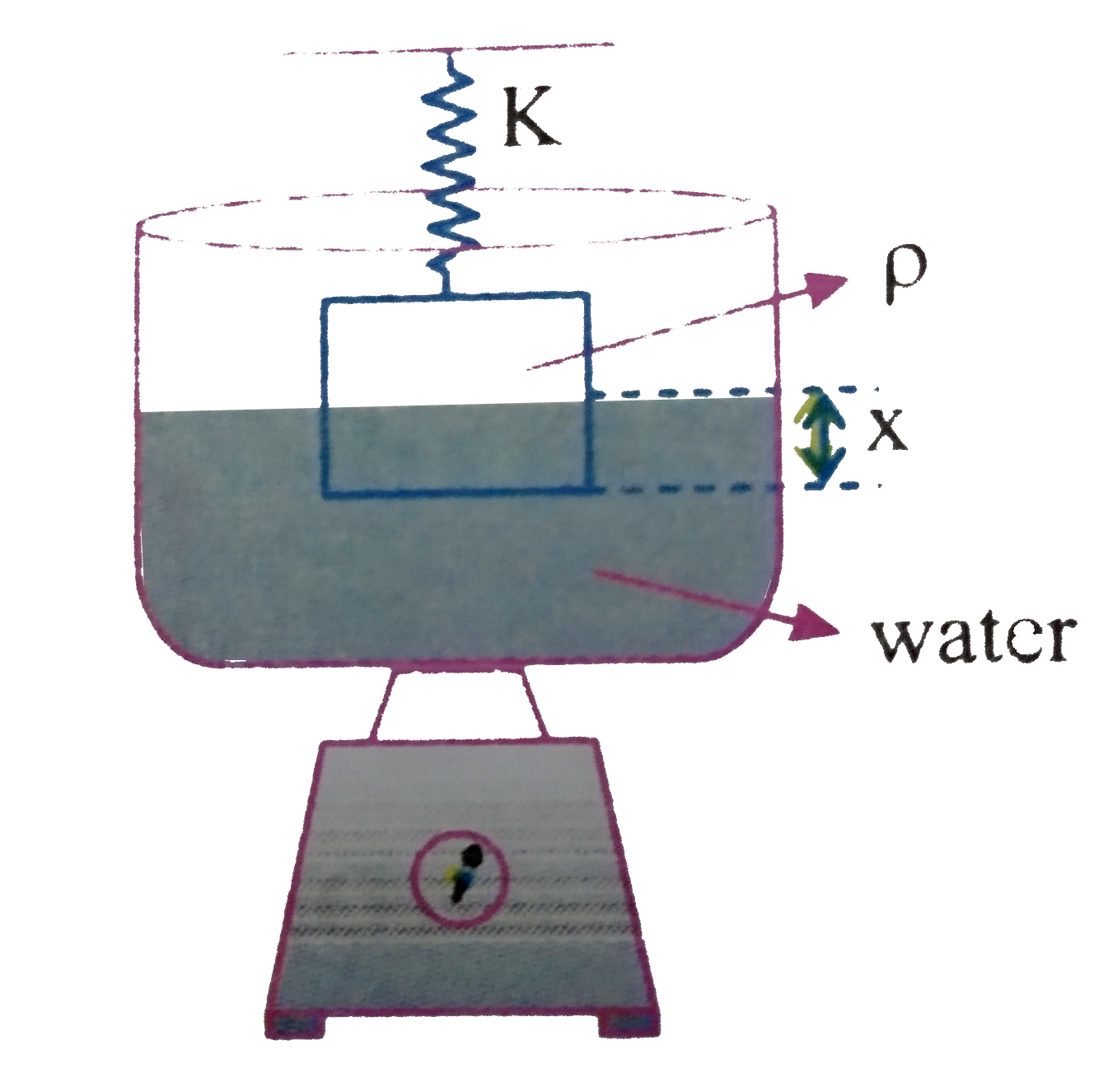
A vessel filled with water is kept on a weighing pan and the scale adjusted to zero. A block of mass 10 kg is suspended by a massless spring of spring constant k=100N/m. This block is submerged inside into the water in the vessel such that elongation i spring is x=10cm. What is the reading of the scale?
View SolutionA mass M is suspended as shown in fig. The system is in equilibrium. Assume pulleys to be massless. K is the force constant of the spring.
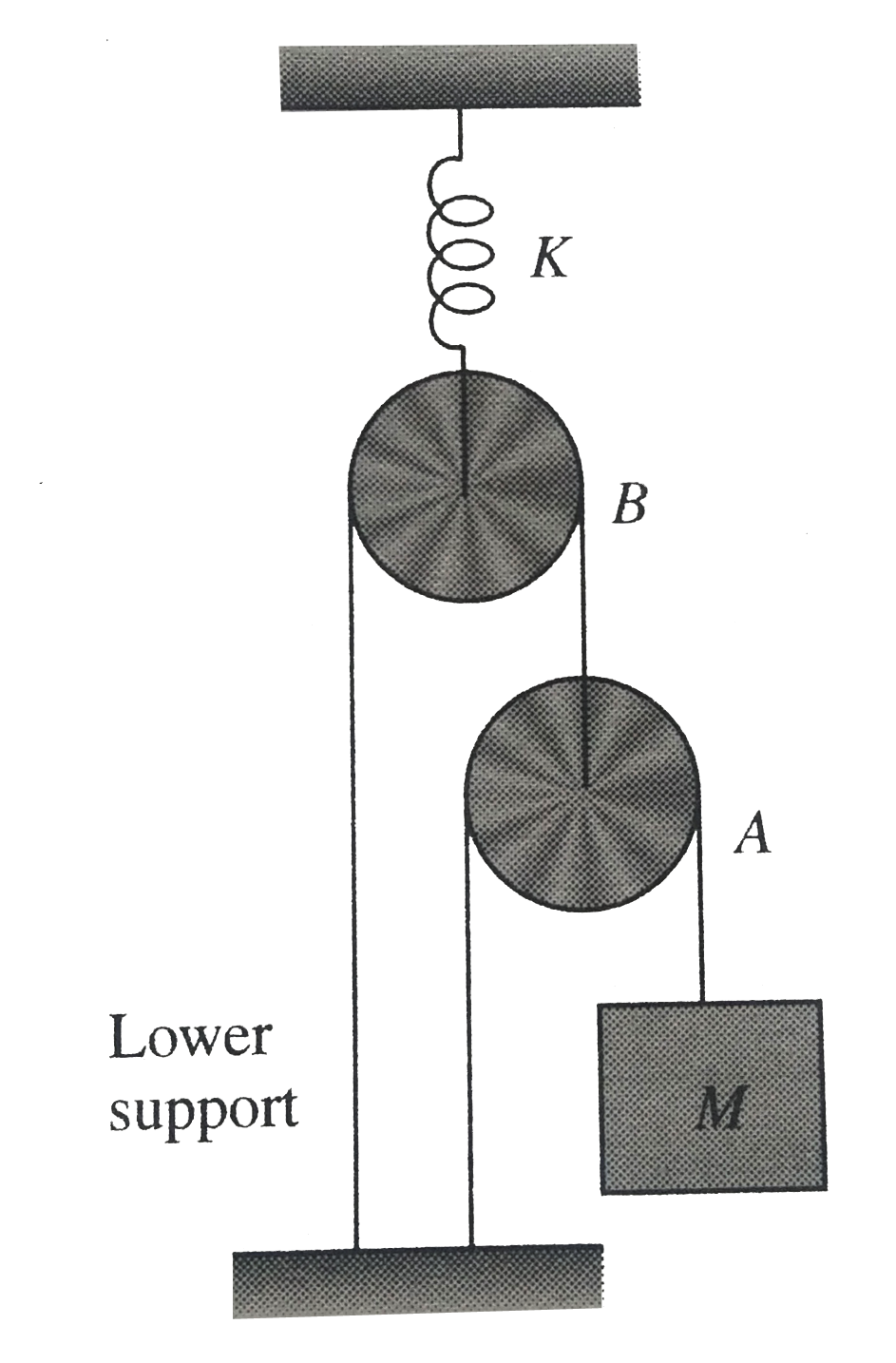
The extension produced in the spring is given by
View Solution
