From a circle of radius a, an isosceles right angles triangle with the hypotenuse as the diameter of the circle is removed. The distance of the centre of mass of the remaining portion from the centre of the circle is
3(π−1)a
(π−1)a6
a3(π−1)
a3(π+1)
The correct Answer is:C
To solve the problem of finding the distance of the center of mass of the remaining portion from the center of the circle after removing an isosceles right triangle with the hypotenuse as the diameter of the circle, we can follow these steps:
1. Understand the Geometry:
- We have a circle with radius a. The diameter of the circle serves as the hypotenuse of the isosceles right triangle.
- The triangle will have its base along the diameter of the circle and its apex at the topmost point of the circle.
2. Determine the Area and Mass of the Circle:
- The area Ac of the circle is given by:
Ac=πa2
- Assuming uniform mass density σ, the mass M of the circle can be expressed as:
M=σAc=σπa2
3. Determine the Area and Mass of the Triangle:
- The base of the triangle is the diameter of the circle, which is 2a, and the height is a.
- The area At of the triangle is:
At=12×base×height=12×2a×a=a2
- The mass Mt of the triangle is:
Mt=σAt=σa2
4. Determine the Center of Mass of the Triangle:
- The center of mass of the isosceles right triangle lies at a distance of 13 of the height from the base. The height of the triangle is a, so the center of mass is located at:
yt=a3
- The coordinates of the center of mass of the triangle (taking the base along the x-axis) are (0,a3).
5. Set Up the Center of Mass Equation:
- The center of mass of the remaining portion (circle minus triangle) can be found using the formula:
ycm=Myc−MtytM−Mt
- Here, yc=0 (the center of the circle), and yt=a3:
ycm=M⋅0−Mt⋅a3M−Mt=−Mt⋅a3M−Mt
6. Substituting Values:
- Substitute M=σπa2 and Mt=σa2:
ycm=−σa2⋅a3σπa2−σa2
- Simplifying gives:
ycm=−σa33σa2(π−1)=−a3(π−1)
7. Distance from the Center:
- The distance of the center of mass of the remaining portion from the center of the circle is:
d=|ycm|=a3(π−1)
Final Answer
The distance of the center of mass of the remaining portion from the center of the circle is:
a3(π−1)
- We have a circle with radius a. The diameter of the circle serves as the hypotenuse of the isosceles right triangle.
- The triangle will have its base along the diameter of the circle and its apex at the topmost point of the circle.
- The area Ac of the circle is given by:
Ac=πa2
- Assuming uniform mass density σ, the mass M of the circle can be expressed as:
M=σAc=σπa2
- The base of the triangle is the diameter of the circle, which is 2a, and the height is a.
- The area At of the triangle is:
At=12×base×height=12×2a×a=a2
- The mass Mt of the triangle is:
Mt=σAt=σa2
- The center of mass of the isosceles right triangle lies at a distance of 13 of the height from the base. The height of the triangle is a, so the center of mass is located at:
yt=a3
- The coordinates of the center of mass of the triangle (taking the base along the x-axis) are (0,a3).
- The center of mass of the remaining portion (circle minus triangle) can be found using the formula:
ycm=Myc−MtytM−Mt
- Here, yc=0 (the center of the circle), and yt=a3:
ycm=M⋅0−Mt⋅a3M−Mt=−Mt⋅a3M−Mt
- Substitute M=σπa2 and Mt=σa2:
ycm=−σa2⋅a3σπa2−σa2
- Simplifying gives:
ycm=−σa33σa2(π−1)=−a3(π−1)
- The distance of the center of mass of the remaining portion from the center of the circle is:
d=|ycm|=a3(π−1)
The distance of the center of mass of the remaining portion from the center of the circle is:
a3(π−1)
Topper's Solved these Questions
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - EXERCISE-2 [PARAGRAPH TYPE]
10 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - EXERCISE-2 [REASONING TYPE]
10 Videos
- CENTRE OF MASS & MOMENTUM CONSERVATIONBOOK - BANSALCHAPTER - CENTRE OF MASS & MOMENTUM CONSERVATIONEXERCISE - Practice Exercise
20 Videos
- GRAVITATIONBOOK - BANSALCHAPTER - GRAVITATIONEXERCISE - EXERCISE -4 Section - B
6 Videos
Similar Questions
A square hole is punched out of a circular lamina, the diagonal of the square being the radius of the circle. If 'a' be the diameter of the sircle, find the distance of centre of mass of the remainder from the centre of the circle.
View SolutionFrom a uniform circular disc of radius R, a circular disc of radius R/6 and having centre at a distance R/2 from the centre of the disc is removed. Determine the centre of mass of remaining portion of the disc.
View Solution
Knowledge Check
A uniform sphere has radius R. A sphere of diameter R is cut from its edge as shown. Then the distance of centre of mass of remaining portion from the centre of mass of the original sphere is
 AR/7BR/14C2R/7DR/18
AR/7BR/14C2R/7DR/18From a point Q, the length of the tangent to a circle is 12 cm and the distance of Q from the centre is 13 cm. The radius of the circle is 13 cm. The radius of the circle is :
A12 cmB13 cmC5 cmDNone of theseA square hole is punched out of a circular lamina of diameter a. The diagonal of the square is equal to the radius of the circle and one of the corners is at the centre of the circle . The distance of the C.G. of the remainder from the centre of circle is
Aa8π+4Ba8π−4Ca5π−4Da5π+4
If a circle is inscribed in right angled triangle ABC with right angle at B, show that the diameter of the circle is equal to AB+BC−AC.
View SolutionA diameter of a circle is a chord that………the centre.
View SolutionAD is the diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is
View SolutionIf the distance of point P from the centre of the circle is 13 cm and the radius of the circle is 5 cm, then length of the tangent drawn from P to the circle is :
View SolutionA uniform circular disc of radius a is taken. A circular portion of radius b has been removed from it as shown in the figure. If the center of hole is at a distance c from the center of the disc, the distance x2 of the center of mass of the remaining part from the initial center of mass O is given by
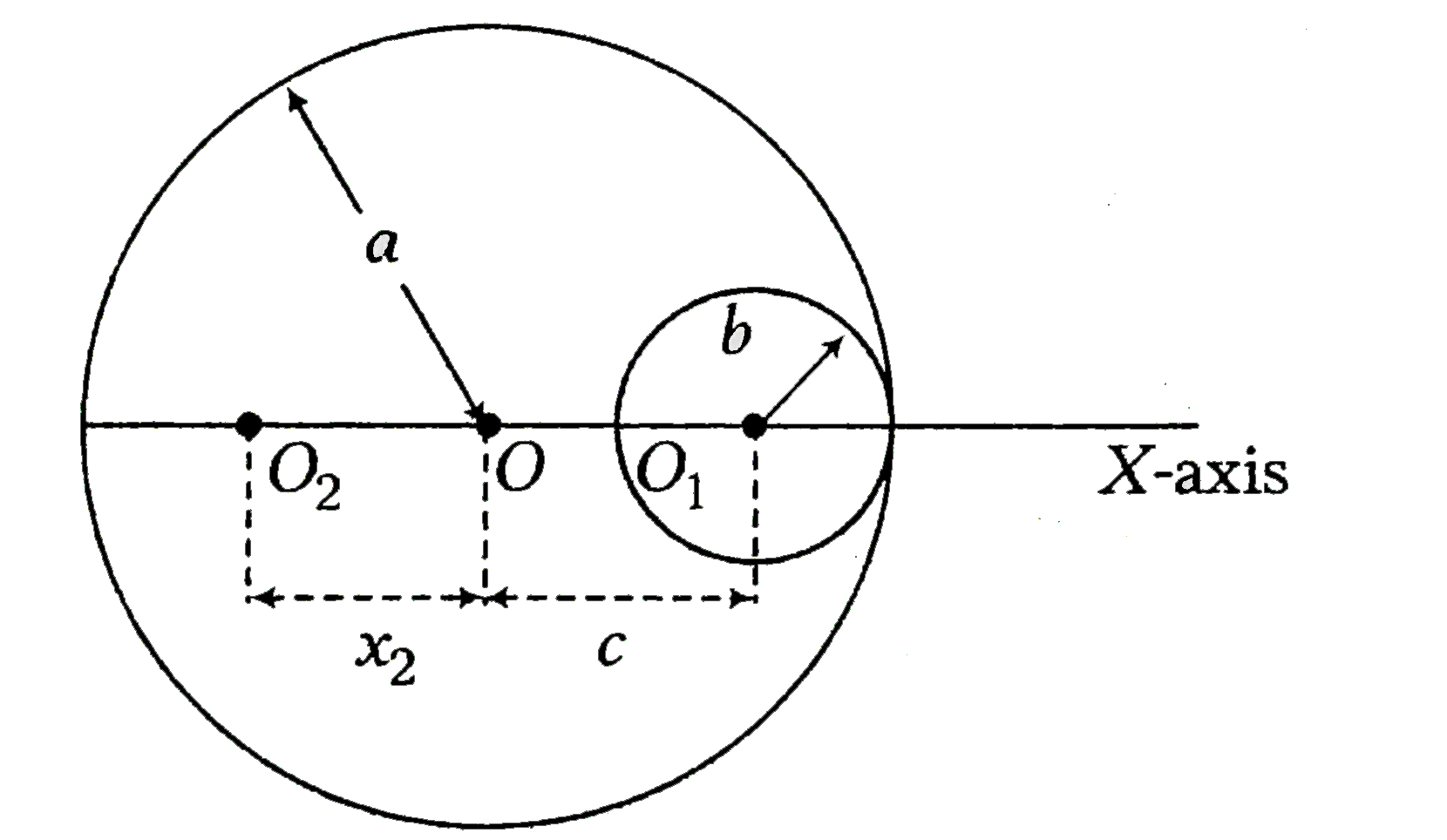
View Solution
