A slab consists of two layers of different materials of the same thickness and having thermal conductivities $K_{1}$ and $K_{2}$ . The equivalent thermal conductivity of the slab is

A

$K_{1} + K_{2}$

B

$\frac{2 K_{1} K_{2}}{K_{1} + K_{2}}$

C

$\frac{K_{1} + K_{2}}{K_{1} K_{2}}$

D

$K_{1} K_{2}$

Video Solution

Text Solution

Verified by Experts

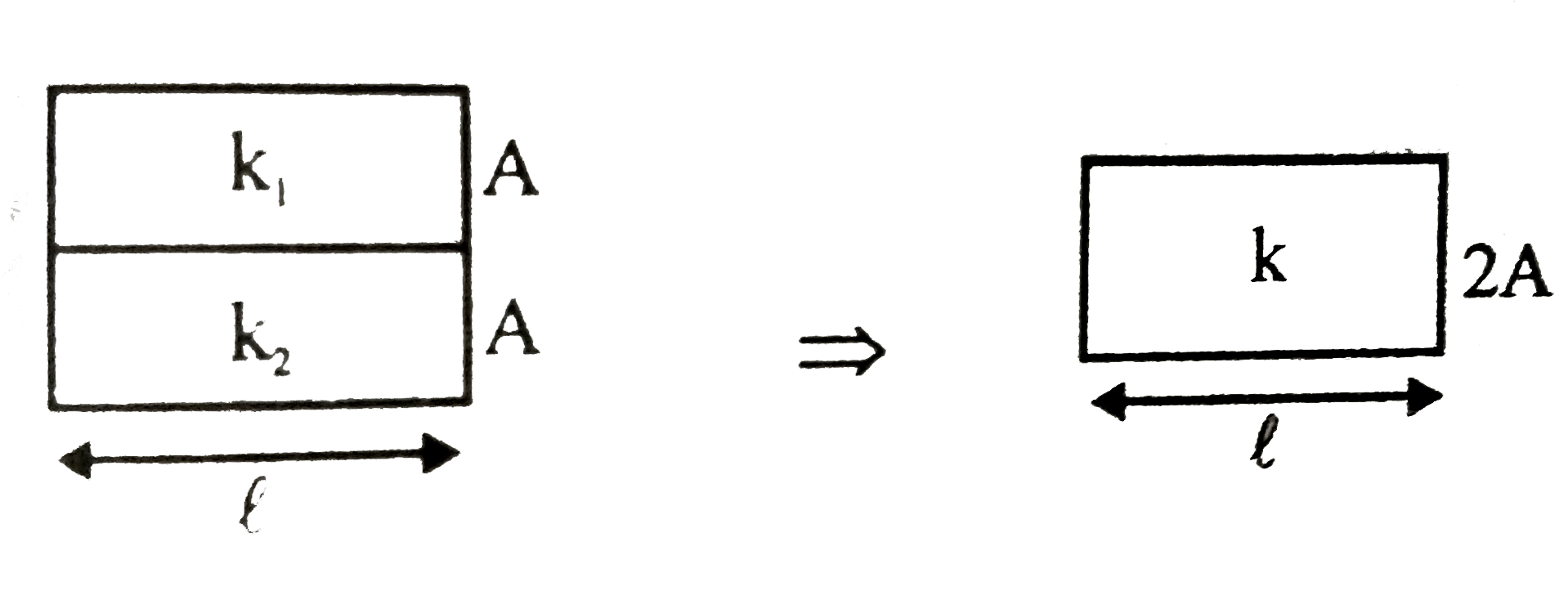
in parallel
$R_{e q} = \frac{R_{1} R_{2}}{R_{1} + R_{2}}$
$R_{1} = \frac{l}{k_{1} A} R_{2} = \frac{l}{k_{2} A}$
$\Rightarrow R_{e q} = \frac{(\frac{l}{A}) \cdot (\frac{l}{A}) (\frac{1}{k_{1} k_{2}})}{\frac{l}{A} (\frac{1}{k_{1} + k_{2}})}$
$\Rightarrow R_{e q} = \frac{l}{A} (\frac{1}{k_{1} + k_{2}})$
$k = \frac{k_{1} + k_{2}}{2}$

|

Updated on:21/07/2023

Class 11 PHYSICS THERMAL EXPANSION AND CALORIMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A slab consists of two parallel layers of two different materials of same thickness having thermal conductivities $K_{1} and K_{2}$ . The equivalent conductivity of the combination is
A $K_{1} + K_{2}$
B $\frac{K_{1} + K_{2}}{2}$
C $\frac{2 K_{1} K_{2}}{K_{1} + K_{2}}$
D $\frac{K_{1} + K_{2}}{2 K_{1} K_{2}}$
Question 2 - Select One
Consider a compound slab consisting of two different material having equal thickness and thermal conductivities $K$ and $2 K$ respectively. The equivalent thermal conductivity of the slab is
A $\sqrt{2 K}$
B $3 K$
C $\frac{4}{3} K$
D $\frac{2}{3} K$
Question 3 - Select One
Consider a compound slab consisting of two different material having equal thickness and thermal conductivities $K$ and $2 K$ respectively. The equivalent thermal conductivity of the slab is
A $\sqrt{2}$
B3 K
C $\frac{4}{3} K$
D $\frac{2}{3} K$

BANSAL-HEAT TRANSFER-Exercise

Home
Ask Doubt
Profile