Particles of masses 1g, 28, 3g ..., 100g are kept at the marks 1 cm, 2 cm, 3 cm ... , 100 cm respectively on a meter scale. Find the moment of inertia of the system of particles about a perpendicular bisector of the meter scale.
Given Masses of 1g, 28, 3g ....... 100 g are 1, 2, 3 ...... 100 cm on a scale.

i) Sum of masses Σm=nnii=1
Sum of n natural numbers S
=n(n+1)2=100×1012=5051
∴ Total mass M = 5051 gr = 5.051 kg →(1)
ii) Centre of mass of all these masses is given by
XCM=m1x1+m2x2+.....Σm
1×1+2×2+3×3+........+100×100M
= 12+22+32+......+1002M
But sum of squares of 1st n natural numbers is S=n(n+1)(2n+1)6
∴CM=n(n+1)(2n+1)6×n(n+1)use M=n(n+1)2
∴CM=2012=67CMto (2)<br>iii)M.O.I=m1r21+m2r22+m3r23+......+m100r2100
therefoer1=1×1×1+2×22+3×32+.......+100×1002
=1+23+33+......+1003×10−7kgm2
(∵1g=10−3kg&1.CM=10−2m)
Sum of cubes of 1st n natural numbers is
S=n2(n+1)24
∴I=1002×(101)24g.cm2
=2.550×107g.cm2=2.550kg.m2 (From one end of scale)
M.O.I about C.M = IG−I−MR2
=2.550−5.05×0.67×0.67
=2.550−2.267=0.238kgm2
iv) Perpendicular bisector is at 50 CM.
so shift M.O.I from centre of mass to x1 = 50cm point from x = 67 CM
therefoer Distance between the axis
R=67−50=17cm=0.17M
M.O.I about this axis I=IG+MR2
=0.283+5.05×0.17×0.17
=0.283+0.146=0.429kgm2
∴ M.O.I about perpendicular bisector of scale = 0.429kg−m2
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - LONG ANSWER QUESTIONS
5 Videos
- SOUNDBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - SOUNDEXERCISE - WORKED OUT EXAMPLES
2 Videos
- THERMODYNAMICSBOOK - VGS PUBLICATION-BRILLIANTCHAPTER - THERMODYNAMICSEXERCISE - LONG ANSWER QUESTIONS
4 Videos
Similar Questions
Particles of masses 1g, 2g, 3g ….100g are kept at the marks 1cm, 2cm, 3cm …., 100 cm respectively on a metre scale. Find the moment of inertia of the system of particles about a perpendicular bisector of the metre scale.
View SolutionParticles of masses 1g, 2g, 3g ….100g are kept at the marks 1cm, 2cm, 3cm …., 100 cm respectively on a metre scale. Find the moment of inertia of the system of particles about a perpendicular besector of the metre scale.
View Solution
Knowledge Check
Two masses of 500 gram and 600 grams are attached to the 10 cm and 80 cm marks respectively of a light metre scale. The moment of inertia of this system about an axis passing through the centre of the scale will be
A0.134 kg-m2B2 kg-m2C0.56 kg-m2D4.5 kg-m2Weights of 1g, 2g,...., 100g are suspended from the 1-cm, 2-cm,......, 100-cm marks respectively of a light meter scale. Where should it be supported for the system to be in equilibrium ?
A55-cm markB60-cm markC66-cm markD72-cm markParticles of masses 1g, 2g, 3g, ……. 100g re kept at the marks 1 cm, 2cm, 3cm,………. 100 cm, respectively on a metre scale. Find the moment of inertia of the system of particle about a perpendicular bisector of the metre scale.
A55 cm markB60 cm markC66 cm markD72 cm mark
Particles of masses 1g, 2g, 3g, ……. 100g re kept at the marks 1 cm, 2cm, 3cm,………. 100 cm, respectively on a metre scale. Find the moment of inertia of the system of particle about a perpendicular bisector of the metre scale.
View SolutionTwo masses of 3kg and 5kg are placed at 20 cm and 70cm marks respectively on a light wooden meter scale. The M.I. of the system about an axis passign through 100cm mark and perpendicular to the meter scale is
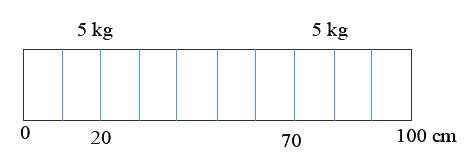
View SolutionTwo masses of 3kg and 5kg are placed at 20 cm and 70cm marks respectively on a light wooden meter scale. The M.I. of the system about an axis passign through 100cm mark and perpendicular to the meter scale is

View SolutionTwo masses of 3kg and 5kg are placed at 20cm and 70cm marks respectively on a light wooden meter scale. The M.I. of the system about an axis passing through 100 cm mark and perpendicular to the meter scale is
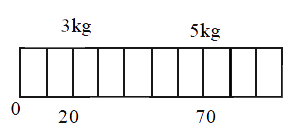
View SolutionThree particles of masses 1gm,2gm&3gm are at 1cm,2cm&3cm from the axis of rotation respectively then the moment of inertia of the system & radius of gyration of the system respectively are …. gmcm2 and ….. cm
View Solution
