A uniform surface charge of density σ is given to a quarter of a disc extending up to infinity in the first quadrant of x−y plane. The centre of the disc is at the origin O. Find the z-component of the electric field at the point (0,0,z) and the potential difference between the point (0,0,d) and (0,0,2d)
The correct Answer is:σ8ε0
Step by step video, text & image solution for A uniform surface charge of density sigma is given to a quarter of a disc extending up to infinity in the first quadrant of x-y plane. The centre of the disc is at the origin O. Find the z-component of the electric field at the point (0,0,z) and the potential difference between the point (0,0,d) and (0,0,2d) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.320videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.415videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.114videos
ELECTRIC POTENTIAL
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s38videos
ELECTRIC FLUX AND GAUSS LAW
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q2videos
ELECTRICAL MEASURING INSTRUMENTS
Similar Questions
A uniform surface charge of density σ is given to a quarter of a disc extending upto infinity in the first quadrant of x-y plane. The centre of the disc is at the origin O. Find the z-component of the electric field at the point (0,0,z) and the potential difference between the points (0,0,d) & (0,0,2d).
 View Solution
View SolutionA unifrom surcace charfge of denstity σ is given to quarter infinite non conducting place in fist quardrant of x−y plane . Find the z-compounct of the elctric field at the point (0, 0, x) Hence or otherwise find the potential difference between the points (0, 0, d) and (0, 0, 2d)
 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
The surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc is σ2∈o. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc reduces by
Areduces by 70.7%Breduces by 29.3%Creduces by 9.7%Dreduces by 14.6% - Question 2 - Select One
A circular ring of radius R and uniform linear charge density +λC/m are kept in x - y plane with its centre at the origin. The electric field at a point (0,0,R√2) is
Aλ3√3ε0RB2λ3√3ε0R2C23√3λε0RDnone the these - Question 3 - Select One
The electric potential at any point x,yanz in metres is given by V=3x2. The electric field at a point (2,0,1) is
A12 Vm−1B−6 Vm−1C6 Vm−1D−12 Vm−1
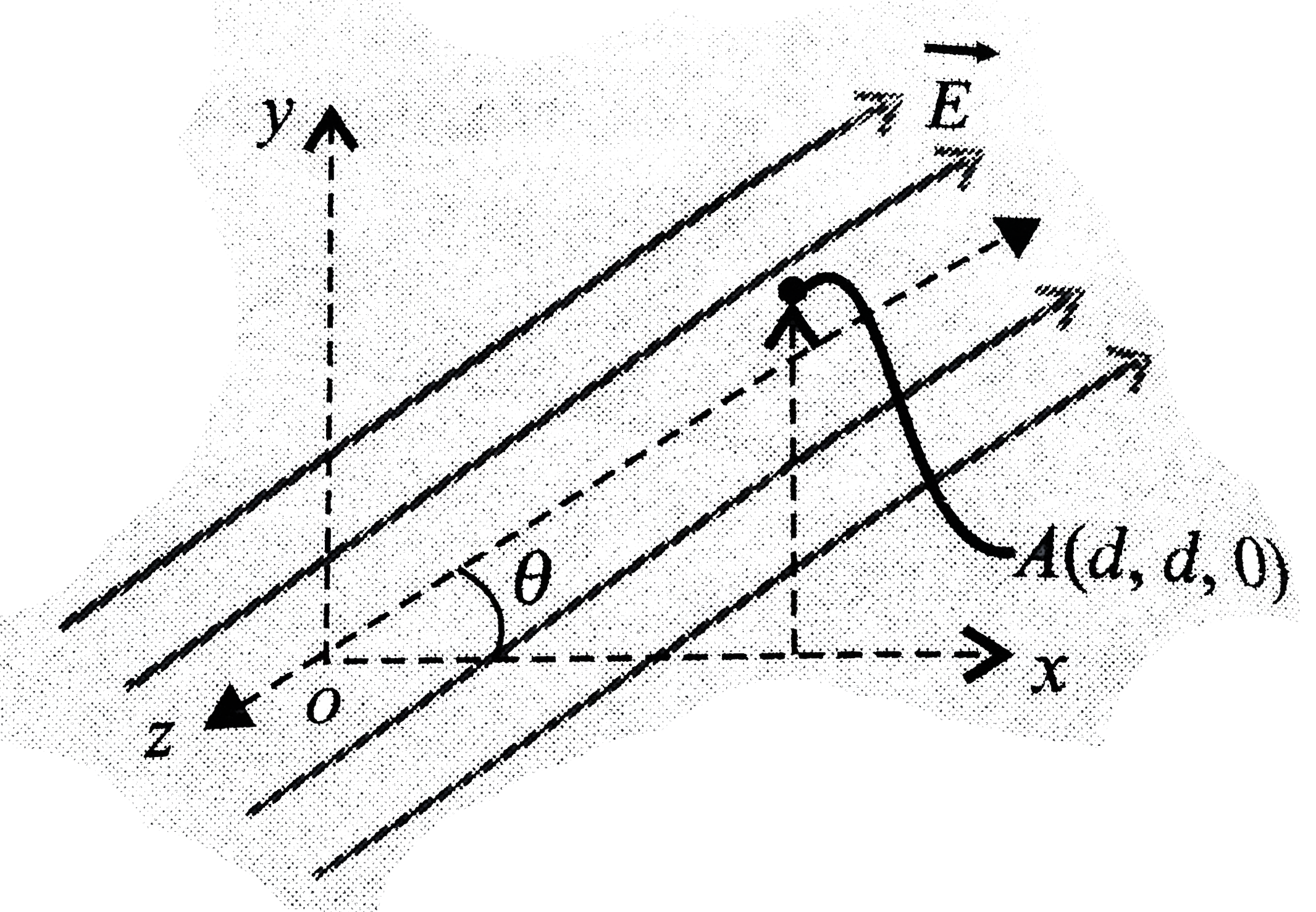
A uniform electric field exists in x y plane as shown in Fig. 3.45. Find the potential difference. Between origin O and A(d,d,0).
 .View Solution
.View SolutionA circular ring lying in the x-y plane with its centre at the origin carries a uniformly distributed positive charge. The variation of the electric field E at the point (0,0,z) is correctly represented by the graph is
View Solution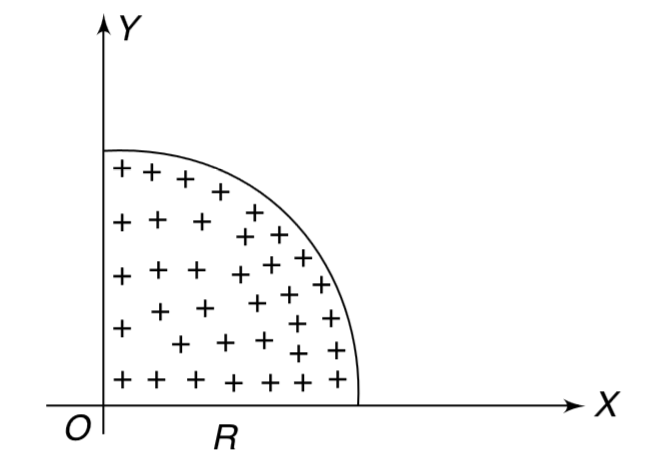
The quarter disc of radius R (see figure) has a uniform surface charge density σ.
(a) Find electric potential at a point (O,O,Z)
(b). Find the Z component of electrif field at (O,O,Z)View SolutionA circular coil with 100 turns and radius 20 cm is kept in Y-Z plane with its centre at the origin. Find the magnetic field at point (20 cm, 0, 0) if coil carries a current of2.0 A.
View SolutionThe electric potential at a point (x, y, z) is given by V=−xy+z−z3yx . The electric field →E at the point (1,0,1) is (all quantities are in SI units)
View Solution

