A particle at angular position θ0 inside a fixed smooth hemispherical bowl of radius r is projected horizontally with velocity v0. Calculate its value so that the particle may rise to the top.
The correct Answer is:v0=√2gr/cosθ0
Step by step video, text & image solution for A particle at angular position theta_(0) inside a fixed smooth hemispherical bowl of radius r is projected horizontally with velocity v_(0). Calculate its value so that the particle may rise to the top. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - NN GHOSHCHAPTER - ROTATIONAL DYNAMICS, MOMENT OF INERTIAEXERCISE - Exercises42videos
ROTATIONAL DYNAMICS, MOMENT OF INERTIA
- BOOK - NN GHOSHCHAPTER - MOMENT, TORQUE, EQUILIBRIUM OF BODIESEXERCISE - Exercises33videos
MOMENT, TORQUE, EQUILIBRIUM OF BODIES
- BOOK - NN GHOSHCHAPTER - SATURATED AND UNSATURATED VAPOUREXERCISE - All Questions1videos
SATURATED AND UNSATURATED VAPOUR
Similar Questions
A particle rests on the top of a smooth hemisphere of radius r. It is imparted a horizontal velocity of √ηgr. Find the angle made by the radius vector joining the particle with the vertical at the instant the particle losses contact with the sphere.
 View Solution
View SolutionA small particle is placed at the top point A of a fixed smooth hemisphere of radius R. Particle is given small displacement towards right and it starts slipping. Calculate velocity of the particle after hitting horizontal perfectly inelastic surface.
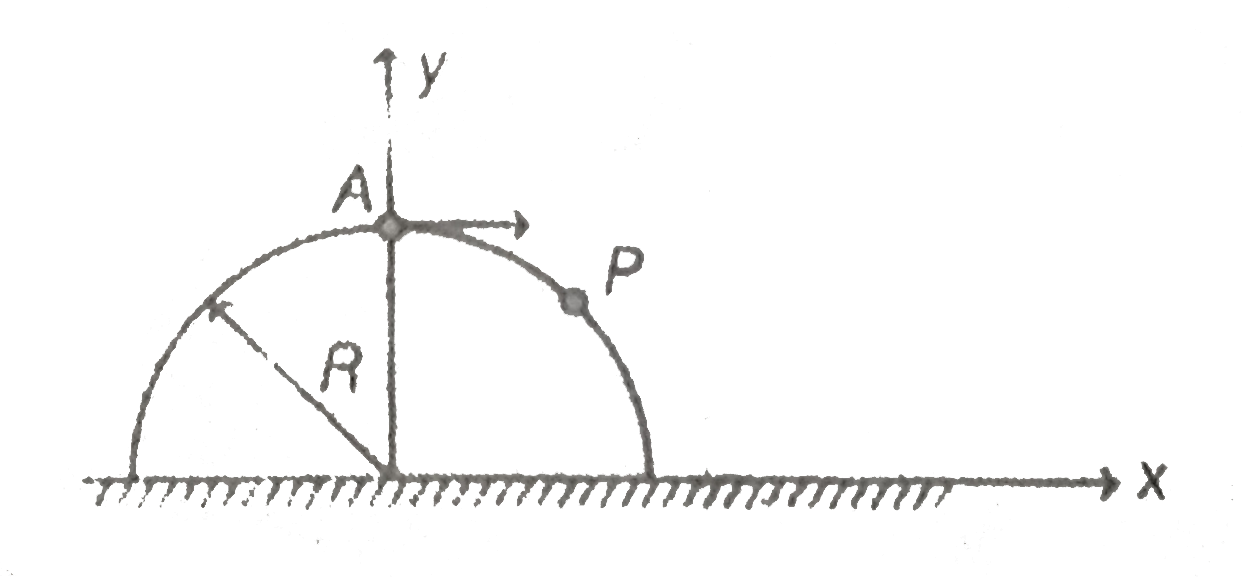 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass m initially at rest starts moving from point A on the surface of a fixed smooth hemisphere of radius r as shown. The particle looses its contact with hemisphere at point B.C is centre of the hemisphere. The equation relating θ and θ' is
 .A3sinθ=2cosθ'B2sinθ=3cosθ'C3sinθ'=2cosθD2sinθ=3cosθ'
.A3sinθ=2cosθ'B2sinθ=3cosθ'C3sinθ'=2cosθD2sinθ=3cosθ' - Question 2 - Select One or More
A horizontal plane supports a fixed vertical cylinder of radius R and a particle is attached to the cylinder by a horizontal thread AB as shown in Fig. The particle initially rest on a horizontal plane. A horizontal velocity v0 is imparted to the particle, normal to the threading during subsequent motion. Point out the false statements:
 AAngular momentum of particle about O remains constant.BAngular momentum about B remains constantCMomentum and kinetic energy both remain constant.DKinetic energy remains constant
AAngular momentum of particle about O remains constant.BAngular momentum about B remains constantCMomentum and kinetic energy both remain constant.DKinetic energy remains constant - Question 3 - Select One or More
A small block of mass m is released from rest from position A inside a smooth hemispherical bowl of radius R as shown in figure Choose the wrong option.
 AAcceleration of block is constant throughoutBAcceleration of block is g at ACAcceleration of block is 3g at BDAcceleration of block is 2g at B
AAcceleration of block is constant throughoutBAcceleration of block is g at ACAcceleration of block is 3g at BDAcceleration of block is 2g at B
A particle of mass m is released from the top of a smooth hemisphere of radius R with the horizontal speed μ. Calculate the angle with verticle where it loses contact with the hemisphere.
View SolutionA small mass particle is projected with an initial velocity v0 tangent to the horizontal rim of smooth hemispherical bowl at a radius r0 from the vertical centre line, as shown at point A. As the particle slide past point B, a distance h below A and distance r from the vertical centre line, its velocity v makes an angle θ with the horizontal tangent to the bowl through B. Determine θ.
 View Solution
View SolutionA particle moving on the inside of a smooth sphere of radius r describing a horizontal circle at a distance r/2 below the centre of the sphere. What is its speed ?
View SolutionA particle is given an initial speed u inside a smooth spherical shell of radius R so that it is just able to complete the circle. Acceleration of the particle, when its velocity is vertical, is
 View Solution
View SolutionWhen a particle is projected from the surface of earth its mechanical energy and angular momentum about center of earth at all time are constant.
Q. A particle of mass m is projected from the surface of earth with velocity v0 at angle 0 with horizontal suppose h be the maximum height of particle from surface of earth and v its speed at that point then v isv0cos0View Solution

