A massive black hole of mass m and radius R is spinning with angular velocity ω. The power P radiated by it as gravitational waves is given by P=Gc–5mxRyωz, where c and G are speed of light in free space, and the universal gravitational constant , respectively. Then
x =–1, y = 2, z = 4
x = 1, y = 1, z = 4
x = –1, y = 4, z = 4
x = 2, y = 4, z = 6
The correct Answer is:D
Step by step video, text & image solution for A massive black hole of mass m and radius R is spinning with angular velocity omega. The power P radiated by it as gravitational waves is given by P = Gc^(–5)m^(x)R^(y)omega^(z), where c and G are speed of light in free space, and the universal gravitational constant , respectively. Then by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
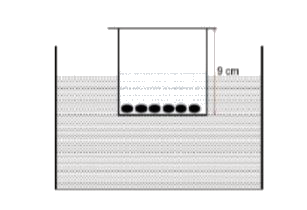
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
 01:42View Solution
01:42View SolutionThe condition for a uniform spherical mass m of a radius r to be a black hole is [G =gravitational constant and g=acceleration due to gravity]
06:28View SolutionIf the energy, E=Gphqcr, where G is the universal gravitational constant, h is the Planck's constant and c is the velocity of light, then the values of p are q and r are, respectively
09:46View SolutionThree point masses each of mass m rotate in a circle of radius r with constant angular velocity ω due to their mutual gravitational attraction. If at any instant, the masses are on the vertices of an equilateral triangle of side a, then the value of ω is
03:55View SolutionA ring of mass m and radius R is being rotated about its axis with constant angular velocity omega in the gravity free space. Find tension in the ring.
03:02View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then :
01:44View SolutionA neutron star with magnetic moment of magnitude m is spinning with angular velocity ω about its magnetic axis. The electromagnetic power P radiated by it is given by μx0myωzcu where μ0 and c are the permeability and speed of light in free space, respectively. Then
02:23View SolutionThe condition for the uniform sphere of mass m of radius r to be a black hole is (G = gravitational constant) :
View SolutionIf the energy, E=Gphqcr, where G is the universal gravitational constant, h is the Planck's constant and c is the velocity of light, then the values of p, q and r are, respectively
03:29View SolutionThe condition for a uniform spherical mass m of radius r to be a black hole is .....
(G = gravitational constant and g = acceleration due to gravity)03:59View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity ω in free space then:
06:25View Solutionm द्रव्यमान तथा r त्रिज्या के एक समरूप गोले को ब्लैक होल में बदलने के लिए आवश्यक शर्त है
(G = गुरुत्वाकर्षण नियतांक तथा g = गुरुत्वीय त्वरण)05:09View SolutionIf the energy, E=Gphqcr, where G is the universal gravitational constant, h is the Planck's constant and c is the velocity of light, then the values of p are q and r are, respectively
02:45View SolutionThree point masses each of mass m rotate in a circle of radius r with constant angular velocity ω due to their mutual gravitational attraction. If at any instant, the masses are on the vertices of an equilateral triangle of side a, then the value of ω is
04:02View SolutionFind the angular momentum of a satellite of mass m moving round the earth of mass m if its orbital radius is r, assuming it to be circular. Express it in terms of G, M, m and r, where G is the universal gravitational constant ?
02:47View Solution

