Three point masses each of mass $m$ rotate in a circle of radius $r$ with constant angular velocity $ω$ due to their mutual gravitational attraction. If at any instant, the masses are on the vertices of an equilateral triangle of side $a$ , then the value of $ω$ is

A

$\sqrt{\frac{G m}{a^{3}}}$

B

$\sqrt{\frac{3 G m}{a^{3}}}$

C

$\sqrt{\frac{G m}{3 a^{3}}}$

D

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

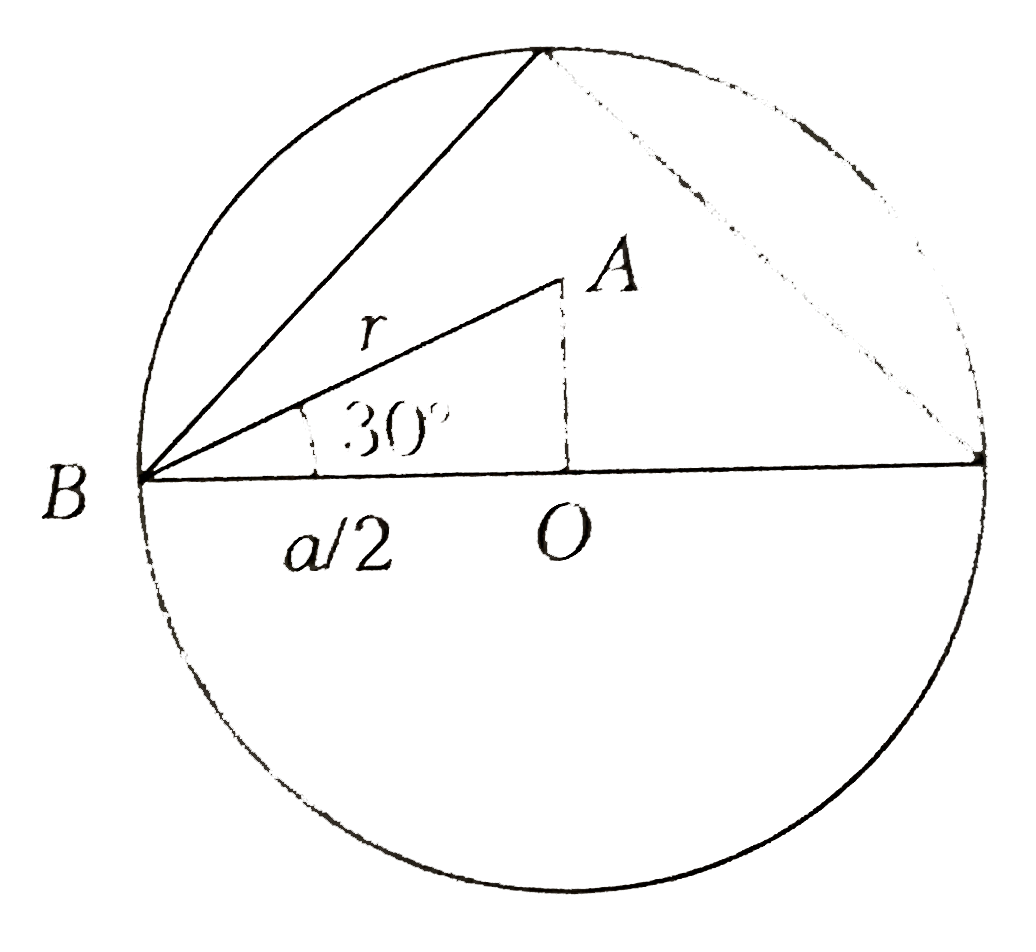
In right angled $Δ A O B, {cos 30}^{\circ} = \frac{O B}{A B}$
$\Rightarrow \frac{\sqrt{3}}{2} = \frac{a}{2 r} \Rightarrow r = \frac{a}{\sqrt{3}}$
$\sqrt{3} (\frac{G m m}{a^{2}}) = m \frac{a}{\sqrt{3}} . ω^{2}$
$∴ ω = \sqrt{\frac{3 G m}{a^{3}}}$

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two particles of equal mass go around a circle of radius R under the action of their mutual gravitational attraction. The speed v of each particle is
A $\frac{1}{2 R} \sqrt{(\frac{1}{G m})}$
B $\sqrt{(\frac{G m}{2 R})}$
C $\frac{1}{2} \sqrt{(\frac{G m}{R})}$
D $\sqrt{(\frac{4 G m}{R})}$
Question 2 - Select One
A stone of mass M tied at the end of a string, is moving in a circular of radius R, with a constant angular velocity $ω$ . The work done on the stone, in any half circle I s
A $π M R^{2} ω^{2}$
B $2 M R^{2} ω^{2}$
C $M R^{2} ω^{2}$
Dzero

DC PANDEY ENGLISH-GRAVITATION-(A) Chapter Exercises

Home
Ask Doubt
Profile