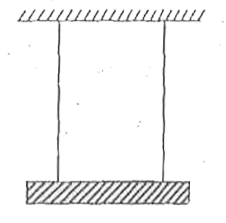
Two wires of equal length and cross-section area suspended as shown in figure. Their Young's modulus are Y1 and Y2 respectively. The equavalent Young's modulus will be

Y1+Y2
Y1+Y22
Y1Y2Y1+y2
√Y1Y2
The correct Answer is:B
Step by step video, text & image solution for Two wires of equal length and cross-section area suspended as shown in figure. Their Young's modulus are Y_(1) and Y_(2) respectively. The equavalent Young's modulus will be by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced
57 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEYCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEYCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEYCHAPTER - RAY OPTICSEXERCISE - Integer type q.
15 Videos
Similar Questions
Two wires of equal length and cross-sectional area are suspended as shown in figure. Their Young's modullare Y1 andY2 respectively. The equivalent Young's modulil will bebr>
View SolutionTwo wires of equal length and cross sectional area suspended as shown in
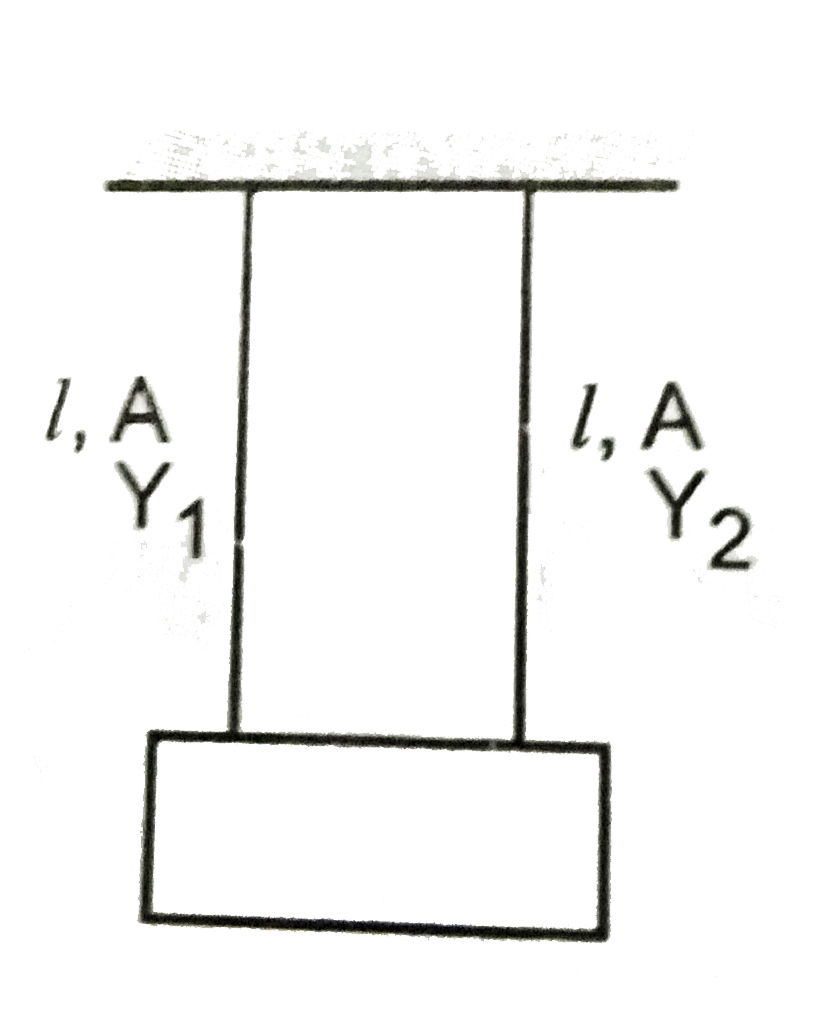
Their Young's modulii are Y1=2×1011 Pa and Y2=0.90×1011 Pa respectively. What will be the equivalent Young's modulus of combination?
View Solution
Knowledge Check
Two wires of equal length and cross-sectional area are suspended as shown in figure. Their Young's modullare Y1 andY2 respectively. The equivalent Young's modulil will bebr>
 AY1+Y2BY1+Y22CY1Y2Y1+Y2D√Y1Y2
AY1+Y2BY1+Y22CY1Y2Y1+Y2D√Y1Y2Three vertical wires, I,II and III are supporting a block of mass M in horizontal positon. The wires are to equal length and cross-sectional area. It is given that Young's modulus of wire II,Y2=Y3 (Yong's modulus of wire III). The wire I and III and attached at extreme ends of the block.
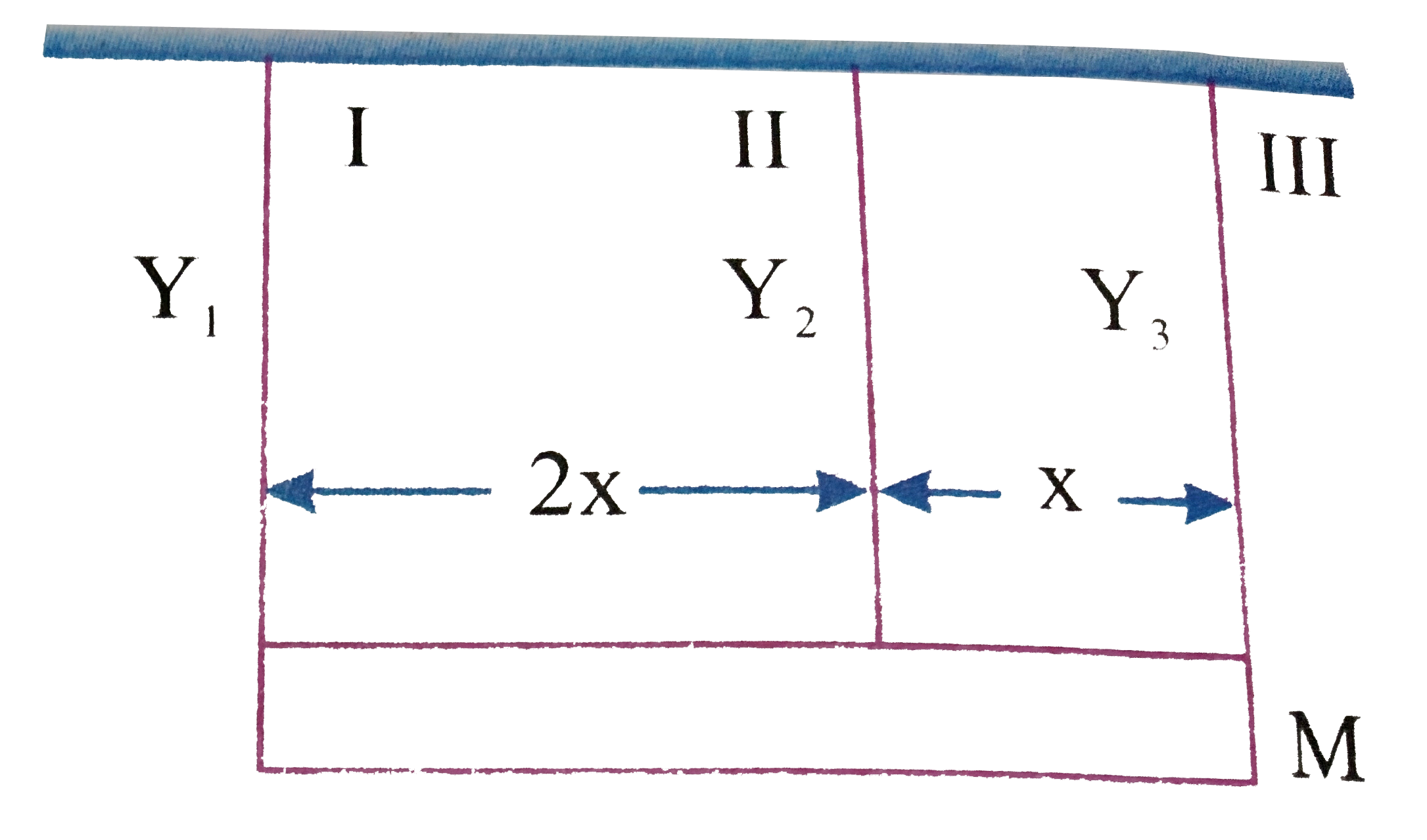 AT1=2T3B2T1=T3CT2=T3DT1=Mg/5
AT1=2T3B2T1=T3CT2=T3DT1=Mg/5The elastic enery stored in a wire of Young's modulus Y is
AY×Strain2VolumeBStress × Strain × VolumeCStress2×Volume2YD12Y× stress × Strain × Volume
A horizontal rod suspended from two wires of same length and cross - section. Their Young's modulus are Y1 and Y2 respectively. The equivalent Young's modulus will be

View SolutionTwo wires have identical lengths and areas of cross-section are suspended by mass M. Their Young's modulus are in the ratio 2:5.
View SolutionA wire having a length L and cross- sectional area A is suspended at one of its ends from a ceiling . Density and young's modulus of material of the wire are ρ and Y, respectively. Its strain energy due to its own weight is ρ2g2AL3αY. Find the value of α
View SolutionTwo wires of equal dimensions and young's modulus Y1 and Y2 are connected end to end. What is the equivalent young's modulus for the combination?
View SolutionA wire of length L and cross-sectional area A is made of material of Young's modulus Y. The work done in stretching the wire by an amount x is
View Solution
