A platform P is moving with a velocity vP over hemispherical shell. A vertical rod AB passing trhough a hole in the platform is moving on the shell and remains vertical. There is sufficient friction between rod and shell to stop the slip. C is the crown of the shell and O is its cenre ∠BOC=θ at any instant. find the velocity of point B in downward motion at that instnat:
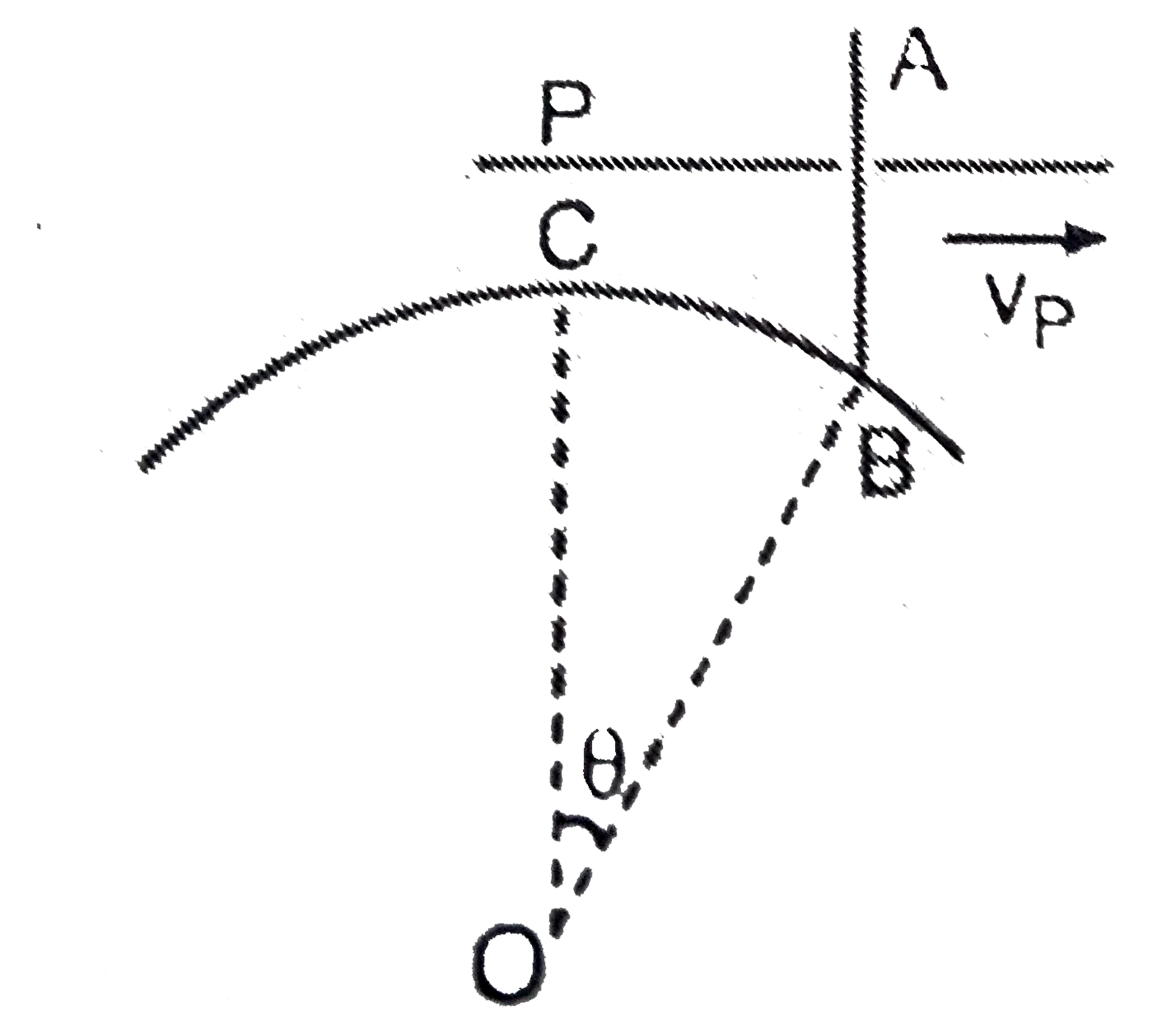
upsinθ
upcosθ
uptanθ
upcotθ
The correct Answer is:c
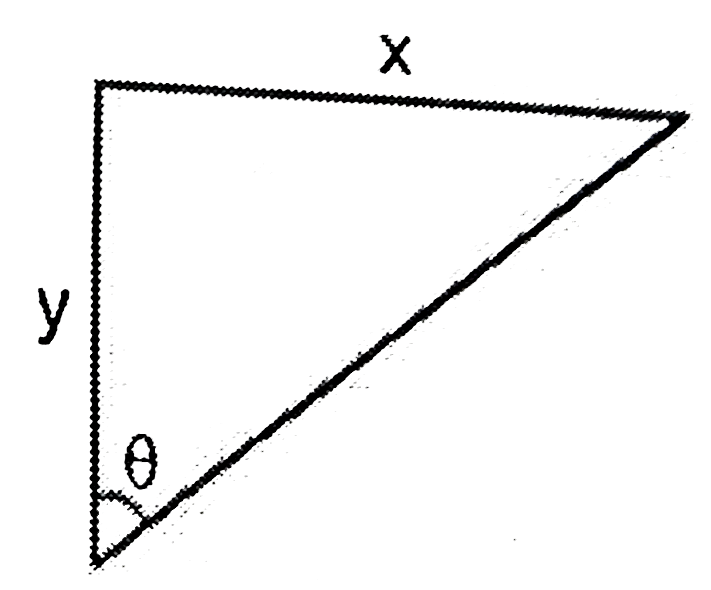
xy=tanθ
x=ytanθ
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the previous problem, the velocity of the shell will be
A30ms−1B30√2ms−1C30√3ms−1Dnone of theseA thin non-conducting hemispherical shell contains a positive charge q on it, which uniformly distributed on the shell. A point P lies on the diameter of shell as show in figure. Then the direction of electric field at the point 'P' is
 A
A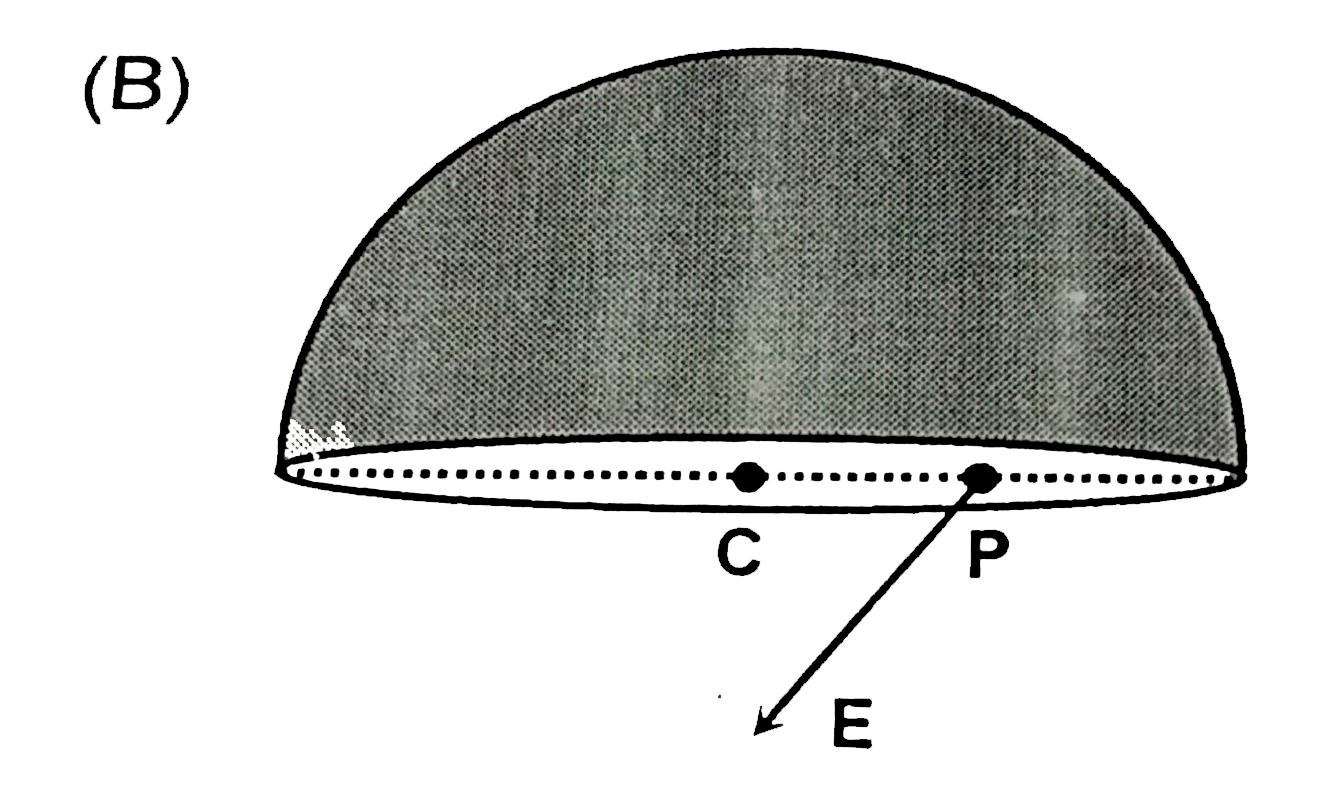 B
B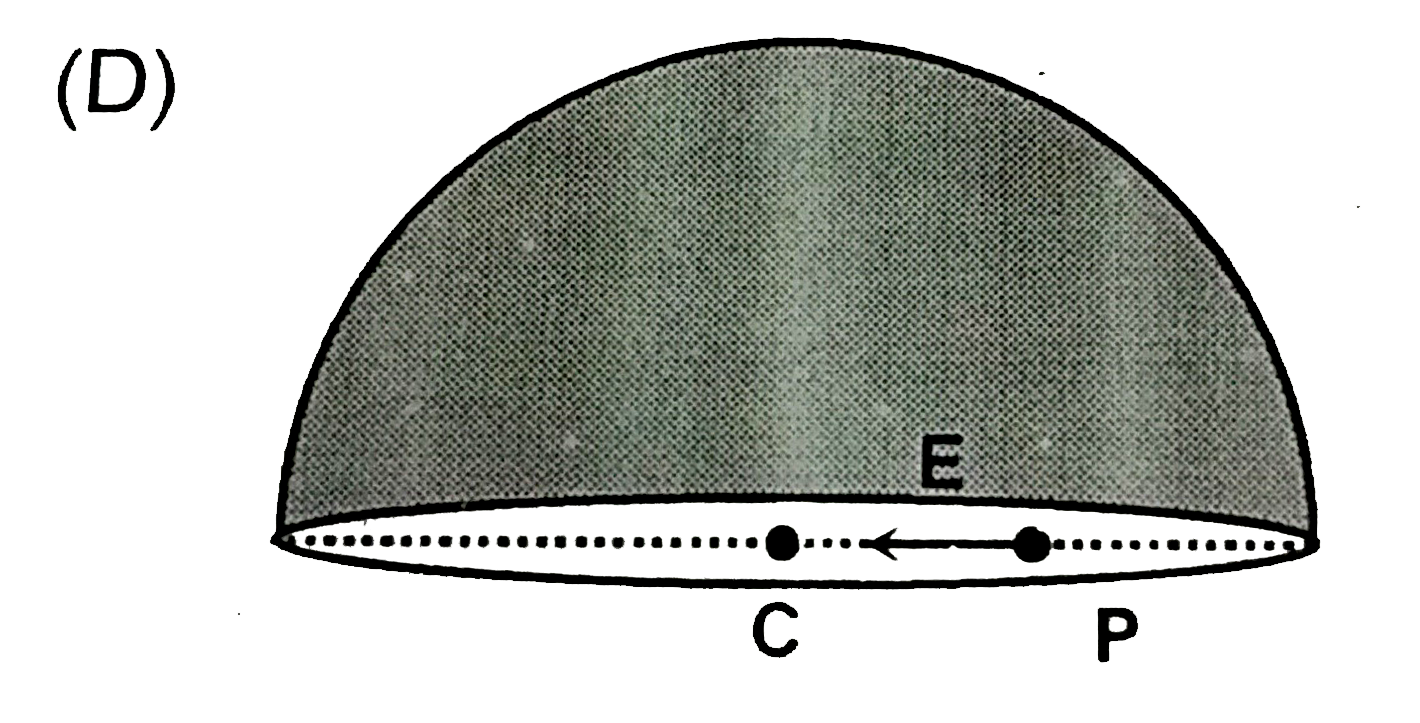 C
C D
D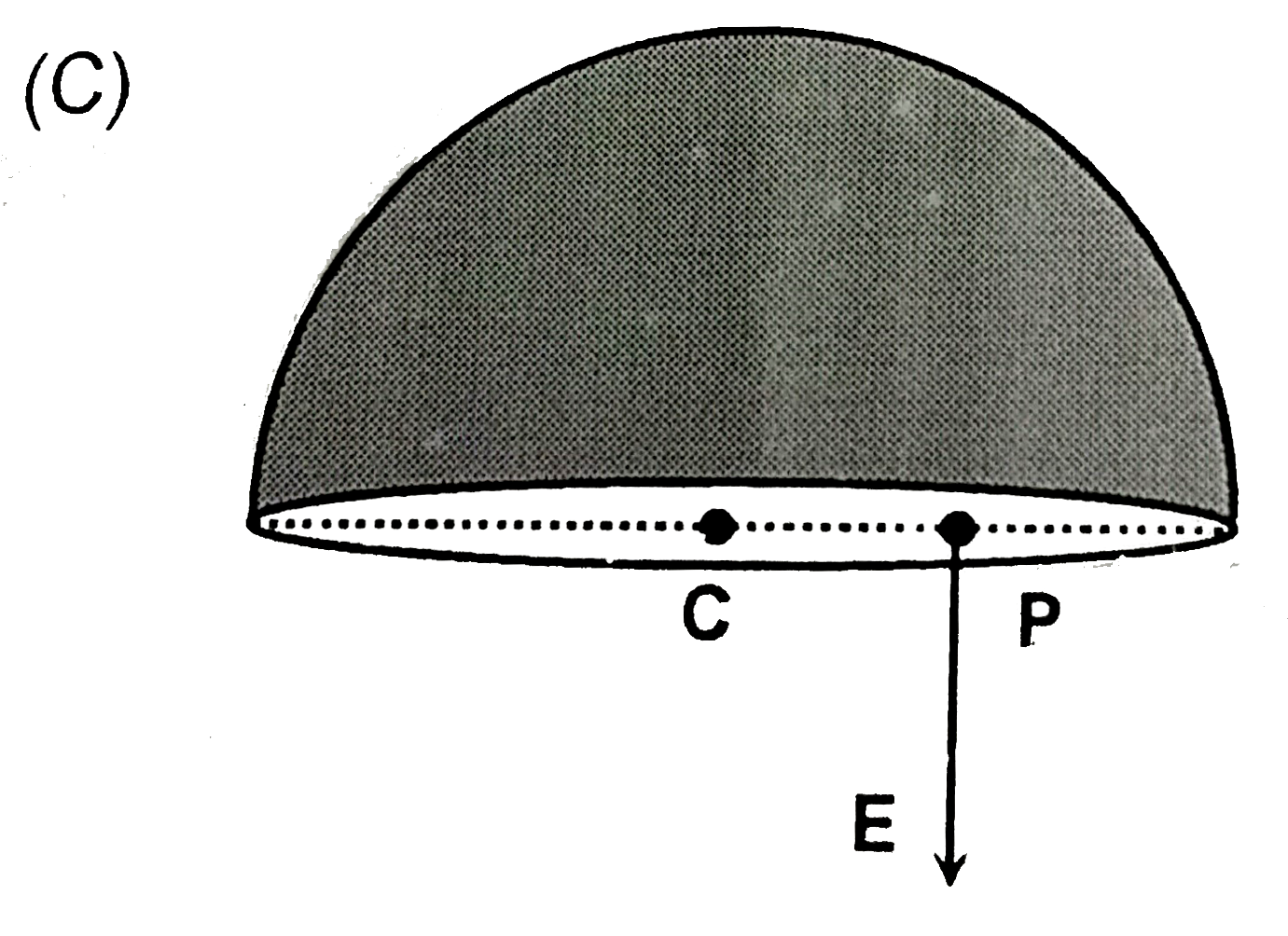
A vertical rod of length l is moved with constant velocity v towards east. The vertical component of earth magnetic field is B and angle of dip is θ. The induced e.m.f. in the rod is
ABlvsinθBBlvtanθCBlvcotθDBlvcosθ
A thin non-conducting hemispherical shell contains a positive charge q on it, which uniformly distributed on the shell. A point P lies on the diameter of shell as show in figure. Then the direction of electric field at the point 'P' is

View SolutionA vertical rod of length l is moved with constant velocity v towards east. The vertical component of earth magnetic field is B and angle of dip is θ. The induced e.m.f. in the rod is
View SolutionMagnetic moment of a rotating ring| Rod and spherical shell
View SolutionA shell of mass m moving with velocity v suddenly breaks into 2 pieces. The part having mass m /4 remains stationary. The velocity of the other shell will be
View SolutionA shell of mass m moving with velocity v suddenly breaks into 2 pieces. The part having mass m /4 remains stationary. The velocity of the other shell will be
View Solution
