For component of a vector A=(3ˆi+4ˆj−5ˆk), match the following table

The correct Answer is:a→q;b→r;c→s;d→s
→A=3ˆi+4ˆj−5ˆk
along vector (2ˆi+ˆj+2ˆk) we have to take projection.
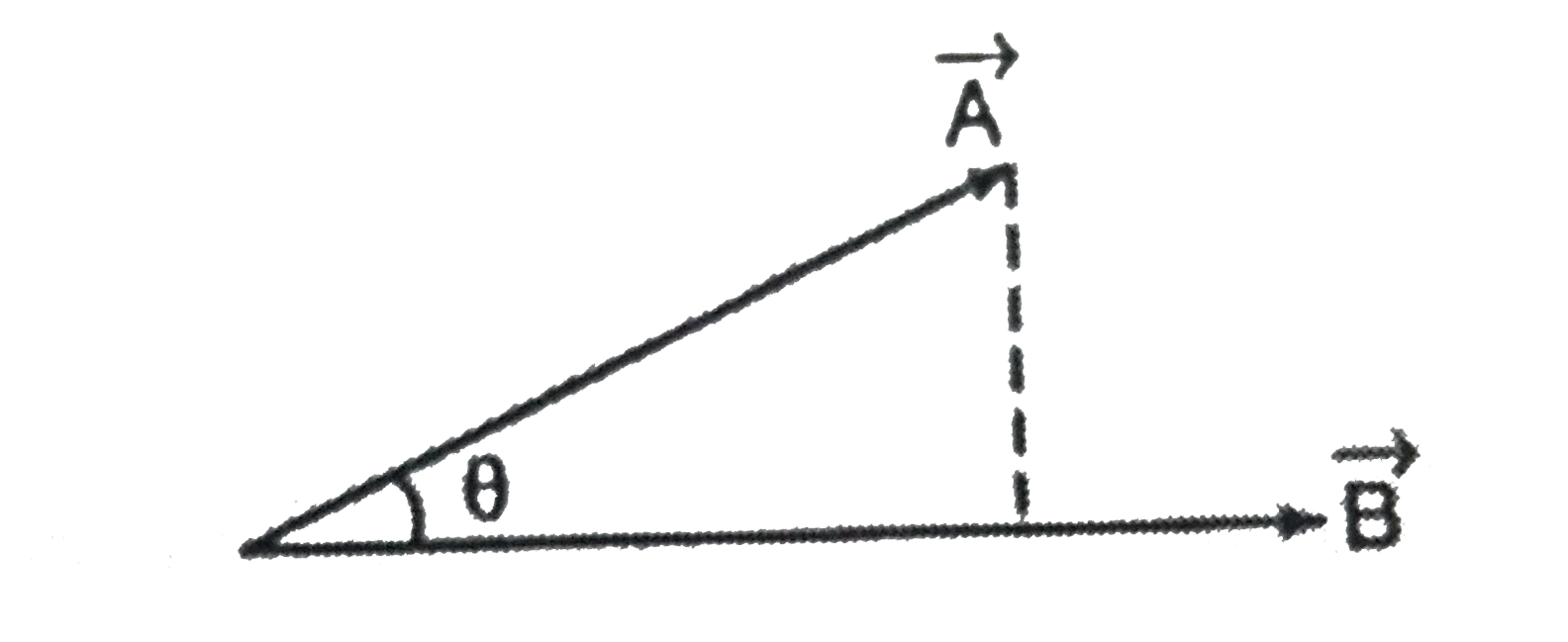
→A.→B=ABcosθ
Acosθ=(→A.→BB)
Along y-axis the component is 4 units.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The vector component of vector
→A=3ˆi+4ˆj+5ˆk along vector →B=ˆi+ˆj+ˆk is :A2ˆi+2ˆj+2ˆkB3ˆi+3ˆj+3ˆkC4ˆi+4ˆj+4ˆkD5ˆi+5ˆj+5ˆk - Question 2 - Select One
The direction cosines of the vector 3ˆi−4ˆj+5ˆk are
A35,−45,15B35√2,−45√2,1√2C3√2,−4√2,1√2D35√2,45√2,1√2. - Question 3 - Select One
The direction cosines of the vector 3ˆi−4ˆj+5ˆk are
A35,−45,15B35√2,−45√2,1√2C3√2,−4√2,1√2D35√2,45√2,1√2.
If the magnitude of the vector product of the vector ˆi+ˆj+ˆk with a unit vector along the sum of vector 2ˆi+4ˆj−5ˆk and λˆi+2ˆj+3ˆk is equal to √2, then find the value of 'λ'
View SolutionFind the (i) Scalar component and (ii)vector component of A=3ˆi+4ˆj+5ˆkonB=ˆi+ˆj+ˆk.
View SolutionThe scalar product of the vector ˆi+ˆj+ˆk with a unit vedctor along the sum of the vectors 2ˆi+4ˆj−5ˆkandλˆi+2ˆj+3ˆk is equal to one. Find the value of λ.
View SolutionFind a unit vector in the diection of the vector.
(i) (3ˆi+4ˆj−5ˆk) , (ii) (3ˆi−2ˆj+6ˆk)
(iii) (ˆi+ˆk) , (iv) (2ˆi+ˆj+2ˆk)View SolutionIf →A=2ˆi+ˆj+ˆkand→B=ˆi+ˆj+ˆk are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:
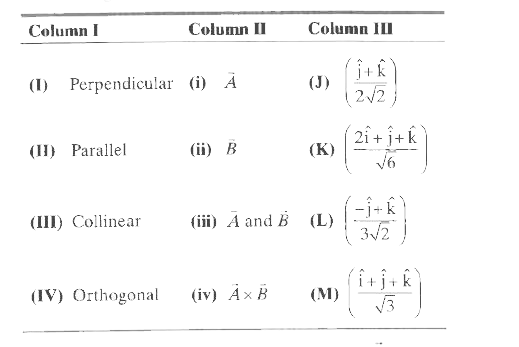
The correct alternative for a collinear vector to →A isView Solution

